Local ring
A commutative ring with a unit that has a unique maximal ideal. If  is a local ring with maximal ideal
is a local ring with maximal ideal  , then the quotient ring
, then the quotient ring  is a field, called the residue field of
is a field, called the residue field of  .
.
Examples of local rings. Any field or valuation ring is local. The ring of formal power series  over a field
over a field  or over any local ring is local. On the other hand, the polynomial ring
or over any local ring is local. On the other hand, the polynomial ring  with
with  is not local. Let
is not local. Let  be a topological space (or a differentiable manifold, an analytic space or an algebraic variety) and let
be a topological space (or a differentiable manifold, an analytic space or an algebraic variety) and let  be a point of
be a point of  . Let
. Let 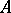 be the ring of germs at
be the ring of germs at  of continuous functions (respectively, differentiable, analytic or regular functions); then
of continuous functions (respectively, differentiable, analytic or regular functions); then 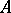 is a local ring whose maximal ideal consists of the germs of functions that vanish at
is a local ring whose maximal ideal consists of the germs of functions that vanish at  .
.
Some general ring-theoretical constructions lead to local rings, the most important of which is localization (cf. Localization in a commutative algebra). Let 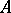 be a commutative ring and let
be a commutative ring and let 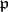 be a prime ideal of
be a prime ideal of  . The ring
. The ring 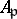 , which consists of fractions of the form
, which consists of fractions of the form 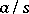 , where
, where  ,
, 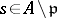 , is local and is called the localization of the ring
, is local and is called the localization of the ring 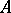 at
at  . The maximal ideal of
. The maximal ideal of 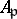 is
is  , and the residue field of
, and the residue field of 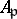 is identified with the field of fractions of the integral quotient ring
is identified with the field of fractions of the integral quotient ring 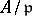 . Other constructions that lead to local rings are Henselization (cf. Hensel ring) or completion of a ring with respect to a maximal ideal. Any quotient ring of a local ring is also local.
. Other constructions that lead to local rings are Henselization (cf. Hensel ring) or completion of a ring with respect to a maximal ideal. Any quotient ring of a local ring is also local.
A property of a ring 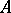 (or an
(or an 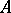 -module
-module 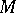 , or an
, or an 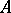 -algebra
-algebra 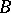 ) is called a local property if its validity for
) is called a local property if its validity for 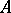 (or
(or 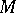 , or
, or 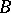 ) is equivalent to its validity for the rings
) is equivalent to its validity for the rings  (respectively, modules
(respectively, modules 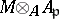 or algebras
or algebras 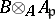 ) for all prime ideals
) for all prime ideals 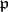 of
of 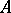 (see Local property).
(see Local property).
The powers 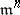 of the maximal ideal
of the maximal ideal 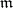 of a local ring
of a local ring 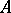 determine a basis of neighbourhoods of zero of the so-called local-ring topology (or
determine a basis of neighbourhoods of zero of the so-called local-ring topology (or  -adic topology). For a Noetherian local ring this topology is separated (Krull's theorem), and any ideal of it is closed.
-adic topology). For a Noetherian local ring this topology is separated (Krull's theorem), and any ideal of it is closed.
From now on only Noetherian local rings are considered (cf. also Noetherian ring). A local ring is called a complete local ring if it is complete with respect to the 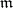 -adic topology; in this case
-adic topology; in this case 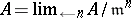 . In a complete local ring the
. In a complete local ring the 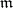 -adic topology is weaker than any other separated topology (Chevalley's theorem). Any complete local ring can be represented as the quotient ring of the ring
-adic topology is weaker than any other separated topology (Chevalley's theorem). Any complete local ring can be represented as the quotient ring of the ring 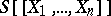 of formal power series, where
of formal power series, where 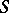 is a field (in the case of equal characteristic) or of a complete discrete valuation ring (in the case of different characteristic). This theorem makes it possible to prove that complete local rings have a number of specific properties that are absent in arbitrary Noetherian local rings (see [5]); for example, a complete local ring is an excellent ring.
is a field (in the case of equal characteristic) or of a complete discrete valuation ring (in the case of different characteristic). This theorem makes it possible to prove that complete local rings have a number of specific properties that are absent in arbitrary Noetherian local rings (see [5]); for example, a complete local ring is an excellent ring.
A finer quantitative investigation of a local ring 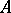 is connected with the application of the concept of the adjoint graded ring
is connected with the application of the concept of the adjoint graded ring 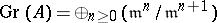 . Let
. Let 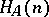 be the dimension of the vector space
be the dimension of the vector space 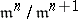 over the residue field
over the residue field 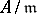 ; as a function of the integer argument
; as a function of the integer argument  it is called the Hilbert–Samuel function (or characteristic function) of the local ring
it is called the Hilbert–Samuel function (or characteristic function) of the local ring 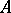 . For large
. For large  this function coincides with a certain polynomial
this function coincides with a certain polynomial 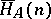 in
in  , which is called the Hilbert–Samuel polynomial of the local ring
, which is called the Hilbert–Samuel polynomial of the local ring 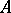 (see also Hilbert polynomial). This fact can be expressed in terms of a Poincaré series: The formal series
(see also Hilbert polynomial). This fact can be expressed in terms of a Poincaré series: The formal series
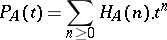 |
is a rational function of the form 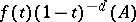 , where
, where 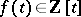 is a polynomial and
is a polynomial and 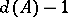 is the degree of
is the degree of 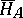 . The integer
. The integer 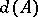 is the (Krull) dimension
is the (Krull) dimension 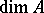 of the ring
of the ring  and is one of the most important invariants of a ring. Moreover,
and is one of the most important invariants of a ring. Moreover, 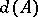 is equal to the least number of elements
is equal to the least number of elements 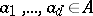 for which the quotient ring
for which the quotient ring 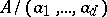 is Artinian (cf. Artinian ring). If these elements can be chosen in such a way that they generate the maximal ideal
is Artinian (cf. Artinian ring). If these elements can be chosen in such a way that they generate the maximal ideal  , then
, then 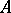 is called a regular local ring. The regularity of
is called a regular local ring. The regularity of 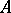 is equivalent to the fact that
is equivalent to the fact that 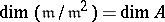 . For a
. For a 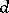 -dimensional regular ring
-dimensional regular ring  ,
,
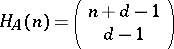 |
and 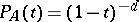 . Geometrically, regularity means that the corresponding point of the (analytic or algebraic) variety is non-singular.
. Geometrically, regularity means that the corresponding point of the (analytic or algebraic) variety is non-singular.
Besides the characteristic function 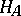 and the dimension and multiplicity connected with it, a local ring has various invariants of a homological kind. The main one of these is the depth
and the dimension and multiplicity connected with it, a local ring has various invariants of a homological kind. The main one of these is the depth 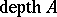 (see Depth of a module); the condition
(see Depth of a module); the condition 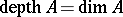 distinguishes among local rings the so-called Cohen–Macaulay rings (cf. Cohen–Macaulay ring). It is not known (1989) whether there is a module
distinguishes among local rings the so-called Cohen–Macaulay rings (cf. Cohen–Macaulay ring). It is not known (1989) whether there is a module 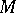 with
with 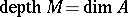 for an arbitrary or a complete local ring
for an arbitrary or a complete local ring 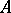 . Other homological invariants are the so-called Betti numbers
. Other homological invariants are the so-called Betti numbers 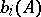 of a local ring
of a local ring 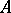 , that is, the dimensions of the
, that is, the dimensions of the  -spaces
-spaces 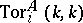 , where
, where 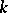 is the residue field of
is the residue field of 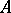 . The question of the rationality of the Poincaré series
. The question of the rationality of the Poincaré series 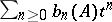 is open, although for many classes of rings an affirmative answer is known. There are also invariants of an algebraic-geometrical nature; for their definition one uses resolution of the singularity corresponding to the local ring.
is open, although for many classes of rings an affirmative answer is known. There are also invariants of an algebraic-geometrical nature; for their definition one uses resolution of the singularity corresponding to the local ring.
A similar theory has been constructed for semi-local rings; that is, rings that have finitely many maximal ideals. The role of a maximal ideal for them is played by the Jacobson radical.
References
| [1] | W. Krull, "Dimensionstheorie in Stellenringen" J. Reine Angew. Math. , 179 (1939) pp. 204–226 |
| [2] | C. Chevalley, "On the theory of local rings" Ann. of Math. (2) , 44 (1943) pp. 690–708 |
| [3] | I.S. Cohen, "On the structure and ideal theory of complete local rings" Trans. Amer. Math. Soc. , 59 (1946) pp. 54–106 |
| [4] | P. Samuel, "Algèbre locale" , Gauthier-Villars (1953) |
| [5] | M. Nagata, "Local rings" , Interscience (1962) |
| [6] | O. Zariski, P. Samuel, "Commutative algebra" , 2 , Springer (1975) |
| [7] | J.-P. Serre, "Algèbre locale. Multiplicités" , Lect. notes in math. , 11 , Springer (1965) |
| [8] | N. Bourbaki, "Elements of mathematics. Commutative algebra" , Addison-Wesley (1972) (Translated from French) |
| [9] | M.F. Atiyah, I.G. Macdonald, "Introduction to commutative algebra" , Addison-Wesley (1969) |
Comments
For the notion of Krull dimension see Dimension of an associative ring.
A counter-example to the question of the rationality of the Poincaré series was given by D. Anick [a1].
References
| [a1] | D. Anick, "Construction d'espaces de lacets et d'anneaux locaux à séries de Poincaré–Betti non rationelles" C.R. Acad. Soc. Paris , 290 (1980) pp. 1729–1732 (English abstract) |
Local ring. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Local_ring&oldid=12768