Gel'fond-Baker method
for Diophantine equations
One of the most efficient methods for obtaining explicit upper bounds on the size of integer solutions of some broad classes of Diophantine equations arises from the theory of transcendental numbers, from the solution by A.O. Gel'fond of Hilbert's seventh problem and from subsequent work of A. Baker. The Gel'fond–Baker method also incorporates ideas due to C.L. Siegel, K. Mahler and S. Lang. From this method, explicit upper bounds have been derived for:
1) integer points on curves of genus  and
and 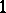 (effective versions of Siegel's theorem, due to Baker, J. Coates and W.M. Schmidt); [a2], Chapt. IV, Sect. 12;
(effective versions of Siegel's theorem, due to Baker, J. Coates and W.M. Schmidt); [a2], Chapt. IV, Sect. 12;
2) Thue equations 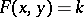 , where
, where 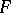 is a homogeneous polynomial with algebraic coefficients,
is a homogeneous polynomial with algebraic coefficients, 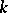 is a fixed non-zero algebraic number, and the polynomial
is a fixed non-zero algebraic number, and the polynomial 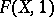 has at least three distinct roots; [a1], Chapt. 5; [a2], Chapt. 3; [a3], Chapt. 4–5;
has at least three distinct roots; [a1], Chapt. 5; [a2], Chapt. 3; [a3], Chapt. 4–5;
3) hyper-elliptic and super-elliptic equations, namely 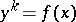 where
where 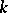 is a fixed integer and
is a fixed integer and 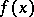 is a polynomial with algebraic coefficients, which define curves of positive genus. This is the case when
is a polynomial with algebraic coefficients, which define curves of positive genus. This is the case when 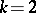 and
and 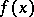 has at least three distinct roots or when
has at least three distinct roots or when  and
and  has at least two distinct roots; [a2], Chapt. 4; [a3], Chapt. 6. The method also applies to some classes of norm-form equations in more than two variables (by the work of K. Győry).
has at least two distinct roots; [a2], Chapt. 4; [a3], Chapt. 6. The method also applies to some classes of norm-form equations in more than two variables (by the work of K. Győry).
The bounds obtained from this method are typically extremely large, and some further results on Diophantine approximations are required to be able to completely determine all the solutions. Upper estimates are obtained for the height of the solutions, either in the ring of integers of a fixed algebraic number field 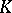 , or, more generally, in the ring of
, or, more generally, in the ring of 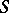 -integers, where
-integers, where 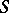 is a fixed finite set of prime ideals of
is a fixed finite set of prime ideals of 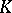 (cf. also Ideal; Prime ideal). This means that a denominator for
(cf. also Ideal; Prime ideal). This means that a denominator for  and
and 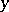 is allowed, but is required to be the product of primes from a fixed finite set
is allowed, but is required to be the product of primes from a fixed finite set  . This generalizes ordinary integers and units, which arise from taking for
. This generalizes ordinary integers and units, which arise from taking for 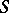 the empty set. For instance, for the field of rational numbers, given a finite set
the empty set. For instance, for the field of rational numbers, given a finite set  of prime numbers, the
of prime numbers, the 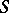 -integers are the rational numbers
-integers are the rational numbers  such that all prime divisors of the denominator
such that all prime divisors of the denominator 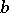 belong to
belong to 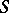 , while the
, while the 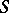 -units are the rational numbers of the form
-units are the rational numbers of the form 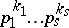 with
with 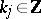 (
(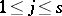 ).
).
Furthermore, the method can be extended in some cases to allow the exponents on  and
and  to vary. The most celebrated example is Tijdeman's theorem on the Catalan equation [a1], Chapt. 12, [a3], Chapt. 7, Sect. 3: There are only finitely many tuples
to vary. The most celebrated example is Tijdeman's theorem on the Catalan equation [a1], Chapt. 12, [a3], Chapt. 7, Sect. 3: There are only finitely many tuples 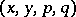 of integers, all of them
of integers, all of them 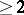 , satisfying
, satisfying  .
.
According to Pillai's conjecture [a1], p. 201, [a2], p. 207, for each  the same should hold for the equation
the same should hold for the equation 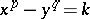 .
.
To demonstrate Baker's method, consider the Thue equation 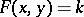 , where
, where 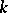 is a fixed non-zero algebraic number, while
is a fixed non-zero algebraic number, while 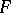 is a homogeneous polynomial with algebraic coefficients such that
is a homogeneous polynomial with algebraic coefficients such that  has at least three distinct roots,
has at least three distinct roots,  ,
, 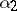 ,
, 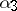 . The unknowns
. The unknowns  and
and  are algebraic integers in a number field
are algebraic integers in a number field 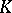 , which is assumed to contain not only the number
, which is assumed to contain not only the number 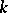 , but also the coefficients of
, but also the coefficients of 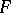 as well as the roots of
as well as the roots of 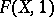 . Then one may write, for
. Then one may write, for  ,
, 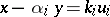 , where
, where 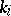 belongs to a fixed finite subset of
belongs to a fixed finite subset of 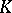 (independent of the solution
(independent of the solution 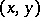 ), while
), while 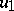 ,
, 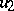 ,
, 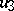 are (unknown) units in
are (unknown) units in 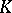 . Eliminating
. Eliminating  and
and 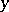 from these three equations yields the relation
from these three equations yields the relation
 |
Therefore, a key step in the Gel'fond–Baker method is the following result, which deals with the 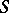 -unit equation: If
-unit equation: If 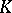 is a number field and
is a number field and 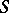 is a finite set of prime ideals of
is a finite set of prime ideals of 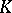 , the equation
, the equation 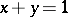 has only finitely many solutions in
has only finitely many solutions in 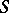 -units
-units 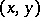 of
of 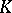 . Moreover, explicit bounds for the size of the solutions can be given.
. Moreover, explicit bounds for the size of the solutions can be given.
The proof of this result relies on an estimate from transcendental number theory. Write  and
and  using a basis
using a basis  of the group of
of the group of 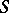 -units modulo torsion. Then a "large" solution
-units modulo torsion. Then a "large" solution  to the Diophantine equation
to the Diophantine equation  gives rise to a "small" value for a number of the form
gives rise to a "small" value for a number of the form 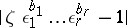 , where
, where 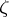 is a root of unity and
is a root of unity and  are rational integers. The so-called "theory of linear forms in logarithms" (cf. Linear form in logarithms) provides a lower bound for such numbers, which is sharp in terms of
are rational integers. The so-called "theory of linear forms in logarithms" (cf. Linear form in logarithms) provides a lower bound for such numbers, which is sharp in terms of 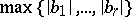 . This lower bound, together with the link between large solutions and small values, then provides an upper bound for the size of solutions.
. This lower bound, together with the link between large solutions and small values, then provides an upper bound for the size of solutions.
The Schmidt subspace theorem and its  -adic variants by H.P. Schlickewei [a2], Chapt.5, Sect. 1, imply more generally that for each
-adic variants by H.P. Schlickewei [a2], Chapt.5, Sect. 1, imply more generally that for each 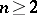 , the Diophantine equation
, the Diophantine equation 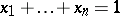 has only finitely many solutions in
has only finitely many solutions in  -units of
-units of 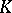 (one considers only solutions where no subsum on the left-hand side vanishes). But for
(one considers only solutions where no subsum on the left-hand side vanishes). But for  the result is not effective: upper bounds for the number of solutions can be given, but no bound for the size of the solutions is known in general.
the result is not effective: upper bounds for the number of solutions can be given, but no bound for the size of the solutions is known in general.
References
| [a1] | T.N. Shorey, R. Tijdeman, "Exponential diophantine equations" , Tracts in Math. , 87 , Cambridge Univ. Press (1986) |
| [a2] | W.M. Schmidt, "Diophantine approximations and Diophantine equations" , Lecture Notes in Mathematics , 1467 , Springer (1991) |
| [a3] | V.G. Sprindžuk, "Classical diophantine equations" , Lecture Notes in Mathematics , 1559 , Springer (1993) (In Russian) |
Gel'fond-Baker method. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gel%27fond-Baker_method&oldid=11349