Continuous functional
A continuous operator (continuous mapping) mapping a topological space 
, which as a rule is also a vector space, into 
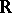or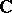 
. Therefore, the definition of, and criteria for, continuity of an arbitrary operator continue to hold for functionals. For example,
1) for a functional 
, where 
is a subset of a topological space 
, to be continuous at a point 
there must for any 
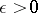be a neighbourhood 
of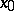 
such that 
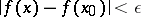for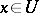 
(definition of continuity of functionals);
2) a functional that is continuous on a compact set of a separable topological vector space is bounded on this set and attains its least upper and greatest lower bounds (Weierstrass' theorem);
3) since every non-zero linear functional maps a Banach space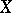 
onto the whole of 
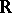(or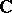 
), it induces an open mapping, that is, the image of any open set 
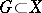is an open set in 
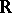(or 
).
Comments
References
| [a1] | A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980) |
Continuous functional. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Continuous_functional&oldid=11921