Class

A term used in mathematics mainly as a synonym for the term "set" for denoting arbitrary collections of objects possessing some definite property of indication (for example, in algebra, equivalence classes with respect to a given equivalence relation). Sometimes the term class is preferred for collections whose elements are sets (for example, in recursion theory: denumerable classes). In some cases, influenced by axiomatic set theory (see 2)), the term "class" is used to emphasize the fact that the given collection is a proper class rather than a set in the narrow sense (for example, in algebra, the primitive classes of universal algebras, also called varieties). The set-theoretic operations on classes are defined in the same way as for sets.

A class in axiomatic set theory (more precisely, in the Gödel–Bernays axiomatic system) is one of the forms of primitive objects considered in these systems. The difference between sets and classes consists here in the fact that only sets, but not (proper) classes, are allowed to be elements of classes. The idea of introducing into set theory classes in the above sense is due to J. von Neumann and is based on his observation that the well-known contradictions in Cantor's set theory arise not as a result of allowing the formation of very large sets, but rather because such sets are allowed to be members of other sets. Apart from this restriction, all usual set-theoretic operations are allowed in the above-mentioned systems for classes, the result being a class and not a set. Furthermore, for each admissible (in some sense) predicate defined on sets, there exists a class consisting precisely of those sets that satisfy the predicate. It has been proved that the consistency of each of the systems of Gödel–Bernays and Zermelo–Fraenkel follows from the consistency of the other (which confirms the standpoint of von Neumann). See also Axiomatic set theory.

References

[1]	P.J. Cohen, "Set theory and the continuum hypothesis" , Benjamin (1966)
[2]	A.A. Fraenkel, Y. Bar-Hillel, "Foundations of set theory" , North-Holland (1958)

V.A. Dushskii

The class of a Riemannian space $ V ^ {l} $ is the number $ p $ such that $ V ^ {l} $ can be locally isometrically imbedded in the $ ( l + p ) $- dimensional Euclidean space $ E ^ {l+} p $ but cannot be imbedded in a Euclidean space of lower dimension. It is required that the imbedding is sufficiently regular (since the Riemannian space $ V ^ {l} $ admits a local isometric imbedding as a $ C ^ {1} $- smooth hypersurface in $ E ^ {l+} 1 $( Nash's theorem); the class of an analytic Riemannian space $ V ^ {l} $ does not exceed $ l ( l - 1 ) / 2 $( the Janet–Cartan theorem). The class of a Riemannian space of differentiability class $ C ^ \alpha $( $ \alpha > 2 $) also does not exceed $ l ( l - 1 ) / 2 $, cf. [10].

The class of a Riemannian space is zero if and only if the curvature tensor of the manifold $ V ^ {l} $ is identically zero. Metrics of constant curvature have class 1 and can be realized as hyperspheres in a Euclidean space. The class of an $ l $- dimensional space of constant negative curvature is $ l - 1 $( Cartan's theorem). The class of a Riemannian manifold $ V ^ {l} $ of strictly negative two-dimensional sectional curvature is at least $ l - 1 $( see [3]). If a Riemannian manifold $ V ^ {l} $ has negative $ k $- dimensional sectional curvature, where $ k $ is even, then its class $ p \geq ( l - 1 ) / ( k - 1 ) $. Algebraic criteria have been found

enabling one to determine whether the class of a given manifold is equal to 1; these are based on the fact that for metrics of class 1, under certain additional conditions, the Peterson–Codazzi equations are consequences of the Gauss equations.

If a Riemannian manifold $ V ^ {l} $ is a metric product of Riemannian manifolds $ V ^ {l _ {i} } $:

$$ V ^ {l} = V ^ {l _ {1} } \times \dots \times V ^ {l _ {k} } ,\ \ l _ {1} + \dots + l _ {k} = l , $$

where the $ V ^ {l _ {i} } $ are spaces of class 1, then $ V $ is of class $ p = k $( see [5]). If the $ V ^ {l _ {i} } $ have constant negative sectional curvature, then the class of their metric product is $ l - k $( see ([5]).

The class of two-dimensional Riemannian manifolds with curvature of constant sign is equal to 1. The question remains open (1978) for a metric of alternating curvature. An example has been constructed [6] of a two-dimensional Riemannian manifold of differentiability class $ C ^ {2,1} $ that does not have a locally isometric immersion into $ E ^ {3} $ of differentiability class $ C ^ {2} $. However, any compact part of a complete metric on the plane can be isometrically immersed into $ E ^ {4} $( where the surface is of differentiability class $ C ^ {2 , \alpha } $ if the metric has regularity $ C ^ {3 , \alpha } $), that is, the class does not exceed 2 [7].

The notion of a class has been introduced for pseudo-Riemannian spaces as well. Let $ V ^ {n} ( p , q ) $ be a pseudo-Riemannian manifold the metric tensor of which has $ p $ positive and $ q $ negative eigen values, $ p + q = n $, and let $ E ^ {n} ( p , q ) $ be the pseudo-Euclidean space with metric

$$ d s ^ {2} = d x _ {1} ^ {2} + \dots + d x _ {p} ^ {2} - d x _ {p+} 1 ^ {2} - \dots - d x _ {n} ^ {2} . $$

Let $ k _ {0} $ be the least non-negative integer such that $ V ^ {n} ( p , q ) $ has an immersion into $ E ^ {n + k _ {0} } ( p , q + k _ {0} ) $. Then for each $ k $ in $ 0 \leq k \leq k _ {0} $ the $ k $- th class of the immersion of $ V ^ {n} ( p , q ) $ is defined to be the least number $ N _ {k} $ for which $ V ^ {n} ( p , q ) $ has an immersion into $ E ^ {n + N _ {k} } ( p + a _ {k} , q + k ) $, where $ a _ {k} = N _ {k} - k $. The immersion class of $ V ^ {n} ( p , q ) $ is defined as $ \min _ {0 \leq k \leq k _ {0} } N _ {k} $.

Any pseudo-Riemannian manifold $ V ^ {n} ( p , q ) $ with an analytic metric has an analytic and isometric immersion into $ E ^ {m} ( r , s ) $, where $ m = n ( n + 1 ) / 2 $ and $ r , s $ are any given integers such that $ r \geq p $, $ s \geq q $, that is, $ N _ {k} \leq n ( n + 1 ) / 2 $ for all $ k $[8]. If the Ricci tensor for $ V ^ {n} ( p , q ) $ is equal to zero, then $ N _ {k} \neq 1 $.

If $ V ^ {n} ( p , q ) $ has constant curvature, then its class is equal to 1, that is, there exists a space $ E ^ {n+} 1 ( r , s ) $ with $ r \geq p $, $ s \geq q $ such that $ V ^ {n} ( p , q ) $ is locally isometric to a part of the hypersphere in $ E ^ {n+} 1 ( r , s ) $. For spaces of constant negative curvature $ N _ {0} = n - 1 $, while $ N = 1 $( see [9]).

References

[1]	L.P. Eisenhart, "Riemannian geometry" , Princeton Univ. Press (1949)
[2]	J.D. Moore, "Isometric immersions of space forms in space forms" Pacific J. Math. , 40 (1972) pp. 157–166
[3]	A.A. Borisenko, "The class of Riemannian spaces of strictly negative curvature" Ukrain. Geom. Sb. , 13 (1973) pp. 15–18 (In Russian)
[4a]	N.A. Rozenson, "On Riemannian spaces of class I. I" Izv. Akad. Nauk SSSR Ser. Mat. , 4 (1940) pp. 181–192 (In Russian) (French summary)
[4b]	N.A. Rozenson, "On Riemannian spaces of class I. II" Izv. Akad. Nauk SSSR Ser. Mat. , 5 (1941) pp. 325–352 (In Russian) (French summary)
[4c]	N.A. Rozenson, "On Riemannian spaces of class I. III" Izv. Akad. Nauk SSSR Ser. Mat. , 7 (1943) pp. 253–284 (In Russian) (French summary)
[5]	J.D. Moore, "Isometric immersions of Riemannian products" J. Differential Geom. , 5 : 1–2 (1971) pp. 159–168
[6]	A.V. Pogorelov, "An example of a two-dimensional Riemannian metric that does not admit a local realization in 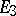" Dokl. Akad. Nauk SSSR , 198 : 1 (1971) pp. 42–43 (In Russian)
[7]	E.G. Poznyak, "Isometric imbedding of two-dimensional Riemannian metrics in Euclidean space" Uspekhi Mat. Nauk , 28 : 4 (172) (1973) pp. 47–76 (In Russian)
[8]	A. Friedman, "Isometric embedding of Riemannian manifolds into Euclidean space" Rev. Modern Physics , 37 (1965) pp. 201–203
[9]	A.A. Borisenko, "Isometric immersion of pseudo-Riemannian spaces of constant curvature" Ukrain. Geom. Sb. , 19 (1976) pp. 11–18 (In Russian)
[10]	H. Jacobowitz, "Extending isometric embeddings" J. Differential Geom. , 9 : 2 (1974) pp. 291–307

A.A. Borisenko

Comments

Good surveys are [a1] and the well-known book of S. Kobayashi and K. Nomizu [a2]. Most of the results mentioned can be found in the latter.

In Western literature the Peterson–Codazzi equations are usually called the Mainardi–Codazzi equations.

References

[a1]	M. Spivak, "A comprehensive introduction to differential geometry" , 5 , Publish or Perish (1976) pp. 1–5
[a2]	S. Kobayashi, K. Nomizu, "Foundations of differential geometry" , 1–2 , Interscience (1963–1969)