Riemannian space, generalized
A space with an internal metric subject to certain restrictions on the curvature. Spaces of "bounded curvature from above" and others belong to this class (see [3]). Generalized Riemannian spaces differ from Riemannian spaces (cf. Riemannian space) not only by greater generality but also by the fact that they are defined and studied on the basis of their metric alone, without coordinates. Under a certain combination of conditions concerning the curvature and the behaviour of shortests curves (i.e. curves whose lengths are equal to the distances between the end points), a generalized Riemannian space turns out to be Riemannian, which gives a purely metric definition of a Riemannian space.
Definitions of generalized Riemannian spaces are based on the classical relation between the curvature and the excess of a geodesic triangle (excess  sum of the angles minus
sum of the angles minus 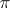 ). These concepts are carried over to a space with an internal metric, such that each point of it has a neighbourhood in which any two points can be connected by a shortest curve. This condition is assumed hereafter without further stipulation. A triangle
). These concepts are carried over to a space with an internal metric, such that each point of it has a neighbourhood in which any two points can be connected by a shortest curve. This condition is assumed hereafter without further stipulation. A triangle  is triplet of shortests curves
is triplet of shortests curves 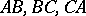 — the sides of the triangle — connecting in pairs three different points
— the sides of the triangle — connecting in pairs three different points  — the vertices of the triangle. The angle between curves can be defined in any metric space: Let
— the vertices of the triangle. The angle between curves can be defined in any metric space: Let 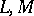 be curves starting at the same point
be curves starting at the same point 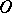 in a space with metric
in a space with metric 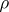 . One chooses points
. One chooses points 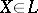 ,
, 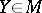
 and constructs the Euclidean triangle with sides
and constructs the Euclidean triangle with sides 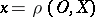 ,
, 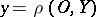 ,
, 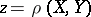 and angle
and angle 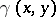 opposite to the side
opposite to the side  . One defines the upper angle between
. One defines the upper angle between  and
and  as:
as:
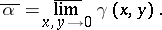 | (1) |
The upper angles of the triangle are the upper angles 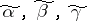 between its sides at the vertices
between its sides at the vertices 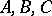 and the excess of the triangle is
and the excess of the triangle is 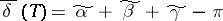 .
.
A generalized Riemannian space of bounded curvature (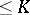 and
and 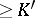 ) is defined by the following condition:
) is defined by the following condition:
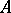 ) for any sequence of triangles
) for any sequence of triangles  contracting to a point,
contracting to a point,
 | (2) |
where 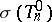 is the area of the Euclidean triangle with the same sides as
is the area of the Euclidean triangle with the same sides as 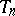 (if
(if  , then
, then  ). Such a space turns out to be Riemannian under two natural additional conditions:
). Such a space turns out to be Riemannian under two natural additional conditions:
1) local compactness of the space (in a space with an internal metric this already ensures the condition of local existence of shortests);
2) local extendibility of shortests, i.e. each point has a neighbourhood 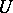 such that any shortest
such that any shortest 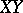 , where
, where 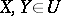 , can be extended beyond its end points. Under all these conditions the space is Riemannian (see [4]); moreover, in a neighbourhood of each point one can introduce coordinates
, can be extended beyond its end points. Under all these conditions the space is Riemannian (see [4]); moreover, in a neighbourhood of each point one can introduce coordinates 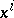 so that the metric will be given by a line element
so that the metric will be given by a line element 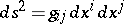 with coefficients
with coefficients 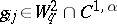 ,
, 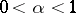 . Thus, a parallel displacement is given (with continuous
. Thus, a parallel displacement is given (with continuous 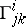 ) and, almost everywhere, a curvature tensor (see [9]).
) and, almost everywhere, a curvature tensor (see [9]).
Moreover, it has been proved [9] that the coordinates 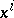 can be taken harmonic, i.e. satisfying the equalities
can be taken harmonic, i.e. satisfying the equalities 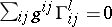 . Harmonic coordinate systems form an atlas of class
. Harmonic coordinate systems form an atlas of class  for any
for any 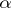 ,
,  .
.
A generalized Riemannian space of bounded curvature with  and satisfying conditions 1) and 2) is a Riemannian space of constant Riemannian curvature
and satisfying conditions 1) and 2) is a Riemannian space of constant Riemannian curvature  (see [3]).
(see [3]).
Any Riemannian space of Riemannian curvature contained in between 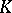 and
and 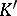 (
(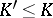 ) is a generalized Riemannian space of curvature
) is a generalized Riemannian space of curvature 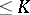 and
and  and satisfies conditions 1) and 2).
and satisfies conditions 1) and 2).
A "space of curvature ≤ K" is defined by the left inequality in 2), i.e. by the condition:
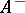 ) for any sequence of triangles
) for any sequence of triangles 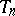 contracting to a point,
contracting to a point,
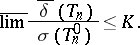 | (3) |
Another, equivalent, definition and a starting point for the study of generalized Riemannian spaces are based on the comparison between an arbitrary triangle 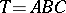 and a triangle
and a triangle 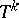 with sides of the same lengths in a space of constant curvature
with sides of the same lengths in a space of constant curvature 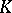 . Let
. Let 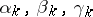 be the angles of such a triangle; the relative upper excess of the triangle
be the angles of such a triangle; the relative upper excess of the triangle 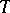 is defined as
is defined as  . Condition
. Condition 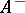 ) in the definition of a space of curvature
) in the definition of a space of curvature 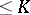 can be replaced by the following condition:
can be replaced by the following condition:
 ) any point has a neighbourhood
) any point has a neighbourhood 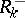 in which
in which 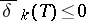 for any triangle
for any triangle 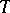 .
.
An even stronger property of concavity of the metric also holds. Namely, let 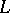 and
and 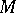 be shortests starting at the same point
be shortests starting at the same point 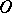 and let
and let 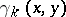 be the angle in the triangle
be the angle in the triangle 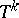 with sides
with sides 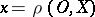 ,
, 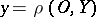 ,
, 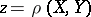 ,
, 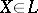 ,
, 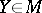 , in a space of constant curvature
, in a space of constant curvature 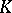 , opposite to the side
, opposite to the side  . In
. In  (locally) the angle
(locally) the angle  turns out to be a non-decreasing function (
turns out to be a non-decreasing function (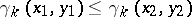 for
for 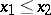 ,
,  , a
, a 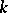 -concave metric). Hence one obtains the following local properties:
-concave metric). Hence one obtains the following local properties:
I) between any two shortests starting at the same point there exists an angle and even an "angle in the strong sense" 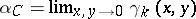 (so that, in particular, if
(so that, in particular, if  ,
,  );
);
II) for the angles 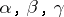 of a triangle in
of a triangle in 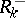 and the corresponding triangle
and the corresponding triangle 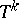 ,
,
 |
III) in 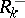 , if
, if  ,
, 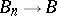 , then the shortests
, then the shortests 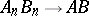 (thus, a shortest with given end points is unique in
(thus, a shortest with given end points is unique in 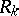 ).
).
Dual to spaces of curvature  are the spaces of curvature
are the spaces of curvature 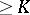 subject to the condition dual to
subject to the condition dual to 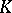 -concavity:
-concavity:
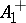 ) each point has a neighbourhood
) each point has a neighbourhood 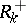 in which the angle
in which the angle 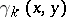 for two shortests
for two shortests 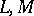 is a non-increasing function (a
is a non-increasing function (a 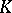 -concave metric, cf. also Convex metric).
-concave metric, cf. also Convex metric).
Similarly to spaces of curvature  , for spaces of curvature
, for spaces of curvature 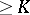 the following (local) properties analogous to I) and II) are valid: Between two shortests there exists an angle in the strong sense;
the following (local) properties analogous to I) and II) are valid: Between two shortests there exists an angle in the strong sense; 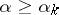 ,
, 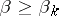 ,
,  for any triangle in
for any triangle in 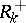 . Instead of III) the condition of non-overlapping of shortests or, which is the same, uniqueness of their extension holds: If
. Instead of III) the condition of non-overlapping of shortests or, which is the same, uniqueness of their extension holds: If 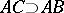 and
and 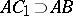 in
in 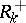 , then either
, then either 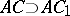 or
or 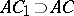 .
.
Thus, a space of bounded curvature is obtained by combining the conditions determining both classes of spaces — with curvature bounded from above and from below (moreover, on the left-hand side of inequality (3) there is no need to take 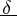 ). Condition
). Condition 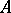 ) can be replaced, similar to
) can be replaced, similar to 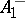 ), by the condition:
), by the condition:
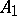 ) each point has a neighbourhood
) each point has a neighbourhood 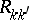 , where
, where  ,
,  for any triangle
for any triangle 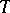 .
.
The above turns out to be equivalent to the following:
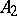 ) for any quadruple of points in
) for any quadruple of points in  there exists a quadruple of points with the same pairwise distances in a space of constant curvature
there exists a quadruple of points with the same pairwise distances in a space of constant curvature 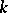 , where
, where 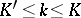 and
and  depends, in general, on the chosen quadruple of points in
depends, in general, on the chosen quadruple of points in 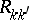 (see [10]).
(see [10]).
An example of a generalized Riemannian space of curvature 
 is a domain of a Riemannian space such that the Riemannian curvatures of all two-dimensional surface elements at all points of this domain are bounded from above by
is a domain of a Riemannian space such that the Riemannian curvatures of all two-dimensional surface elements at all points of this domain are bounded from above by 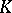 (from below by
(from below by 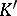 ).
).
A set 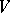 in a space with an internal metric is called convex if any two points
in a space with an internal metric is called convex if any two points  can be connected by a shortest
can be connected by a shortest 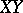 and if every such shortest lies in
and if every such shortest lies in  .
.
The following result [7] has been established: If a space 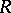 with an internal metric is obtained by glueing together of two spaces
with an internal metric is obtained by glueing together of two spaces 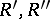 of curvatures
of curvatures  along convex sets
along convex sets 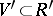 and
and 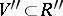 , then
, then 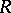 itself is a space of curvature
itself is a space of curvature 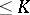 . The glueing condition is that
. The glueing condition is that 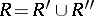 ,
, 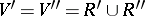 and the metrics of
and the metrics of  are induced by that of the space
are induced by that of the space 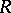 .
.
By definition, two curves  ,
, 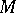 starting at a point
starting at a point 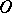 have the same direction at
have the same direction at 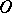 if the upper angle between them is equal to zero (if
if the upper angle between them is equal to zero (if 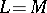 ,
, 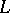 is said to have a definite oriented direction at
is said to have a definite oriented direction at 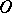 ). A direction at the point
). A direction at the point 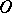 is defined as a class of curves having the same direction at
is defined as a class of curves having the same direction at 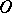 . The directions at the point
. The directions at the point 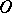 form a metric space in which the distance between two directions is determined by the upper angle between any two representatives of them. Such a space is called a space of directions at
form a metric space in which the distance between two directions is determined by the upper angle between any two representatives of them. Such a space is called a space of directions at 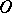 .
.
The following has been proved [5]: If the point  lies in a neighbourhood of a space of curvature
lies in a neighbourhood of a space of curvature 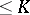 homeomorphic to
homeomorphic to 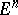 , then the space of directions at the point
, then the space of directions at the point  has curvature
has curvature 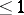 . In the general case it is not homeomorphic to the
. In the general case it is not homeomorphic to the  -dimensional sphere.
-dimensional sphere.
In the two-dimensional case, the theory of manifolds of curvature 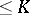 is included as a special case in the theory of manifolds of bounded curvature (see Two-dimensional manifold of bounded curvature). An example of a two-dimensional manifold of curvature
is included as a special case in the theory of manifolds of bounded curvature (see Two-dimensional manifold of bounded curvature). An example of a two-dimensional manifold of curvature  is a ruled surface in
is a ruled surface in  provided with an internal metric, i.e. the surface formed by the interior parts of shortests whose ends cut out two rectifiable curves
provided with an internal metric, i.e. the surface formed by the interior parts of shortests whose ends cut out two rectifiable curves  . If the curve
. If the curve 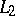 degenerates to a point
degenerates to a point 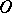 , the surface is called the cone of shortests spanned from the point
, the surface is called the cone of shortests spanned from the point 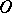 over the curve
over the curve 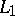 . If
. If 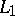 is a triangle
is a triangle 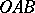 , then such a cone is called a surface triangle (see [3]).
, then such a cone is called a surface triangle (see [3]).
A mapping 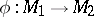 of metric spaces is called non-stretching if
of metric spaces is called non-stretching if  for any
for any 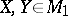 . A mapping
. A mapping 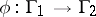 of a closed curve
of a closed curve  in
in 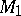 onto a closed curve
onto a closed curve 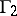 in
in 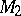 is called length-preserving if the lengths of corresponding arcs of
is called length-preserving if the lengths of corresponding arcs of 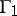 and
and 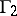 coincide under
coincide under 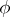 . Let
. Let 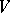 be a convex domain in a space of constant curvature
be a convex domain in a space of constant curvature 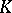 and
and 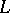 be the boundary contour of
be the boundary contour of 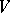 . The domain
. The domain 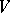 is said to majorize a closed curve
is said to majorize a closed curve 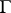 in a metric space
in a metric space  if there exists a non-stretching mapping from
if there exists a non-stretching mapping from 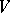 into
into  that is length-preserving from
that is length-preserving from 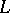 to
to 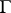 . The mapping itself is called majorizing.
. The mapping itself is called majorizing.
Let 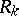 be a convex space with an internal metric; let
be a convex space with an internal metric; let  be the cone of shortests spanned over a closed rectifiable curve
be the cone of shortests spanned over a closed rectifiable curve 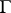 in
in 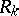 from a point
from a point 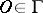 , and, moreover, let, if
, and, moreover, let, if 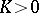 , the length
, the length 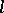 of
of 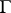 be less than
be less than 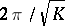 . Then in a space of constant curvature
. Then in a space of constant curvature 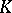 there exists a convex domain
there exists a convex domain 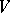 majorizing
majorizing 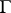 and such that
and such that 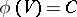 for the corresponding majorizing mapping
for the corresponding majorizing mapping 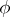 . This property is characteristic for spaces of curvature
. This property is characteristic for spaces of curvature  . The existence of a length-preserving non-stretching mapping of the contour
. The existence of a length-preserving non-stretching mapping of the contour  of
of 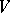 onto
onto 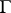 is already sufficient (see [8]).
is already sufficient (see [8]).
A continuous mapping 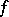 from a disc
from a disc 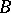 into a metric space
into a metric space 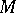 is called a surface in
is called a surface in 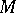 . Let
. Let 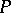 be a triangulated polygon, i.e. a complex of triangles
be a triangulated polygon, i.e. a complex of triangles  inscribed in
inscribed in 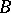 . To the triangle
. To the triangle 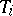 with vertices
with vertices  there corresponds the Euclidean triangle
there corresponds the Euclidean triangle 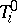 with sides equal to the distances between points
with sides equal to the distances between points 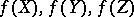 . Let
. Let 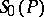 be the sum of the areas
be the sum of the areas 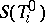 of all triangles
of all triangles 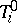 ; then the area
; then the area 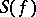 of the surface
of the surface 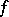 is defined (see [3]) as the limes inferior of
is defined (see [3]) as the limes inferior of  under the condition that the vertices of
under the condition that the vertices of 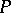 unboundedly contract in
unboundedly contract in 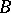 :
: 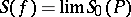 . This definition is modified as follows (see [6]). Instead of
. This definition is modified as follows (see [6]). Instead of 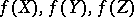 , the vertices
, the vertices 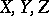 of the triangle
of the triangle 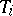 of the complex
of the complex 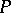 are put into correspondence with points
are put into correspondence with points 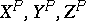 in
in 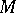 , where, moreover, to vertices of the complex
, where, moreover, to vertices of the complex 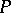 correspond the same points if and only if the images of the vertices under
correspond the same points if and only if the images of the vertices under 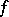 coincide. For the area
coincide. For the area 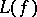 of the surface
of the surface 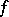 one takes the limes inferior of the sums of the areas of the Euclidean triangles
one takes the limes inferior of the sums of the areas of the Euclidean triangles 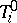 with sides equal to the distances between
with sides equal to the distances between 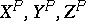 , under the additional assumption that
, under the additional assumption that 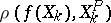 tends to zero for all vertices
tends to zero for all vertices 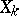 of the complex
of the complex 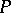 . One always has
. One always has 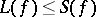 .
.
 ) If a sequence of surfaces
) If a sequence of surfaces 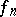 in
in 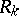 converges uniformly to a surface
converges uniformly to a surface  , then
, then
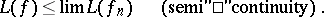 |
 ) If
) If 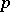 is a non-stretching mapping from
is a non-stretching mapping from 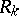 into
into  and
and  is a surface in
is a surface in 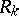 , then
, then
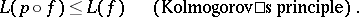 |
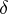 ) The area
) The area 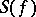 of a surface triangle
of a surface triangle 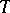 in
in 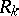 is not larger than the area of the corresponding triangle
is not larger than the area of the corresponding triangle  and is equal to it if and only if
and is equal to it if and only if 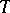 is isometric to
is isometric to  (the local property).
(the local property).
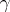 ) Under the conditions of the existence theorem for a majorizing mapping (see above), the area
) Under the conditions of the existence theorem for a majorizing mapping (see above), the area 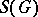 is not larger than the area of the disc of perimeter 1 in a space of constant curvature
is not larger than the area of the disc of perimeter 1 in a space of constant curvature 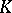 (the isoperimetric inequality) (see [3], [6]).
(the isoperimetric inequality) (see [3], [6]).
In [6] the Plateau problem on the existence of a surface of minimal area spanned over a closed curve 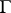 in
in 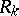 is solved. The following has been proved. Let
is solved. The following has been proved. Let 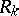 be a metrically-complete space of curvature
be a metrically-complete space of curvature 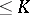 (for
(for 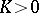 , the diameter
, the diameter 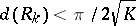 ) and let
) and let  be a closed Jordan curve in
be a closed Jordan curve in 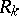 . Then there exists a surface
. Then there exists a surface 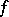 of minimal area
of minimal area 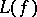 spanned over the curve
spanned over the curve 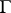 . Let
. Let 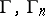 ,
, 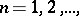 be closed Jordan curves in such a space and let
be closed Jordan curves in such a space and let 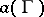 ,
, 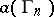 be the minimal areas of the surfaces spanned over
be the minimal areas of the surfaces spanned over 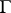 and
and  , respectively. If the
, respectively. If the 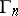 converge under some parametrizations uniformly to
converge under some parametrizations uniformly to 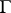 , then
, then 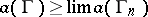 .
.
Two-dimensional manifolds with an indefinite metric of bounded curvature have been studied. The problem of a coordinate-free definition of multi-dimensional spaces with an indefinite metric of bounded curvature, and, in particular, of spaces in the general theory of relativity, has not yet been solved (1990).
References
| [1] | A.D. Aleksandrov, "Die innere Geometrie der konvexen Flächen" , Akademie Verlag (1955) (Translated from Russian) |
| [2] | A.D. Aleksandrov, "A theorem on triangles in metric space and certain applications" Trudy Mat. Inst. Steklov. , 38 (1951) pp. 5–23 (In Russian) |
| [3] | A.D. [A.D. Aleksandrov ] Alexandroff, "Über eine Verallgemeinerung der Riemannschen Geometrie" Schrift. Inst. Math. Deutsch. Akad. Wiss. , 1 (1957) pp. 33–84 |
| [4] | V.N. Berestovskii, "Introduction of a Riemann structure into certain metric spaces" Sib. Math. J. , 16 : 4 (1975) pp. 499–507 Sibirsk. Mat. Zh. , 16 : 4 (1975) pp. 651–662 |
| [5] | I.G. Nikolaev, "Space of directions at a point in a space of curvature not greater than  " Sib. Math. J. , 19 : 6 (1978) pp. 944–948 Sibirsk. Math. Zh. , 19 : 6 (1978) pp. 1341–1348 " Sib. Math. J. , 19 : 6 (1978) pp. 944–948 Sibirsk. Math. Zh. , 19 : 6 (1978) pp. 1341–1348 |
| [6] | I.G. Nikolaev, "Solution of Plateau's problem in spaces of curvature not greater than 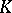 " Sib. Math. J. , 20 : 2 (1979) pp. 246–251 Sibirsk. Mat. Zh. , 20 : 2 (1979) pp. 345–353 " Sib. Math. J. , 20 : 2 (1979) pp. 246–251 Sibirsk. Mat. Zh. , 20 : 2 (1979) pp. 345–353 |
| [7] | Yu.G. Reshetnyak, "To the theory of spaces with curvature not greater than 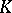 " Mat. Sb. , 52 : 3 (1960) pp. 789–798 (In Russian) " Mat. Sb. , 52 : 3 (1960) pp. 789–798 (In Russian) |
| [8] | Yu.G. Reshetnyak, "Inextensible mappings in a space of curvature no greater than 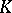 " Sib. Math. J. , 9 : 4 (1968) pp. 683–689 Sibirsk. Mat. Zh. , 9 : 4 (1968) pp. 918–927 " Sib. Math. J. , 9 : 4 (1968) pp. 683–689 Sibirsk. Mat. Zh. , 9 : 4 (1968) pp. 918–927 |
| [9] | I.G. Nikolaev, "Smoothness of the metric of spaces with two-sided bounded A.D. Aleksandrov curvature" Sib. Math. J. , 24 : 2 (1983) pp. 247–263 Sibersk. Mat. Zh. , 24 : 2 (1983) pp. 114–132 |
| [10] | V.N. Berestovskii, "Spaces with bounded curvature and distance geometry" Sib. Math. J. , 27 : 1 (1986) pp. 8–18 Sibersk. Mat. Zh. , 27 : 1 (1986) pp. 11–25 |
Comments
References
| [a1] | W. Rinow, "Die innere Geometrie der metrischen Räume" , Springer (1961) |
| [a2] | M. Gromov, "Structures métriques pour les variétés riemanniennes" , Cedec-Nathan (1981) (Translated from Russian) |
| [a3] | "Elie Cartan et les mathématiques d'aujourd'hui" Astérisque (1985) |
| [a4] | A.V. Pogorelov, "Intrinsic geometry of surfaces" , Amer. Math. Soc. (1973) (Translated from Russian) |
| [a5] | H. Busemann, "The geometry of geodesics" , Acad. Press (1955) |
Riemannian space, generalized. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Riemannian_space,_generalized&oldid=41327