Defective value
2020 Mathematics Subject Classification: Primary: 30D35 [MSN][ZBL]
of a meromorphic function 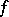
A complex number  (finite or infinite) whose defect
(finite or infinite) whose defect  (see below) is positive. Let the function
(see below) is positive. Let the function 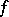 be defined in the disc
be defined in the disc  of the complex plane
of the complex plane 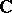 . The defect (or deficiency) of the value
. The defect (or deficiency) of the value  is
is
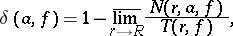 |
where  is Nevanlinna's characteristic function representing the growth of
is Nevanlinna's characteristic function representing the growth of 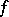 for
for 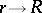 , and
, and
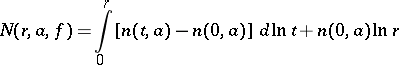 |
is the counting function; here, 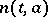 is the number of solutions of the equation
is the number of solutions of the equation 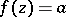 in
in  (counted with multiplicity). If
(counted with multiplicity). If 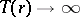 as
as  , then
, then 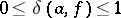 for all
for all 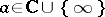 . If
. If 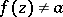 for any
for any  , then
, then 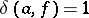 and
and  is a defective value; this equality also holds in some other cases (e.g.
is a defective value; this equality also holds in some other cases (e.g. 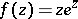 ,
,  and
and 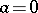 ).
).
If
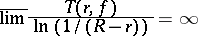 |
(or  is meromorphic throughout the plane), then
is meromorphic throughout the plane), then 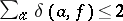 (the defect, or deficiency, relation), and the number of defective values for such
(the defect, or deficiency, relation), and the number of defective values for such 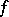 is at most countable. Otherwise, the set of defective values may be arbitrary; thus, for any sequences
is at most countable. Otherwise, the set of defective values may be arbitrary; thus, for any sequences  and
and 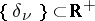 ,
, 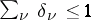 , it is possible to find an entire function
, it is possible to find an entire function  such that
such that 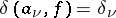 for all
for all  and there are no other defective values of
and there are no other defective values of 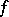 . Limitations imposed on the growth of
. Limitations imposed on the growth of 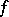 entail limitations on the defective values and their defects. For instance, a meromorphic function of order zero or an entire function of order
entail limitations on the defective values and their defects. For instance, a meromorphic function of order zero or an entire function of order 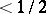 cannot have more than one defective value.
cannot have more than one defective value.
The number
 |
( meromorphic in
meromorphic in 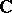 ) is known as the defect in the sense of Valiron. The set of numbers
) is known as the defect in the sense of Valiron. The set of numbers  for which
for which 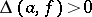 may have the cardinality of the continuum, but always has logarithmic capacity zero.
may have the cardinality of the continuum, but always has logarithmic capacity zero.
See also Exceptional value; Value-distribution theory.
References
| [1] | R. Nevanilinna, "Analytic functions" , Springer (1970) (Translated from German) |
| [2] | W.K. Hayman, "Meromorphic functions" , Oxford Univ. Press (1964) |
| [3] | A.A. Gol'dberg, I.V. Ostrovskii, "Value distribution of meromorphic functions" , Moscow (1970) (In Russian) |
Defective value. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Defective_value&oldid=34918