Differentiable manifold
A locally Euclidean space with a differentiable structure. Let  be a topological Hausdorff space.
be a topological Hausdorff space. 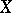 is known as a locally Euclidean space or as a topological manifold of dimension
is known as a locally Euclidean space or as a topological manifold of dimension  if for each point
if for each point  a neighbourhood
a neighbourhood  of
of  can be found that is homeomorphic to an open set of
can be found that is homeomorphic to an open set of  . The pair
. The pair  , where
, where  is this homeomorphism, is known as a local chart of
is this homeomorphism, is known as a local chart of  at
at  . Thus, to each point corresponds a selection of
. Thus, to each point corresponds a selection of  real numbers
real numbers 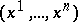 , known as the coordinates of
, known as the coordinates of  in the chart
in the chart 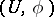 .
.
A family of charts  ,
,  , is known as an
, is known as an  -dimensional
-dimensional 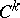 -atlas
-atlas 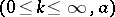 of
of  if a) the totality of all
if a) the totality of all  covers
covers  ,
,  ; and b) for any
; and b) for any 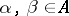 such that
such that 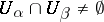 , the mapping
, the mapping
 |
belongs to the class of differentiability 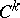 ;
; 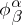 is a differentiable mapping with non-vanishing Jacobian and is known as a transformation of coordinates
is a differentiable mapping with non-vanishing Jacobian and is known as a transformation of coordinates  from the chart
from the chart 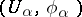 into the chart
into the chart 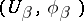 .
.
Two 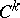 -atlases are said to be equivalent if their union is again a
-atlases are said to be equivalent if their union is again a 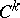 -atlas. The set of
-atlas. The set of  -atlases is thus subdivided into equivalence classes, known as
-atlases is thus subdivided into equivalence classes, known as 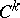 -structures; if
-structures; if 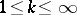 , they are known as differentiable (or smooth) structures, while if
, they are known as differentiable (or smooth) structures, while if  they are known as analytic structures. The topological manifold
they are known as analytic structures. The topological manifold  with a
with a  -structure is known as a
-structure is known as a 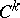 -manifold, or as a differentiable manifold of class
-manifold, or as a differentiable manifold of class 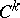 .
.
The concept of a differentiable structure may be introduced for an arbitrary set  by replacing the homeomorphisms
by replacing the homeomorphisms  by bijective mappings on open sets of
by bijective mappings on open sets of 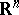 ; here, the topology of the
; here, the topology of the 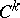 -manifold is described as the topology of the union, constructed from an arbitrary atlas of the corresponding structure. In such a case
-manifold is described as the topology of the union, constructed from an arbitrary atlas of the corresponding structure. In such a case  -dimensional manifolds clearly have an
-dimensional manifolds clearly have an  -dimensional
-dimensional 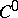 -structure.
-structure.
Problems of analytical and algebraic geometry make it necessary to consider in the definition of a differentiable structure not only the space  , but also more general spaces, such as
, but also more general spaces, such as 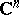 or even
or even 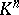 where
where 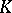 is a complete non-discretely normed field. Thus, if
is a complete non-discretely normed field. Thus, if 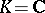 , the corresponding
, the corresponding  -structure,
-structure,  , invariably proves to be a
, invariably proves to be a 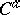 -structure and is called complex-analytic, or simply complex, while the corresponding differentiable manifold is known as a complex manifold. Such a manifold also carries a natural real
-structure and is called complex-analytic, or simply complex, while the corresponding differentiable manifold is known as a complex manifold. Such a manifold also carries a natural real 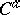 -structure.
-structure.
Any 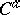 -manifold contains a
-manifold contains a 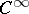 -structure, and there is a
-structure, and there is a  -structure on a
-structure on a 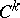 -manifold,
-manifold, 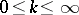 , if
, if 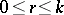 . Conversely, any paracompact
. Conversely, any paracompact 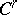 -manifold,
-manifold,  , may be provided with a
, may be provided with a  -structure compatible with the given one, and this structure is unique, up to an isomorphism (see below). It may happen, however, that a
-structure compatible with the given one, and this structure is unique, up to an isomorphism (see below). It may happen, however, that a 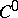 -manifold cannot be provided with a
-manifold cannot be provided with a  -structure (i.e. there exist non-smoothable manifolds, cf. Non-smoothable manifold), and even if it can be provided with such a structure, the structure need not be unique. For example, the number
-structure (i.e. there exist non-smoothable manifolds, cf. Non-smoothable manifold), and even if it can be provided with such a structure, the structure need not be unique. For example, the number 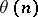 of
of 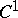 non-isomorphic
non-isomorphic 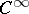 structures on the
structures on the  -dimensional sphere is:
-dimensional sphere is:
<tbody> </tbody>
|
Let 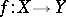 be a continuous mapping of
be a continuous mapping of 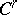 -manifolds
-manifolds 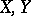 ; it is known as a
; it is known as a 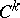 -morphism (or as a
-morphism (or as a  -mapping,
-mapping,  , or as a mapping of class
, or as a mapping of class 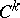 ) of differentiable manifolds if for any pair of charts
) of differentiable manifolds if for any pair of charts  on
on 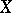 and
and 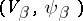 on
on 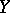 such that
such that 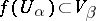 , the mapping
, the mapping
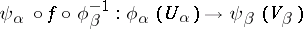 |
belongs to the class 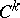 . A bijective mapping
. A bijective mapping 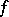 such that it and
such that it and  are
are 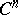 -mappings is called a
-mappings is called a  -isomorphism (or a diffeomorphism of class
-isomorphism (or a diffeomorphism of class 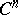 ). In such a case
). In such a case 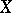 and
and 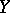 and their determining
and their determining 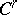 -structures are said to be
-structures are said to be 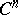 -diffeomorphic.
-diffeomorphic.
A subspace 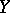 of an
of an  -dimensional
-dimensional 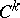 -manifold
-manifold 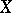 is called a
is called a 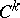 -submanifold of dimension
-submanifold of dimension 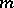 in
in 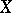 if for any point
if for any point 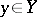 there exists a neighbourhood
there exists a neighbourhood  of it and a chart
of it and a chart 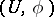 of the
of the 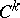 -structure
-structure  such that
such that 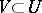 and
and 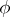 induces a homeomorphism of
induces a homeomorphism of 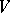 onto the intersection of
onto the intersection of 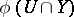 with the closed subspace
with the closed subspace 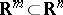 ; in other words, there exists a chart with coordinates
; in other words, there exists a chart with coordinates  such that
such that 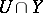 is defined by the relations
is defined by the relations 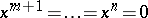 .
.
A mapping 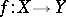 is said to be a
is said to be a 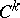 -imbedding if
-imbedding if 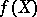 is a
is a 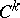 -submanifold in
-submanifold in 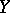 and if
and if  is a
is a 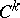 -diffeomorphism. Any
-diffeomorphism. Any  -dimensional
-dimensional 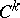 -manifold permits an imbedding in
-manifold permits an imbedding in 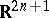 and even in
and even in  . Moreover, the set of such imbeddings is everywhere dense in the space of mappings
. Moreover, the set of such imbeddings is everywhere dense in the space of mappings 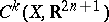 with respect to the compact-open topology. Thus, regarding a differentiable manifold as a submanifold of a Euclidean space is one of the ways of interpreting the theory of differentiable manifolds; for example, the above theorems on
with respect to the compact-open topology. Thus, regarding a differentiable manifold as a submanifold of a Euclidean space is one of the ways of interpreting the theory of differentiable manifolds; for example, the above theorems on 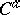 -structures can be proved in this manner.
-structures can be proved in this manner.
There are two fundamental problems in the topology of differentiable manifolds (which is also referred to as differential topology). The first problem is the classification of differentiable manifolds. There exist three main classes of differentiable manifolds — closed (or compact) manifolds, compact manifolds with boundary and open manifolds. Important invariants by which differentiable manifolds are distinguished are the homotopy type and the tangent bundle, in particular the characteristic classes (cf. Characteristic class). Using these a classification of smooth structures for simply-connected manifolds of given homotopy type has been given. Another invariant — the bordism class of a differentiable manifold — was used in solving the generalized Poincaré conjecture, in the study of fixed points under the action of a group on a manifold, etc. This involved the introduction of differentiable structures on manifolds with boundary and of a smoothing apparatus. Finally, methods of algebraic topology also proved useful in this context, since, for example, they permitted to establish that any  -manifold can be triangulated.
-manifold can be triangulated.
The second problem is the classification of mappings of differentiable manifolds. The first class to be considered are immersions, which are a generalization of imbeddings; their classification is reduced to a homotopy problem, as distinct from imbeddings, which have not yet (1987) been completely classified (cf. Topology of imbeddings), and submersions, or fibrations, of one differentiable manifold into another. In particular, the concept of a transversal mapping along a submanifold plays an important role in problems of stability and in the study of typical singularities of mappings. The existence of transversal mappings is ensured by theorems such as Sard's theorem (cf. Sard theorem). All this, and problems in differential dynamics, dealing with the structure of various groups of diffeomorphisms (cf. Diffeomorphism), in particular of integral trajectories and singular points of vector fields on differentiable manifolds (dynamical systems), as well as the various equivalence relationships — isotopy, topological and 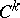 -conjugacy, etc. — makes it necessary to study finite-dimensional spaces
-conjugacy, etc. — makes it necessary to study finite-dimensional spaces 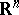 together with arbitrary Banach (or Hilbert) spaces and to determine corresponding differentiable structures. This implies finding additional conditions that are reasonable from the point of view of applications, e.g., a differentiable manifold is separable if and only if the coordinate transformations have a closed graph. In general, infinite-dimensional manifolds provided with such a structure — known as Banach or Hilbert manifolds, respectively, manifolds of mappings of finite-dimensional manifolds being their typical example — are a useful outcome of studies and geometrical interpretation of problems of approximation of mappings (as in the imbedding theorem above), in the analysis of loop spaces (a suitable domain for the construction of Morse theory, cf. Loop space), etc.
together with arbitrary Banach (or Hilbert) spaces and to determine corresponding differentiable structures. This implies finding additional conditions that are reasonable from the point of view of applications, e.g., a differentiable manifold is separable if and only if the coordinate transformations have a closed graph. In general, infinite-dimensional manifolds provided with such a structure — known as Banach or Hilbert manifolds, respectively, manifolds of mappings of finite-dimensional manifolds being their typical example — are a useful outcome of studies and geometrical interpretation of problems of approximation of mappings (as in the imbedding theorem above), in the analysis of loop spaces (a suitable domain for the construction of Morse theory, cf. Loop space), etc.
Differentiable manifolds form a natural base for developing differential geometry. Supplementary infinitesimal structures — orientation, metric, connections, etc. — are introduced on differentiable manifolds, after which a study is made of the objects which are invariant with respect to the group of diffeomorphisms which preserve the supplementary structure. Conversely, the use of a specific structure permits one to study the structure of the differential manifold itself. The simplest example is the expression of the characteristic classes in terms of the curvature of a differentiable manifold with a linear connection.
References
| [1] | L.S. Pontryagin, "Smooth manifolds and their applications in homotopy theory" , Moscow (1976) (In Russian) MR0445517 Zbl 0084.19002 |
| [2] | N. Bourbaki, "Elements of mathematics. Differentiable and analytic manifolds" , Addison-Wesley (1966) (Translated from French) MR0205211 MR0205210 |
| [3] | G. de Rham, "Differentiable manifolds" , Springer (1984) (Translated from French) Zbl 0534.58003 |
| [4] | S. Lang, "Introduction to differentiable manifolds" , Interscience (1967) pp. App. III MR1931083 MR1532744 MR0155257 Zbl 1008.57001 Zbl 0103.15101 |
| [5] | V.A. Rokhlin, D.B. Fuks, "Beginner's course in topology. Geometric chapters" , Springer (1984) (Translated from Russian) MR759162 |
| [6] | H. Whitney, "Geometric integration theory" , Princeton Univ. Press (1957) MR0087148 Zbl 0083.28204 |
| [7] | M.M. Postnikov, "Introduction to Morse theory" , Moscow (1971) (In Russian) MR0315739 |
| [8] | R. Narasimhan, "Analysis on real and complex manifolds" , Springer (1971) MR0832683 MR0346855 MR0251745 Zbl 0583.58001 Zbl 0188.25803 |
| [9] | R.O. Wells jr., "Differential analysis on complex manifolds" , Springer (1980) MR0608414 Zbl 0435.32004 |
| [10] | M. Golubitskii, V. Guillemin, "Stable mappings and their singularities" , Springer (1973) MR0467801 |
| [11] | P. Bröcker, L. Lander, "Differentiable germs and catastrophes" , Cambridge Univ. Press (1975) MR0494220 Zbl 0302.58006 |
| [12] | Z. Nitecki, "Differentiable dynamics. An introduction to the orbit structure of diffeomorphisms" , M.I.T. (1971) MR0649788 Zbl 0246.58012 |
| [13] | S. Sternberg, "Lectures on differential geometry" , Prentice-Hall (1964) MR0193578 Zbl 0129.13102 |
| [14] | C. Godbillon, "Géométrie différentielle et mécanique analytique" , Hermann (1969) MR0242081 Zbl 0653.53001 Zbl 0284.53018 |
| [15] | R. Sulanke, P. Wintgen, "Differentialgeometrie und Faserbündel" , Birkhäuser (1972) MR0413153 Zbl 0327.53020 Zbl 0271.53035 |
See also the references to Differential topology.
Comments
Often the property of being paracompact is taken to be part of the definition of a topological or differentiable manifold. A space that is locally Euclidean is not necessarily paracompact.
References
| [a1] | J.W. Milnor, "Morse theory" , Princeton Univ. Press (1963) MR0163331 Zbl 0108.10401 |
| [a2] | J.W. Milnor, "Toplogy from the differentiable viewpoint" , Univ. Virginia Press (1965) |
| [a3] | J.W. Milnor, J.D. Stasheff, "Characteristic classes" , Princeton Univ. Press (1974) MR0440554 Zbl 0298.57008 |
| [a4] | M.R. Munkres, "Elementary differential topology" , Princeton Univ. Press (1963) MR0163320 Zbl 0107.17201 |
| [a5] | M.W. Hirsch, "Differential topology" , Springer (1976) pp. Chapt. 5, Sect. 3 MR0448362 Zbl 0356.57001 |
| [a6] | C.T.C. Wall, "Surgery on compact manifolds" , Acad. Press (1970) MR0431216 Zbl 0219.57024 |
| [a7] | D.S. Freed, K.K. Uhlenbeck, "Instantons and four-manifolds" , Springer (1984) MR0757358 Zbl 0559.57001 |
Differentiable manifold. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Differentiable_manifold&oldid=34226

