Density theorems

The general name for theorems that give upper bounds for the number $N(\sigma,T,\chi)$ of zeros $\rho=\beta+i\gamma$ of Dirichlet $L$-functions

$$L(s,\chi)=\sum_{n=1}^\infty\frac{\chi(n,k)}{n^s},$$

where $s=\sigma+it$ and $\chi(n,k)$ is a character modulo $k$, in the rectangle $1/2<\sigma\leq\beta<1$, $|\gamma|\leq T$. In the case $k=1$, one gets density theorems for the number of zeros of the Riemann zeta-function

$$\zeta(s)=\sum_{n=1}^\infty\frac{1}{n^s}.$$

The density theorems for $L$-functions with $k\neq1$ are more complicated than those for the Riemann zeta-function. As $T$ and $k$ increase, one obtains bounds depending on these parameters. The parameter $k$ plays a decisive part in applications.

The significance of density theorems is evident from the relations enabling one to estimate the residual term in the formula for the number of prime numbers $p$ belonging to an arithmetic progression $km+l$, $1\leq l\leq k$, $(l,k)=1$, $m=0,1,\ldots,$ and not exceeding $x$, as a function of $N(\sigma,T,\chi)$.

Since $N(\sigma,T,\chi)$ does not increase with $\sigma$ and $N(1,T,\chi)=0$, the purpose of density theorems is to obtain bounds that converge most rapidly to zero as $\sigma\to1$. In turn, these bounds are substantially supplemented by results on the absence of zeros for Dirichlet $L$-functions in neighbourhoods of the straight line $\sigma=1$, obtained using the Hardy–Littlewood–Vinogradov circle method. In this way it has been possible to obtain strong bounds for the amount of even numbers $n\leq x$ that cannot be represented as the sum of two prime numbers.

Yu.V. Linnik obtained the first density theorems providing bounds for $N(\sigma,T,\chi)$ for an individual character $\chi$ and averaged bounds over all characters modulo $a$, given $k$. Subsequent substantial improvements of density theorems were obtained by A.I. Vinogradov and E. Bombieri, who used bounds on $N(\sigma,T,\chi)$ averaged over all moduli $k\leq Q$ and over all primitive characters modulo $a$, given $k$, in proving a theorem on the average distribution of prime numbers in arithmetic progressions (for $Q=\sqrt x/(\ln x)^c$). The Vinogradov–Bombieri theorem enables one to replace the generalized Riemann hypothesis in various classical problems in additive number theory. There are also various other improvements of density theorems.

References

[1]	K. Prachar, "Primzahlverteilung" , Springer (1957)
[2]	H. Davenport, "Multiplicative number theory" , Springer (1980)
[3]	A.F. Lavrik, "A survey of Linnik's large sieve and the density theory of zeros of 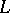-functions" Russian Math. Surveys , 35 : 2 (1980) pp. 63–76 Uspekhi Mat. Nauk , 35 : 2 (1980) pp. 55–65

Comments

For extra references see Density method. Cf. also Distribution of prime numbers.

For the Vinogradov–Bombieri theorem see Density hypothesis.

References

[a1]	A. Ivic, "The Riemann zeta-function" , Wiley (1985)