Torsion group

A group in which every element has finite order. Any periodic Abelian group splits into a direct sum of primary groups with respect to distinct prime numbers. See Burnside problem on periodic groups for finiteness conditions of periodic groups.

Comments

A periodic group is also called a torsion group. For any group 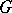, its torsion subgroup is defined by 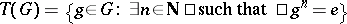. It is a normal subgroup and the quotient 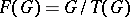is the torsion-free quotient group of . Both and 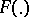are functors.

References

[a1]	A.G. Kurosh, "The theory of groups" , 1–2 , Chelsea (1955–1956) (Translated from Russian)
[a2]	P. Hall, "The theory of groups" , Macmillan (1959) pp. Chapt. 10
[a3]	D.J.S. Robinson, "Finiteness condition and generalized soluble groups" , I , Springer (1972)