Stanley-Reisner ring
Stanley–Reisner face ring, face ring
The Stanley–Reisner ring of a simplicial complex  over a field
over a field  is the quotient ring
is the quotient ring
 |
where  are the vertices of
are the vertices of  ,
,  denotes the polynomial ring over
denotes the polynomial ring over  in the variables
in the variables  , and
, and  is the ideal in
is the ideal in 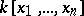 generated by the non-faces of
generated by the non-faces of 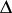 , i.e.,
, i.e.,
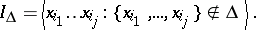 |
The support of any monomial in 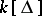 is a face of
is a face of  . In particular, the square-free monomials of
. In particular, the square-free monomials of 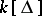 correspond bijectively to the faces of
correspond bijectively to the faces of 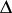 , and are therefore called the face-monomials
, and are therefore called the face-monomials
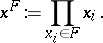 |
One may thus write 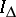 more compactly as
more compactly as  .
.
It is easy to verify that the Krull dimension of 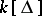 (cf. also Dimension) is one greater than the dimension of
(cf. also Dimension) is one greater than the dimension of 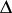 (
( ).
).
Recall that the Hilbert series of a finitely-generated 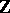 -graded module
-graded module 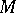 over a finitely-generated
over a finitely-generated 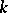 -algebra is defined by
-algebra is defined by 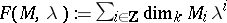 . The Hilbert series of
. The Hilbert series of 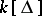 may be described from the combinatorics of
may be described from the combinatorics of 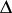 . Let
. Let 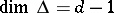 , let
, let  , and call
, and call 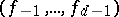 the
the 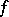 -vector of
-vector of 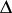 . Then
. Then
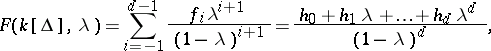 |
where the sequence 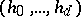 , called the
, called the 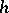 -vector of
-vector of 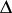 , may be derived from the
, may be derived from the 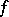 -vector of
-vector of 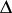 (and vice versa) by the equation
(and vice versa) by the equation
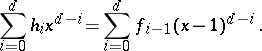 |
The mapping from 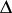 to
to 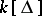 allows properties defined for rings to be naturally extended to simplicial complexes. The most well-known and useful example is Cohen–Macaulayness: A simplicial complex
allows properties defined for rings to be naturally extended to simplicial complexes. The most well-known and useful example is Cohen–Macaulayness: A simplicial complex  is defined to be Cohen–Macaulay (over the field
is defined to be Cohen–Macaulay (over the field 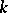 ) when
) when 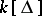 is Cohen–Macaulay (cf. also Cohen–Macaulay ring). The utility of this extension is demonstrated in the proof that if (the geometric realization of) a simplicial complex is homeomorphic to a sphere, then its
is Cohen–Macaulay (cf. also Cohen–Macaulay ring). The utility of this extension is demonstrated in the proof that if (the geometric realization of) a simplicial complex is homeomorphic to a sphere, then its 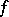 -vector satisfies a condition called the upper bound conjecture (for details, see [a1], Sect. II.3,4). The statement of this result requires no algebra, but the proof relies heavily upon the Stanley–Reisner ring and Cohen–Macaulayness. Many other applications of the Stanley–Reisner ring may be found in [a1], Chaps. II, III.
-vector satisfies a condition called the upper bound conjecture (for details, see [a1], Sect. II.3,4). The statement of this result requires no algebra, but the proof relies heavily upon the Stanley–Reisner ring and Cohen–Macaulayness. Many other applications of the Stanley–Reisner ring may be found in [a1], Chaps. II, III.
Finally, there is an anti-commutative version of the Stanley–Reisner ring, called the exterior face ring or indicator algebra, in which the polynomial ring  in the definition of
in the definition of 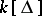 is replaced by the exterior algebra
is replaced by the exterior algebra 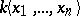 .
.
References
| [a1] | R. Stanley, "Combinatorics and commutative algebra" , Birkhäuser (1996) (Edition: Second) |
Stanley-Reisner ring. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Stanley-Reisner_ring&oldid=23037