Mackey-Borel structure
A Borel structure (i.e. a Borel system of sets) on the spectrum 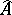 of a separable
of a separable 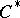 -algebra
-algebra 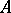 (cf. also Spectrum of a
(cf. also Spectrum of a 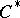 -algebra), defined as follows. Let
-algebra), defined as follows. Let 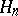 ,
, 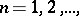 be a Hilbert space of dimension
be a Hilbert space of dimension  , and let
, and let  be the set of non-zero irreducible representations (cf. Irreducible representation) of the
be the set of non-zero irreducible representations (cf. Irreducible representation) of the 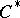 -algebra
-algebra 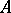 on
on 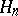 equipped with the topology of pointwise convergence in the weak topology. Let on
equipped with the topology of pointwise convergence in the weak topology. Let on 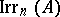 be given the Borel structure generated by its topology (that is, the smallest Borel structure relative to which all mappings
be given the Borel structure generated by its topology (that is, the smallest Borel structure relative to which all mappings 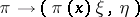 ,
, 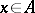 ,
, 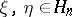 ,
, 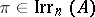 , are Borel functions) and let
, are Borel functions) and let 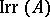 be the union of the subspaces
be the union of the subspaces 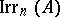 ,
,  provided with the Borel structure such that a subset of
provided with the Borel structure such that a subset of 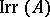 is a Borel set if and only if its intersection with each
is a Borel set if and only if its intersection with each 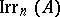 belongs to the Borel structure on the latter. Let
belongs to the Borel structure on the latter. Let 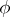 be the mapping of the Borel space
be the mapping of the Borel space 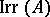 into the spectrum
into the spectrum 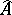 of
of 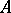 which maps a representation to its unitary equivalence class. The Borel structure on
which maps a representation to its unitary equivalence class. The Borel structure on 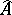 generated by the sets whose inverse images under
generated by the sets whose inverse images under  are Borel sets in
are Borel sets in  is called the Mackey–Borel structure on
is called the Mackey–Borel structure on 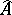 . The Mackey–Borel structure contains all sets of the Borel structure generated by the topology of
. The Mackey–Borel structure contains all sets of the Borel structure generated by the topology of 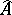 ; each point of
; each point of 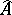 is a Borel set in the Mackey–Borel structure. The following conditions are equivalent: 1) the Mackey–Borel structure is standard (i.e. it is isomorphic as a Borel structure to the Borel structure generated by the topology of some complete separable metric space); 2) the Mackey–Borel structure coincides with the Borel structure generated by the topology on
is a Borel set in the Mackey–Borel structure. The following conditions are equivalent: 1) the Mackey–Borel structure is standard (i.e. it is isomorphic as a Borel structure to the Borel structure generated by the topology of some complete separable metric space); 2) the Mackey–Borel structure coincides with the Borel structure generated by the topology on 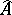 ; 3) the Mackey–Borel structure on
; 3) the Mackey–Borel structure on 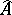 is countably separated; and 4) if
is countably separated; and 4) if 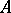 is a
is a 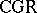 -algebra, then a Mackey–Borel structure can also be introduced on the quasi-spectrum of a separable
-algebra, then a Mackey–Borel structure can also be introduced on the quasi-spectrum of a separable 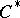 -algebra.
-algebra.
References
| [1] | J. Dixmier, " algebras" , North-Holland (1977) (Translated from French) algebras" , North-Holland (1977) (Translated from French) |
| [2] | L.T. Gardner, "On the Mackey Borel structure" Canad. J. Math. , 23 : 4 (1971) pp. 674–678 |
| [3] | H. Halpern, "Mackey Borel structure for the quasi-dual of a separable  -algebra" Canad. J. Math. , 26 : 3 (1974) pp. 621–628 -algebra" Canad. J. Math. , 26 : 3 (1974) pp. 621–628 |
Comments
References
| [a1] | W. Arveson, "An invitation to 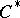 -algebras" , Springer (1976) pp. Chapts. 3–4 -algebras" , Springer (1976) pp. Chapts. 3–4 |
Mackey-Borel structure. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Mackey-Borel_structure&oldid=22785