Freudenthal-Kantor triple system
A triple system considered for constructing all simple Lie algebras (cf. Lie algebra), and introduced as an algebraic system which is a generalization both of the algebraic systems appearing in the metasymplectic geometry developed by H. Freudenthal and of a generalized Jordan triple system of second order developed by I.L. Kantor.
Recall that a triple system is a vector space 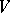 over a field
over a field 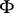 together with a
together with a 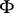 -trilinear mapping
-trilinear mapping 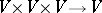 .
.
For 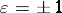 , a vector space
, a vector space 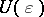 over a field
over a field 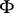 with the trilinear product
with the trilinear product 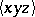 is called a Freudenthal–Kantor triple system if
is called a Freudenthal–Kantor triple system if
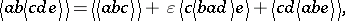 | (a1) |
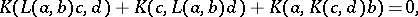 | (a2) |
where 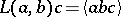 and
and 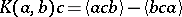 .
.
In particular, a Freudenthal–Kantor triple system 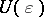 is said to be balanced if there exists a bilinear form
is said to be balanced if there exists a bilinear form  such that
such that 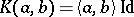 , for all
, for all 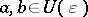 .
.
This balancing property is closely related to metasymplectic geometry.
Note that if  and
and 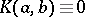 (identically), then the Freudenthal–Kantor triple system reduces to a Jordan triple system.
(identically), then the Freudenthal–Kantor triple system reduces to a Jordan triple system.
As the notion of a Freudenthal–Kantor triple system includes the notions of a generalized Jordan triple system of second order, a structurable algebra, and an Allison–Hein triple system, it is useful in obtaining all Lie algebras, without the use of root systems and Cartan matrices.
Let 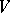 be a vector space with a bilinear form
be a vector space with a bilinear form 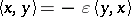 . Then
. Then  is a Freudenthal–Kantor triple system with respect to the triple product
is a Freudenthal–Kantor triple system with respect to the triple product 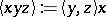 . In particular, it is important that the linear span
. In particular, it is important that the linear span 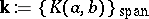 of the set
of the set 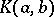 makes a Jordan triple system of
makes a Jordan triple system of 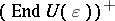 with respect to the triple product
with respect to the triple product 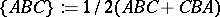 .
.
Let 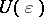 be a Freudenthal–Kantor triple system. The vector space
be a Freudenthal–Kantor triple system. The vector space  becomes a Lie triple system with respect to the triple product defined by
becomes a Lie triple system with respect to the triple product defined by
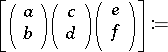 |
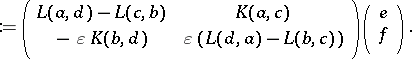 |
Using this, one can obtain the Lie triple system 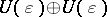 associated with
associated with 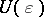 ; it is denoted be
; it is denoted be 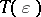 .
.
Using the concept of the standard embedding Lie algebra 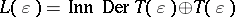 associated with a Lie triple system
associated with a Lie triple system 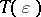 , one can obtain the construction of
, one can obtain the construction of 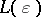 associated with a Freudenthal–Kantor triple system
associated with a Freudenthal–Kantor triple system  . In fact, put
. In fact, put
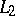 equal to the linear span of the endomorphisms
equal to the linear span of the endomorphisms
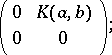 |
 ;
;
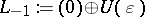 ;
;
 equal to the linear span of the endomorphisms
equal to the linear span of the endomorphisms
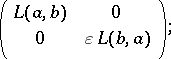 |
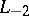 equal to the linear span of the endomorphisms
equal to the linear span of the endomorphisms
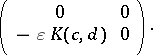 |
Then one obtains the decomposition
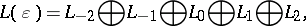 |
and, more precisely,
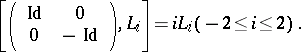 |
These results imply the dimensional formula
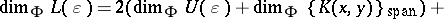 |
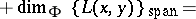 |
 |
This algebra 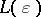 is called the Lie algebra associated with
is called the Lie algebra associated with 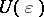 .
.
The concepts of a triple system and a supertriple system are important in the theory of quarks and Yang–Baxter equations.
Note that a "triple system" in the sense discussed above is totally different from "triple system" in combinatorics (see, e.g., Steiner system).
References
| [a1] | H. Freudenthal, "Beziehungen der 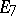 und und 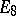 zur Oktavenebene I–II" Indag. Math. , 16 (1954) pp. 218–230; 363–386 zur Oktavenebene I–II" Indag. Math. , 16 (1954) pp. 218–230; 363–386 |
| [a2] | N. Kamiya, "The construction of all simple Lie algebras over 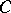 from balanced Freudenthal–Kantor triple systems" , Contributions to General Algebra , 7 , Hölder–Pichler–Tempsky, Wien (1991) pp. 205–213 from balanced Freudenthal–Kantor triple systems" , Contributions to General Algebra , 7 , Hölder–Pichler–Tempsky, Wien (1991) pp. 205–213 |
| [a3] | N. Kamiya, "On Freudenthal–Kantor triple systems and generalized structurable algebras" , Non-Associative Algebra and Its Applications , Kluwer Acad. Publ. (1994) pp. 198–203 |
| [a4] | N. Kamiya, S. Okubo, "On 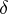 -Lie supertriple systems associated with -Lie supertriple systems associated with  -Freudenthal–Kantor supertriple systems" Proc. Edinburgh Math. Soc. , 43 (2000) pp. 243–260 -Freudenthal–Kantor supertriple systems" Proc. Edinburgh Math. Soc. , 43 (2000) pp. 243–260 |
| [a5] | I.L. Kantor, "Models of exceptional Lie algebras" Soviet Math. Dokl. , 14 (1973) pp. 254–258 |
| [a6] | S. Okubo, "Introduction to octonion and other non-associative algebras in physics" , Cambridge Univ. Press (1995) |
| [a7] | K. Yamaguti, "On the metasymplectic geometry and triple systems" Surikaisekikenkyusho Kokyuroku, Res. Inst. Math. Sci. Kyoto Univ. , 306 (1977) pp. 55–92 (In Japanese) |
Freudenthal-Kantor triple system. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Freudenthal-Kantor_triple_system&oldid=22471