Jacobson radical

of a ring

The ideal of an associative ring (cf. Associative rings and algebras) which satisfies the following two requirements: 1) is the largest quasi-regular ideal in (a ring is called quasi-regular if the equation is solvable for any of its elements ); and 2) the quotient ring contains no non-zero quasi-regular ideals. The radical was introduced and studied in detail in 1945 by N. Jacobson [1].

The Jacobson radical always exists and may be characterized in very many ways: 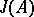is the intersection of the kernels of all irreducible representations of the ring ; it is the intersection of all modular maximal right ideals (cf. Modular ideal); it is the intersection of all modular maximal left ideals; it contains all quasi-regular one-sided ideals; it contains all one-sided nil ideals; etc. If is an ideal of 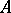, then 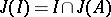. If is the ring of all matrices of order over 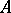, then

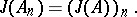If the following -composition is introduced on the associative ring :

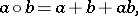then the radical 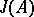in the semi-group 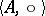will be a subgroup with respect to the composition .

There are no non-zero irreducible finitely-generated modules over a quasi-regular associative ring (i.e. an associative ring coinciding with its own Jacobson radical), but there exist simple associative quasi-regular rings. The Jacobson radical of the associative ring 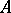is zero if and only if 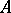is a subdirect sum of primitive rings (cf. Primitive ring).

References

[1]	N. Jacobson, "Structure of rings" , Amer. Math. Soc. (1956)

Comments

The Jacobson radical is the intersection of the right primitive ideals. It is also the intersection of the left primitive ideals. This is perhaps the most frequently occurring definition. Modular ideals are also called regular ideals. If has a unit element, then all ideals are regular, so that in this case the Jacobson radical is the intersection of all right maximal ideals and also the intersection of all left maximal ideals. Nakayama's lemma says that if is a finitely-generated non-zero right 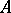-module, then .

References

[a1]	J.C. McConnell, J.C. Robson, "Noncommutative Noetherian rings" , Wiley (1987)
[a2]	I.N. Herstein, "Noncommutative rings" , Math. Assoc. Amer. (1968)