Synge world function
In a manifold 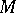 with Riemannian metric
with Riemannian metric 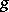 , a natural object characterizing the geometry is the distance
, a natural object characterizing the geometry is the distance  between any two points
between any two points 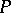 ,
,  in
in  , defined as the infimum of the length of all curves connecting the points. This does not itself generalize to pseudo-Riemannian manifolds (cf. Pseudo-Riemannian space; Pseudo-Riemannian geometry). In that case, however, for any
, defined as the infimum of the length of all curves connecting the points. This does not itself generalize to pseudo-Riemannian manifolds (cf. Pseudo-Riemannian space; Pseudo-Riemannian geometry). In that case, however, for any  there is an open neighbourhood
there is an open neighbourhood 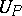 such that for
such that for 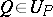 the two points are joined by a unique geodesic
the two points are joined by a unique geodesic 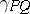 in
in 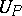 . If
. If  is an affine parameter with
is an affine parameter with  ,
,  , and
, and 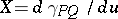 is the corresponding tangent vector, then the quantity
is the corresponding tangent vector, then the quantity
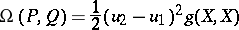 |
is independent of the choice of affine parameter and is well defined for both Riemannian and pseudo-Riemannian metrics 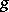 . In the Riemannian case it reduces to
. In the Riemannian case it reduces to 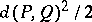 , while in the case of general relativity (pseudo-Riemannian metric of Lorentz signature) it evaluates to zero if
, while in the case of general relativity (pseudo-Riemannian metric of Lorentz signature) it evaluates to zero if 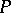 ,
, 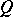 are null-separated and to plus or minus half the square of the space- or time-separation of the points otherwise. This function was introduced by H.S. Ruse [a3] and popularized by J.L. Synge [a4] as the world function for general relativity.
are null-separated and to plus or minus half the square of the space- or time-separation of the points otherwise. This function was introduced by H.S. Ruse [a3] and popularized by J.L. Synge [a4] as the world function for general relativity.
If 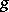 is differentiable of class
is differentiable of class  (
(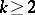 ), then
), then  is of class
is of class 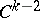 on the manifold
on the manifold 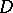 of pairs
of pairs 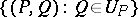 . On the diagonal
. On the diagonal  the first few partial derivatives with respect to the coordinates of the first argument are given, in index notation, by
the first few partial derivatives with respect to the coordinates of the first argument are given, in index notation, by
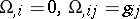 |
 |
where 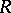 is the Riemann tensor of
is the Riemann tensor of 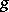 (with sign convention
(with sign convention  ).
).
Since the world function is physically interpretable in terms of the squares of lengths and times, and is linked by the above formulas to the curvature, it could be used by Synge [a4] as a systematic tool in deriving the basic formulas of differential geometry together with their physical interpretation. In this spirit it was used by C.J.S. Clarke and F. de Felice [a1] to express the curvature corrections to radar-ranging measurements; further formulas of this kind have been presented in [a2].
References
| [a1] | C.J.S. Clarke, F. de Felice, "Relativity on curved manifolds" , Cambridge Univ. Press (1990) |
| [a2] | J.M. Gambi, P. Romero, A. Sanmiguel, F. Vicente, "Fermi coordinate transformation under base-line change in relativistic celestial mechanics" Int. J. Theor. Phys. , 30 (1991) pp. 1097–1116 |
| [a3] | H.S. Ruse, "Taylor's theorem in the tensor calculus" Proc. London Math. Soc. , 32 (1931) pp. 87 |
| [a4] | J.L. Synge, "Relativity: the general theory" , North-Holland (1960) |
Synge world function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Synge_world_function&oldid=18235