Convex surface
A domain (a connected open set) on the boundary of a convex body in the Euclidean space 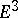 . The entire boundary of a convex body is called a complete convex surface. If the body is finite (bounded), the complete convex surface is called closed. If the body is infinite, the complete convex surface is said to be infinite. An infinite convex surface is homeomorphic to a plane or to a circular cylinder. In the latter case it is itself a cylinder. The simplest kind of convex body is a convex polyhedron, i.e. the intersection of a finite number of half-spaces. The surface of a convex polyhedron is composed of convex polygons and is also called a convex polyhedron.
. The entire boundary of a convex body is called a complete convex surface. If the body is finite (bounded), the complete convex surface is called closed. If the body is infinite, the complete convex surface is said to be infinite. An infinite convex surface is homeomorphic to a plane or to a circular cylinder. In the latter case it is itself a cylinder. The simplest kind of convex body is a convex polyhedron, i.e. the intersection of a finite number of half-spaces. The surface of a convex polyhedron is composed of convex polygons and is also called a convex polyhedron.
The modern theory of convex surfaces was developed chiefly by Soviet geometers, A.D. Aleksandrov and his school. However, individual results of the theory had been known long before. Thus, the rigidity of a closed convex polyhedron was proved by A.L. Cauchy. H. Liebman and W. Blaschke proved that closed convex surfaces are rigid. H. Minkowski proved the existence of a closed convex surface with given Gaussian curvature. H. Weyl outlined the solution of the problem of the existence of a closed convex surface with a given metric. This solution was perfected by H. Lewy. S.E. Cohn-Vossen proved that regular closed convex surfaces are uniquely definable.
To each point 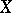 of a convex surface
of a convex surface  naturally corresponds a cone
naturally corresponds a cone 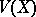 — the limit of the surfaces
— the limit of the surfaces 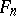 , as
, as 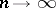 , obtained by a homothety transformation from
, obtained by a homothety transformation from 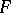 with respect to the point
with respect to the point 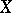 with homothety coefficient
with homothety coefficient  . This cone is called the tangent cone. Depending on the form of the tangent cone, the points of a convex surface are subdivided into conical, ridge and smooth points. A point of a convex surface is called conical if the tangent cone at this point is not degenerate. If, on the other hand, the tangent cone degenerates to a bihedral angle or a plane, the point is called a ridge point or a smooth point, respectively. Non-smooth points on a convex surface are, in a certain sense, an exception. In fact, the set of ridge points has measure zero, while the set of conical points is at most countable.
. This cone is called the tangent cone. Depending on the form of the tangent cone, the points of a convex surface are subdivided into conical, ridge and smooth points. A point of a convex surface is called conical if the tangent cone at this point is not degenerate. If, on the other hand, the tangent cone degenerates to a bihedral angle or a plane, the point is called a ridge point or a smooth point, respectively. Non-smooth points on a convex surface are, in a certain sense, an exception. In fact, the set of ridge points has measure zero, while the set of conical points is at most countable.
The concept of convergence of a series of convex surfaces is defined as follows: A sequence of convex surfaces 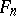 converges to a convex surface
converges to a convex surface 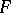 if any open set
if any open set 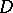 intersects or does not intersect at the same time
intersects or does not intersect at the same time 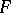 and all
and all  if
if 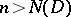 . Any convex surface can be represented as a limit of convex polyhedra. Infinite sets of convex surfaces display the important property of compactness, viz. out of any sequence of complete convex surfaces which do not tend to infinity it is always possible to extract a convergent subsequence with as limit a convex surface, which may degenerate (into a twice-covered plane domain, into a straight line, into a half-line, or into a segment).
. Any convex surface can be represented as a limit of convex polyhedra. Infinite sets of convex surfaces display the important property of compactness, viz. out of any sequence of complete convex surfaces which do not tend to infinity it is always possible to extract a convergent subsequence with as limit a convex surface, which may degenerate (into a twice-covered plane domain, into a straight line, into a half-line, or into a segment).
Any two points of a convex surface can be connected by a rectifiable curve on the surface. The greatest lower bound of the lengths of the curves connecting two given points on a convex surface is said to be the distance between these points on the surface. A curve on a convex surface is called a shortest curve if its length is the smallest of that of the curves on the surface connecting its ends. Any point of a convex surface has a neighbourhood any two points of which can be connected by a shortest curve on the surface. Any two points on a complete convex surface are connected by a shortest curve. A shortest curve on a convex surface has a right and a left semi-tangent at each point. An important property of shortest curves on a convex surface is the non-overlapping property. This means that two shortest curves can be positioned with respect to one another in the following ways only: they have no common points; they have one common point; they have two common points and these points constitute their ends; one shortest curve constitutes a part of the other; or they coincide along a segment, one end of this segment being an end of one shortest curve while the other end is an end of the second. The metric of a convex surface displays the convexity property (cf. Convex metric). The angle between two shortest curves 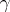 and
and 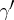 at a point
at a point 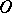 is the limit of the angle
is the limit of the angle  as
as 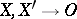 . An angle thus defined exists for any two shortest curves issuing from a common point. Due to the non-overlapping of shortest curves, two shortest curves
. An angle thus defined exists for any two shortest curves issuing from a common point. Due to the non-overlapping of shortest curves, two shortest curves 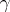 and
and 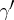 issuing from one point
issuing from one point  subdivide a neighbourhood of this point into two sectors. Let
subdivide a neighbourhood of this point into two sectors. Let 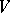 be one of these sectors bounded by the shortest curves
be one of these sectors bounded by the shortest curves 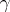 and
and  . Number in this sector the shortest curves
. Number in this sector the shortest curves 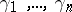 , in the sequence of their occurrence on moving from
, in the sequence of their occurrence on moving from 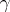 to
to 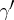 . Let
. Let  be the angles enclosed by neighbouring shortest curves
be the angles enclosed by neighbouring shortest curves 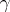 and
and  ,
, 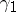 and
and 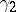 , etc. The angle of the sector
, etc. The angle of the sector 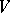 is the least upper bound of the sum of the angles
is the least upper bound of the sum of the angles 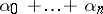 over all shortest curves
over all shortest curves 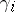 inside the sector. The angle of the sector is equal to the angle between the semi-tangents and the shortest curves at the point
inside the sector. The angle of the sector is equal to the angle between the semi-tangents and the shortest curves at the point 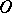 on the evolvent of the tangent cone. The sum of the angles of mutually complementary sectors with apex at the point
on the evolvent of the tangent cone. The sum of the angles of mutually complementary sectors with apex at the point 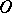 does not depend on the specific shortest curves taken and is called the complete angle at the point
does not depend on the specific shortest curves taken and is called the complete angle at the point 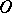 . No complete angle at any point of a convex surface is larger than
. No complete angle at any point of a convex surface is larger than 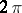 .
.
The concepts of internal (intrinsic) and external (extrinsic) curvature are defined for a convex surface. The internal curvature  is first defined for basic sets — points, open shortest curves and triangles. A triangle is a domain homeomorphic to a circle and bounded by three shortest curves. If
is first defined for basic sets — points, open shortest curves and triangles. A triangle is a domain homeomorphic to a circle and bounded by three shortest curves. If 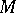 is a point and
is a point and 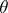 is the complete angle around this point on the surface,
is the complete angle around this point on the surface, 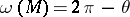 . If
. If 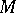 is an open shortest curve, i.e. a shortest curve with excluded ends,
is an open shortest curve, i.e. a shortest curve with excluded ends, 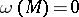 . If
. If 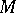 is an open triangle, i.e. a triangle with excluded sides and vertices,
is an open triangle, i.e. a triangle with excluded sides and vertices, 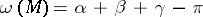 , where
, where  are the angles of the triangle. The curvature is further defined for elementary sets, representable in the form of a set-theoretic sum (union) of pairwise non-intersecting basic sets
are the angles of the triangle. The curvature is further defined for elementary sets, representable in the form of a set-theoretic sum (union) of pairwise non-intersecting basic sets 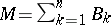 . For such sets
. For such sets 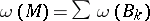 . The internal curvature of any closed set is defined as the greatest lower bound of the internal curvatures of the elementary sets containing the closed set. Finally, the internal curvature of any set is defined as the least upper bound of the internal curvatures of the closed sets contained in it. The internal curvature thus defined on a convex surface is a totally-additive function on the ring of Borel sets (cf. Borel set). The external curvature of a set on a convex surface is defined as the area (Lebesgue measure) under the spherical map of this set. It is defined for all Borel sets on a convex surface and is identical with the internal curvature.
. The internal curvature of any closed set is defined as the greatest lower bound of the internal curvatures of the elementary sets containing the closed set. Finally, the internal curvature of any set is defined as the least upper bound of the internal curvatures of the closed sets contained in it. The internal curvature thus defined on a convex surface is a totally-additive function on the ring of Borel sets (cf. Borel set). The external curvature of a set on a convex surface is defined as the area (Lebesgue measure) under the spherical map of this set. It is defined for all Borel sets on a convex surface and is identical with the internal curvature.
A metric  on a two-dimensional manifold is called internal if the distance
on a two-dimensional manifold is called internal if the distance  between any two points
between any two points 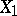 and
and 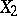 of the manifold is equal to the greatest lower bound of the lengths of the curves on this manifold connecting
of the manifold is equal to the greatest lower bound of the lengths of the curves on this manifold connecting  and
and 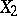 . The length of the curve
. The length of the curve 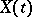 ,
, 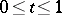 , connecting the points
, connecting the points 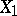 and
and 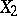 is defined as the least upper bound of the sums
is defined as the least upper bound of the sums
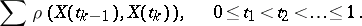 |
Let  and
and 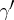 be two curves issuing from a point
be two curves issuing from a point 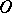 on a manifold with an internal metric. Take points
on a manifold with an internal metric. Take points  and
and 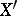 on these curves and construct the plane triangle with sides
on these curves and construct the plane triangle with sides 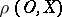 ,
, 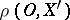 ,
, 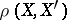 . The lower limit of the angle
. The lower limit of the angle 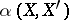 of this triangle opposite to the side
of this triangle opposite to the side 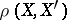 is called the angle between the curves
is called the angle between the curves  and
and 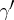 at
at 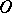 . Clearly, this angle always exists. A metric on a manifold is called convex if the sum of the angles of any triangle whose sides are shortest curves is not less than
. Clearly, this angle always exists. A metric on a manifold is called convex if the sum of the angles of any triangle whose sides are shortest curves is not less than 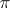 . The metric of a convex surface is convex in this sense. One of the principal results in the theory of convex surfaces is the theorem on the realizability of an internal convex metric on some convex surface. Thus, a complete manifold with an internal convex metric is realized by a complete convex surface.
. The metric of a convex surface is convex in this sense. One of the principal results in the theory of convex surfaces is the theorem on the realizability of an internal convex metric on some convex surface. Thus, a complete manifold with an internal convex metric is realized by a complete convex surface.
The concepts of right and left rotations, which generalize the concept of the integral geodesic curvature, has been introduced for curves on a convex surface. Let 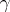 be an arbitrary curve without self-intersections on a convex surface, with ends
be an arbitrary curve without self-intersections on a convex surface, with ends 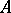 and
and  . A direction on
. A direction on  is specified and a sequence of simple geodesic polygonal curves with ends
is specified and a sequence of simple geodesic polygonal curves with ends  and
and 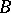 , converging to
, converging to  and situated in the right semi-neighbourhood of the curve, is constructed. Let
and situated in the right semi-neighbourhood of the curve, is constructed. Let 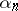 be the angles of the sectors formed by the links of the polygonal curve on the side of the domain bounded by the
be the angles of the sectors formed by the links of the polygonal curve on the side of the domain bounded by the 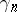 and by
and by 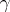 ; let
; let 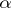 and
and  be the angles of the sectors formed by the polygonal curve
be the angles of the sectors formed by the polygonal curve 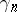 and
and 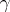 at the end points. The limit of
at the end points. The limit of 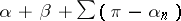 as
as 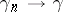 is called the right rotation. This limit always exists if the curve
is called the right rotation. This limit always exists if the curve 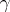 has definite directions at the ends, i.e. semi-tangents, and is independent of the sequence in which the polygonal curves were taken. The left rotation is defined in a similar manner. The rotation of a closed curve is defined by approximating it by a closed polygonal curve on the appropriate side. A generalization of the Gauss–Bonnet theorem applies to convex surfaces. In fact, if a closed curve on a convex surface is the boundary of a domain homeomorphic to a disc, the sum of the curvature of the domain and the rotation of the curve bounding the domain on the side of this domain is
has definite directions at the ends, i.e. semi-tangents, and is independent of the sequence in which the polygonal curves were taken. The left rotation is defined in a similar manner. The rotation of a closed curve is defined by approximating it by a closed polygonal curve on the appropriate side. A generalization of the Gauss–Bonnet theorem applies to convex surfaces. In fact, if a closed curve on a convex surface is the boundary of a domain homeomorphic to a disc, the sum of the curvature of the domain and the rotation of the curve bounding the domain on the side of this domain is 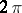 .
.
An isometric transformation is a deformation of a convex surface during which the surface remains convex and its metric remains unchanged, i.e. distances between points on the surface remain unchanged. An isometric transformation is called trivial if it is reduced to a Euclidean motion of the surface as a whole or to a motion and a mirror reflection. A surface without non-trivial isometric transformations is said to be uniquely defined. Closed convex surfaces and infinite convex surfaces with complete curvature  are uniquely defineable. Infinite convex surfaces with complete curvature smaller than
are uniquely defineable. Infinite convex surfaces with complete curvature smaller than 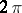 may undergo rather arbitrary non-trivial isometric transformations. All convex surfaces have non-trivial local isometric transformations, i.e. each point on a convex surface has a neighbourhood allowing such transformations.
may undergo rather arbitrary non-trivial isometric transformations. All convex surfaces have non-trivial local isometric transformations, i.e. each point on a convex surface has a neighbourhood allowing such transformations.
The most important tool in studying isometric transformations of convex surfaces is the glueing theorem. According to this theorem, a complete manifold 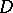 consisting of domains
consisting of domains  that are isometric to a convex surface is itself isometric to a convex surface if the following conditions are met: The boundaries of the domains
that are isometric to a convex surface is itself isometric to a convex surface if the following conditions are met: The boundaries of the domains 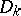 have rotations of bounded variation; the segments of the boundary with equal lengths are identified; the sum of the rotations on any of the segments of identified boundaries is non-negative; and the sum of the sector angles at any common point of the boundaries of domains
have rotations of bounded variation; the segments of the boundary with equal lengths are identified; the sum of the rotations on any of the segments of identified boundaries is non-negative; and the sum of the sector angles at any common point of the boundaries of domains 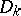 does not exceed
does not exceed 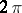 . Theorems on the possibility of non-trivial isometric transformation of a convex surface are usually obtained by "glueing" a planar domain to a convex surface, the above conditions being observed.
. Theorems on the possibility of non-trivial isometric transformation of a convex surface are usually obtained by "glueing" a planar domain to a convex surface, the above conditions being observed.
For general convex surfaces, the concept of an area is introduced for any Borel set; it is introduced at first for the simple sets bounded by shortest curves — geodesic polygons. The polygon is subjected to a fine triangulation  so that the sides of the triangles are smaller than
so that the sides of the triangles are smaller than 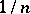 . For each triangle of this triangulation one constructs a planar triangle with sides of the same lengths and takes the sum
. For each triangle of this triangulation one constructs a planar triangle with sides of the same lengths and takes the sum  of the areas of such triangles. It is found that, as
of the areas of such triangles. It is found that, as 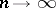 , the sum
, the sum 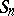 tends to a certain limit, irrespective of the manner in which the polygon has been triangulated. This limit is taken as the area of the polygon. In the following step, the areas of closed, open and general Borel sets are determined using the tools of measure theory. The area of a convex surface is a totally-additive function on the ring of Borel sets.
tends to a certain limit, irrespective of the manner in which the polygon has been triangulated. This limit is taken as the area of the polygon. In the following step, the areas of closed, open and general Borel sets are determined using the tools of measure theory. The area of a convex surface is a totally-additive function on the ring of Borel sets.
The specific curvature of a convex surface in a domain 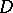 is the ratio of the curvature of the domain to its area. If the specific curvature of a convex surface over all domains lies between positive bounds, the surface is smooth and strictly convex. The Gaussian curvature of a convex surface at a given point
is the ratio of the curvature of the domain to its area. If the specific curvature of a convex surface over all domains lies between positive bounds, the surface is smooth and strictly convex. The Gaussian curvature of a convex surface at a given point 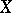 is the limit of the specific curvatures of domains
is the limit of the specific curvatures of domains 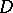 as they contract towards
as they contract towards 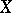 . The Gaussian curvature, if it exists, is a continuous function of the points on the surface. If a certain Gaussian curvature exists on a convex surface, it is possible to introduce polar geodesic coordinates on this surface and to represent the line element of the surface in the form
. The Gaussian curvature, if it exists, is a continuous function of the points on the surface. If a certain Gaussian curvature exists on a convex surface, it is possible to introduce polar geodesic coordinates on this surface and to represent the line element of the surface in the form
 |
The coefficient  is used to determine the Gaussian curvature by the formula:
is used to determine the Gaussian curvature by the formula:
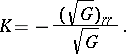 |
A convex surface is called regular if in a neighbourhood of each of its points it can be given by an analytic expression 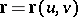 , where
, where 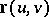 is a regular (i.e. a sufficient number of times differentiable) vector-function satisfying the condition
is a regular (i.e. a sufficient number of times differentiable) vector-function satisfying the condition 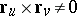 . A metric of a convex surface is called regular if it can be specified using a line element
. A metric of a convex surface is called regular if it can be specified using a line element
 |
and if the coefficients of the form 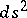 are regular functions. A regular convex surface clearly has a regular metric, since
are regular functions. A regular convex surface clearly has a regular metric, since
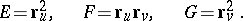 |
The converse proposition is usually false. For instance, a dihedral angle has a regular and even an analytic metric, since it is isometric to a plane, but is not a regular surface. However, if the metric of a convex surface is regular and its Gaussian curvature is positive, the surface is regular. In fact, if the coefficients of the line element are  times differentiable (
times differentiable (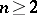 ), the surface is at least
), the surface is at least 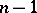 times differentiable.
times differentiable.
The theory of convex surfaces can also be constructed in spaces of constant curvature. As in the case of Euclidean space, a convex surface is a domain in the boundary of a convex body. Many results of the theory of convex surfaces in spaces of constant curvature are formulated and demonstrated as in the case of Euclidean spaces. However, there may also be essential differences between the respective results. Thus, in the Lobachevskii space, a complete convex surface can be homeomorphic to any connected open set on a sphere.
References
| [1] | H. Minkowski, "Volumen und Oberfläche" Math. Ann. , 57 (1903) pp. 447–495 |
| [2] | H. Weyl, "Ueber die Bestimmung einer geschlossenen konvexen Fläche durch ihr Linienelement" Vierteljahrschrift Naturforsch. Gesell. Zurich , 3 : 2 (1916) pp. 40–72 |
| [3] | S.E. Cohn-Vossen, Uspekhi Mat. Nauk , 1 (1936) pp. 33–76 |
| [4] | A.D. Aleksandrov, "Die innere Geometrie der konvexen Flächen" , Akademie Verlag (1955) (Translated from Russian) |
| [5] | A.V. Pogorelov, "Extrinsic geometry of convex surfaces" , Amer. Math. Soc. (1973) (Translated from Russian) |
Comments
Let 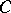 be a convex body in
be a convex body in  . A supporting hyperplane at a point
. A supporting hyperplane at a point  in the boundary of
in the boundary of 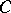 is a plane through this point which contains no interior points of
is a plane through this point which contains no interior points of  . Let
. Let 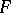 be a convex surface bounding
be a convex surface bounding  . Let
. Let 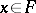 ; for each supporting plane at
; for each supporting plane at  to
to 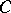 consider the half-space not intersecting the interior of
consider the half-space not intersecting the interior of  (the supporting half-space). The intersection of all supporting half-spaces at
(the supporting half-space). The intersection of all supporting half-spaces at  is a closed cone. The boundary of this cone is the tangent cone at
is a closed cone. The boundary of this cone is the tangent cone at  .
.
For the notion of rigidity of a convex surface see Deformation and Infinitesimal deformation. The Cohn-Vossen theorem says that if 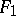 is a closed three times continuously-differentiable surface with positive Gaussian curvature, isometric to another such surface
is a closed three times continuously-differentiable surface with positive Gaussian curvature, isometric to another such surface 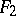 , then
, then 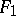 is isometric to
is isometric to 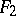 or to its mirror image, where congruence of course means that the one is obtained from the other by a Euclidean motion in the ambient space
or to its mirror image, where congruence of course means that the one is obtained from the other by a Euclidean motion in the ambient space 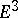 , cf. [5], p. 120.
, cf. [5], p. 120.
A convex surface 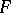 is said to be monotypic in a class
is said to be monotypic in a class 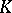 of convex surfaces if any surface in
of convex surfaces if any surface in 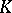 isometric to
isometric to 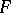 is congruent to
is congruent to 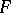 . Thus, one has, e.g., the monotypy theorem that two isometric closed convex polyhedra are congruent. Also, any convex surface
. Thus, one has, e.g., the monotypy theorem that two isometric closed convex polyhedra are congruent. Also, any convex surface  (not necessarily a polyhedron) isometric to a closed convex polyhedron is in fact congruent to that polyhedron (S.P. Olovyanishnikov, 1941).
(not necessarily a polyhedron) isometric to a closed convex polyhedron is in fact congruent to that polyhedron (S.P. Olovyanishnikov, 1941).
References
| [a1] | H. Busemann, "Convex surfaces" , Interscience (1958) |
| [a2] | R. Schneider, "Boundary structure and curvature of convex bodies" J. Tölke (ed.) J.M. Wills (ed.) , Contributions to geometry , Birkhäuser (1979) pp. 13–59 |
Convex surface. A.V. Pogorelov (originator), Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Convex_surface&oldid=18007