Wild sphere

A closed manifold in Euclidean three-space obtained by a wild imbedding of the sphere 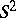in 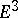. Thus, a wild sphere is the sum of two discs with a common boundary, which is a wild knot. The first example of a wild sphere is the so-called "horned sphere of Alexanderhorned sphere" or Alexander sphere (Fig. a); it bounds a domain which is not homeomorphic to (in the figure this is the interior of the cylinder without any interlinking handles and points forming their boundary). Fig. b shows a wild sphere in which the exterior domain alone is not homeomorphic to .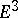

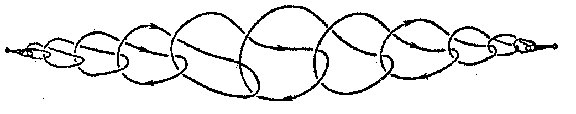
Figure: w097990a

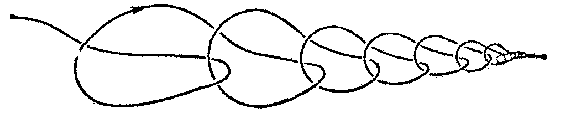
Figure: w097990b

References

[1]	L.V. Keldysh, "Topological imbeddings in Euclidean space" Proc. Steklov Inst. Math. , 81 (1968) Trudy Mat. Inst. Akad. Nauk. SSSR , 81 (1966)

References

[a1]	R.H. Bing, "The geometric topology of -manifolds" , Amer. Math. Soc. (1983)
[a2]	E.E. Moise, "Geometric topology in dimensions 2 and 3" , Springer (1977)