Evolute (surface)
focal surface
The set of cuspidal edges of the developable surfaces formed by the normals to a given surface 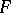 along a family of curvature lines of
along a family of curvature lines of 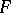 . The evolute consists of two sheets
. The evolute consists of two sheets 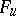 and
and 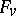 , each of which is the set of centres of normal curvature of the corresponding family
, each of which is the set of centres of normal curvature of the corresponding family  or
or  of curvature lines. The surface itself is called the evolvent (the evolvent surface) of its evolute. For example, the evolute of a torus is its axis of revolution and the circle described by the centre of its rotating circle.
of curvature lines. The surface itself is called the evolvent (the evolvent surface) of its evolute. For example, the evolute of a torus is its axis of revolution and the circle described by the centre of its rotating circle.
The radius vectors 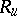 and
and 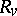 of
of 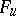 and
and 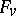 , respectively, are
, respectively, are
 |
where 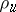 and
and 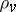 are the radii of normal curvature of the curvature lines of
are the radii of normal curvature of the curvature lines of  and
and  ,
,  is the radius vector of the surface
is the radius vector of the surface 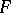 , and
, and  is the unit normal to
is the unit normal to 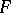 .
.
The envelope of the planes that are parallel to the tangent planes to a given surface 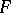 and pass through the middle of the segment between the centres of normal curvature of the curvature lines is called the mean evolute (mean enveloping surface)
and pass through the middle of the segment between the centres of normal curvature of the curvature lines is called the mean evolute (mean enveloping surface) 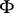 . Its radius vector is
. Its radius vector is 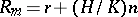 , where
, where 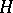 and
and 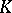 are the mean and Gaussian curvatures of
are the mean and Gaussian curvatures of 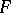 , respectively; consequently,
, respectively; consequently,  and
and 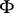 are parallel surfaces. In addition, if
are parallel surfaces. In addition, if 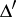 is the Laplace operator corresponding to the third fundamental form of
is the Laplace operator corresponding to the third fundamental form of  and
and  , then
, then  . If
. If 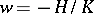 , that is, if the mean evolute degenerates to a plane, then
, that is, if the mean evolute degenerates to a plane, then 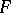 is called a Bonnet surface; if
is called a Bonnet surface; if 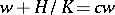 , then
, then 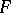 is homothetic to
is homothetic to  and is called a Goursat surface. In particular, for
and is called a Goursat surface. In particular, for 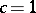 one obtains a minimal surface.
one obtains a minimal surface.
Comments
Both leaves of the evolute of a surface can be obtained as the set of critical values of the end-point mapping, defined on the normal bundle of the surface and assigning to the normal vector  at
at  the value
the value  in space. This can be easily generalized to define focal sets or evolute submanifolds in higher dimensions and codimensions.
in space. This can be easily generalized to define focal sets or evolute submanifolds in higher dimensions and codimensions.
References
| [a1] | G. Darboux, "Leçons sur la théorie générale des surfaces et ses applications géométriques du calcul infinitésimal" , 1 , Gauthier-Villars (1887) pp. 1–18 |
| [a2] | M. Berger, B. Gostiaux, "Differential geometry: manifolds, curves, and surfaces" , Springer (1988) (Translated from French) |
Evolute (surface). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Evolute_(surface)&oldid=16367