Category cohomology
Let  be a small category,
be a small category,  an Abelian category with exact infinite products, and
an Abelian category with exact infinite products, and  a covariant functor. Define the objects
a covariant functor. Define the objects  for
for  in the following way:
in the following way:
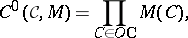 |
 |
where  is a sequence of morphisms of
is a sequence of morphisms of  with
with 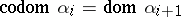 ,
,  . Let
. Let
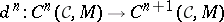 |
be the monomorphism induced by the family of morphisms
 |
and by the family
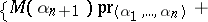 |
 |
 |
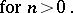 |
Here, 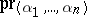 denotes the projection
denotes the projection 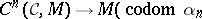 ).
).
The morphisms 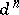 ,
, 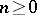 satisfy the conditions
satisfy the conditions 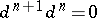 , and therefore one obtains a complex
, and therefore one obtains a complex  in
in  . The homology objects of this complex are called the cohomology of
. The homology objects of this complex are called the cohomology of  with coefficients in
with coefficients in 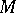 and are denoted by
and are denoted by 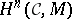 . For any functor
. For any functor 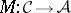 there are a functor
there are a functor 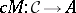 (called the co-induced functor) and a monomorphism
(called the co-induced functor) and a monomorphism  such that
such that 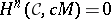 for
for 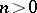 .
.
The functor 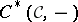 is an exact functor. Therefore, any short exact sequence of functors induces a long exact sequence of cohomology objects of
is an exact functor. Therefore, any short exact sequence of functors induces a long exact sequence of cohomology objects of 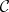 . It can be proved that the functors
. It can be proved that the functors 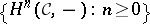 form a universal connected (exact) sequence of functors and that
form a universal connected (exact) sequence of functors and that
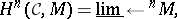 |
where 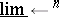 is
is  th right satellite of the functor
th right satellite of the functor  (here,
(here, 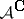 denotes the category of functors from
denotes the category of functors from  to
to 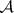 , and
, and  is an (inverse) limit functor).
is an (inverse) limit functor).
For a small category 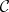 , let
, let  denote the pre-additive category whose objects are those of
denote the pre-additive category whose objects are those of 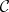 and
and 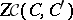 is the free Abelian group on
is the free Abelian group on 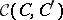 (cf. also Free group). Composition is defined in the unique way so as to be bilinear and to make the inclusion
(cf. also Free group). Composition is defined in the unique way so as to be bilinear and to make the inclusion 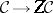 a functor. If
a functor. If 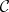 is a monoid, then
is a monoid, then 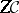 is the monoid ring of
is the monoid ring of 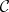 with coefficients in
with coefficients in 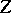 . The inclusion
. The inclusion 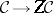 induces an isomorphism of categories
induces an isomorphism of categories
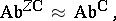 |
where the left-side is the category of additive functors from 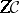 to
to 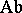 (the category of Abelian groups) and the right-hand side side is the category of all functors from
(the category of Abelian groups) and the right-hand side side is the category of all functors from 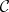 to
to 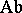 .
.
If  is the category of Abelian groups (
is the category of Abelian groups ( ), one has
), one has
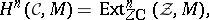 |
where  denotes the constant functor
denotes the constant functor 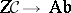 (i.e.
(i.e. 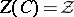 ,
, 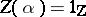 for any object
for any object 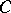 and any morphism
and any morphism  of
of  ), and
), and 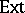 is taken in the category of additive functors
is taken in the category of additive functors 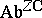 .
.
If 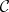 is a group (i.e. a category with one object and whose morphisms are invertible) and
is a group (i.e. a category with one object and whose morphisms are invertible) and  , then the groups
, then the groups 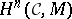 are the cohomology groups (cf. also Cohomology group) of the group
are the cohomology groups (cf. also Cohomology group) of the group  with coefficients in
with coefficients in 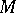 , which is a module over the group ring
, which is a module over the group ring 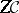 (cf. also Cross product). In this case the co-induced functor
(cf. also Cross product). In this case the co-induced functor  is a co-induced
is a co-induced 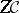 -module
-module 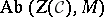 .
.
As for the case of groups,  -fold extensions of categories can be defined and the isomorphism with
-fold extensions of categories can be defined and the isomorphism with  cohomologies of categories can be established. Under additional assumptions, the properties of group cohomologies are obtained for category cohomologies (e.g. the universal coefficient formula for the cohomology group of a category, etc.).
cohomologies of categories can be established. Under additional assumptions, the properties of group cohomologies are obtained for category cohomologies (e.g. the universal coefficient formula for the cohomology group of a category, etc.).
For a commutative ring  , a
, a 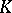 -category is a category
-category is a category 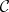 equipped with a
equipped with a  -module structure on each hom-set in such a way that composition induces a
-module structure on each hom-set in such a way that composition induces a 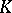 -module homomorphism. If
-module homomorphism. If 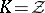 , a
, a  -category is just a pre-additive category. B. Mitchell has defined the (Hochshild) cohomology group of a small
-category is just a pre-additive category. B. Mitchell has defined the (Hochshild) cohomology group of a small 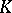 -category
-category 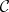 with coefficients in a bimodule (i.e., bifunctor)
with coefficients in a bimodule (i.e., bifunctor) 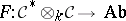 (where
(where 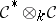 denotes the tensor product of categories). H.-J. Baues and G. Wirshing have introduced cohomology of a small category with coefficients in a natural system, which generalizes known concepts and uses Abelian-group-valued functors (i.e. modules) and bifunctors as coefficients.
denotes the tensor product of categories). H.-J. Baues and G. Wirshing have introduced cohomology of a small category with coefficients in a natural system, which generalizes known concepts and uses Abelian-group-valued functors (i.e. modules) and bifunctors as coefficients.
References
| [a1] | H.-J. Baues, G. Wirshing, "Cohomology of small categories" J. Pure Appl. Algebra , 38 (1985) pp. 187–211 |
| [a2] | T. Datuashvili, "The cohomology of categories" Tr. Tbiliss. Mat. Inst. A. Razmadze, Akad. Nauk Gruzin.SSR , 62 (1979) pp. 28–37 |
| [a3] | G. Hoff, "On the cohomology of categories" Rend. Math. (VI) , 7 : 2 (1974) pp. 169–192 |
| [a4] | M.J. Lee, "A generalized Mayer–Vietoris sequence" Math. Jap. , 19 : 1 (1974) pp. 41–50 |
| [a5] | B. Mitchell, "Rings with several objects" Adv. Math. , 8 (1972) pp. 1–161 |
| [a6] | D.G. Quillen, "Higher algebraic 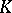 -theories" H. Bass (ed.) , Algebraic -theories" H. Bass (ed.) , Algebraic 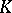 -theory I , Lecture Notes Math. , 341 , Springer (1973) pp. 85–147 -theory I , Lecture Notes Math. , 341 , Springer (1973) pp. 85–147 |
| [a7] | J.-E. Roos, "Sur les foncteurs derives de  . Applications" C.R. Acad. Sci. Paris , 252 (1961) pp. 3702–3704 . Applications" C.R. Acad. Sci. Paris , 252 (1961) pp. 3702–3704 |
| [a8] | Ch.E. Watts, "A homology theory for small categories" , Proc. Conf. Categorical Algebra (La Jolla, Calif., 1965) , Springer (1966) pp. 331–335 |
Category cohomology. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Category_cohomology&oldid=50499