Quasi-triangular Hopf algebra

dual quasi-triangular Hopf algebra, co-quasi-triangular Hopf algebra, quantum group

A quantum group in the strict sense, i.e. a Hopf algebra 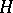equipped with a further (co-) quasi-triangular structure 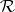obeying certain axioms such that the category of (co-) modules of 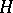is a braided category (cf. also Quantum groups). This is arguably the key property behind the quantum group enveloping algebras 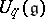or their associated quantum group coordinate rings .

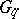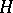More precisely, a quasi-triangular Hopf algebra is where is a Hopf algebra over a fiel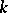d and 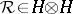obeys

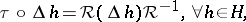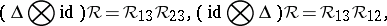where is the permutation operation on and 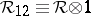, 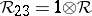are in 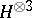in the latter equations. One may show that then obeys

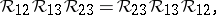which is an abstract form of the Yang–Baxter equation. One denotes the Hopf algebra structure by 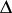for the co-product, and denotes by the co-unit and by 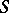the antipode.

Examples.

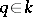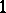1) When is an th root of , the quantum group is given by the polynomial algebra 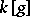modulo 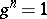(the group ring of 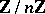) with co-algebra, antipode and quasi-triangular structure

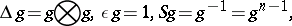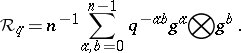It is assumed that is invertible in .

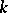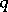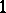2) When is a primitive th root of , the finite-dimensional quantum group 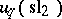is the free associative algebra 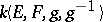modulo the relations

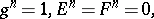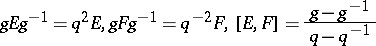and the co-algebra, antipode and quasi-triangular structure

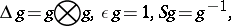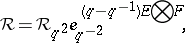where

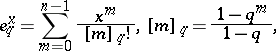is the 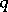-exponential with 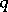-integers 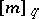in the factorial.

For general 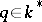(an invertible element of 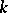), one has the infinite-dimensional Hopf algebra 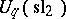, where the 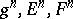relations above are omitted. In this case 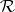has to be described via some form of completion. One formulation is to work over the ring of formal power series rather than over a field as above. If it is assumed that and 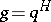, one can reformulate 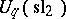with 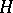as a generator and define 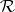with 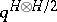in place of 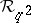above (and now take an infinite sum in the exponential). It does not, however, live in the algebraic tensor product but in a completion of it. On the other hand, this formulation allows one to consider the structure to lowest order in 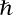. This is the Lie algebra , the Lie co-bracket (forming a Lie bi-algebra) and a Lie quasi-triangular structure 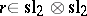obeying the classical Yang–Baxter equations. It extends to a Poisson bracket (cf. Poisson brackets) on the group 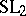, making it a Poisson Lie group. This means a Poisson bracket on the group such that the product mapping is a Poisson mapping from the direct product Poisson structure. There are similar quantum group enveloping algebras 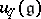and 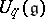for all complex semi-simple Lie algebras .

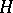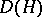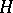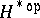3) Every finite-dimensional Hopf algebra with invertible antipode can be "doubled" to obtain a quasi-triangular Hopf algebra , called the quantum double of . This contains and (the dual of 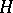with reversed product) as sub-Hopf algebras and the additional cross relations

for all 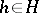and 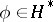, where 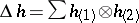is a notation for the co-product of 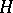, etc., and 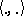denotes the evaluation pairing. The quasi-triangular structure is

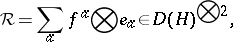where 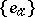is a basis of 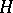and is a dual basis. can in fact be built explicitly on the vector space 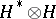as a double cross product 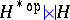. The braided category of 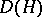-modules can be identified with that of crossed 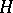-modules by viewing a co-action of 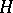as an action of 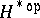by evaluation.

Dually, i.e. reversing all arrows, one has the notion of a dual quasi-triangular or co-quasi-triangular Hopf algebra 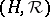where 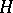is a Hopf algebra and obeys

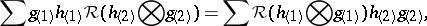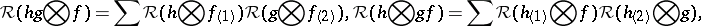for 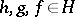. One also requires 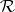to be convolution-invertible in the sense

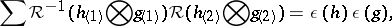for .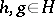

A) Let 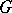be an Abelian group equipped with a bi-character (a function multiplicative in each input). The group algebra 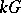is the vector space with basis and with product among basis elements the group product. The co-product 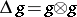and co-unit 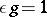extended linearly make 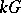into a Hopf algebra. The bi-character makes this dual quasi-triangular, with 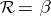on basis elements, extended linearly.

B) The dual quasi-triangular Hopf algebra 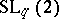is the free associative algebra 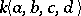modulo the relations

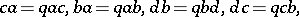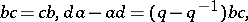and the "q-determinant relation"

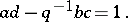The co-algebra has the matrix form

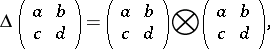(matrix multiplication understood). The antipode is

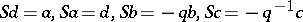and the dual quasi-triangular structure is

on a basis 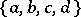of generators. The extension to products is then determined. Here it is assumed that 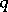has a square root in 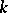. The quantum group 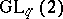is similar, with 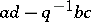inverted rather than set to .

There are similar quantum group coordinate rings 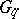for the standard families of simple Lie groups, known explicitly for the non-exceptional families. They are deformations of the classical coordinate rings and in fact quantize the Poisson Lie group structures on 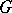corresponding to 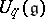. They are dually paired as Hopf algebras with .

C) More generally, given any invertible matrix solution 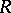of the Yang–Baxter equations, there is a dual-quasi-triangular bi-algebra of quantum matrices 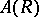as the free associative algebra 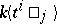(on a matrix of generators) modulo the relations

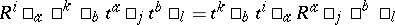(summation of repeated indices). The co-algebra has the matrix form

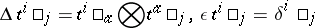(summation over ). The dual-quasi-triangular structure is

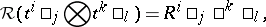extended to products by the quasi-triangularity axioms. Note that 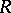need not obey the Yang–Baxter equations in order to have a bi-algebra: 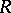provides the dual quasi-triangular structure.

In between these formulations is an intermediate one, called a quasi-triangular dual pair. This is a pair of Hopf algebras 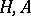, a duality pairing 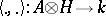between them, and a mapping 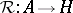obeying certain axioms. In particular, 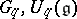are dually paired and there is a suitable mapping 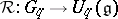. This provides a way of working with quasi-triangular structures that includes quantum group enveloping algebras but avoids formal power series.

References

[a1]	V.G. Drinfel'd, "Quantum groups" A. Gleason (ed.) , Proc. Internat. Math. Congress , Amer. Math. Soc. (1987) pp. 798–820
[a2]	L.D. Faddeev, N.Yu. Reshetikhin, L.A. Takhtajan, "Quantization of Lie groups and Lie algebras" Leningrad Math. J. , 1 (1990) pp. 193–225
[a3]	S. Majid, "Quasitriangular Hopf algebras and Yang-Baxter equations" Internat. J. Modern Physics A , 5 : 1 (1990) pp. 1–91
[a4]	S. Majid, "Foundations of quantum group theory" , Cambridge Univ. Press (1995)