Riedtmann classification

A finite-dimensional algebra 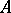over an algebraically closed field 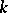is called self-injective if 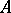, considered as a right 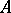-module, is injective (cf. also Injective module). Well-known examples for self-injective algebras are the group algebras 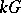for finite groups 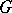(cf. also Group algebra). An arbitrary finite-dimensional algebra 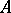is said to be representation-finite provided that there are only finitely many isomorphism classes of indecomposable finite-dimensional right 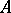-modules.

C. Riedtmann made the main contribution to the classification of all self-injective algebras that are representation-finite. Her key idea was not to look at the algebra 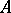itself, but rather at its Auslander–Reiten quiver 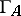. (Quiver is an abbreviation for directed graph, see Quiver.) The vertices of the Auslander–Reiten quiver (see also Representation of an associative algebra) are the isomorphism classes of finite-dimensional 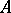-modules. The number of arrows from the isomorphism class of 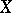to the isomorphism class of 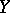is the dimension of the space 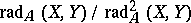, where 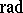is the Jacobson radical of the category of all finite-dimensional 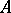-modules. The Auslander–Reiten quiver is a translation quiver, which means that it carries an additional structure, namely a translation mapping the non-projective vertices bijectively to the non-injective vertices. 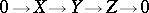The translation is induced by the existence of almost-spit sequences (see also Representation of an associative algebra; Almost-split sequence) and sends the isomorphism class of a non-projective indecomposable module 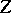to the starting term .

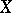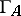The stable part of the Auslander–Reiten quiver of 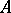is the full subquiver of given by the modules that cannot be shifted into an injective or projective vertex by a power 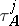for some integer 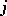. 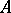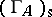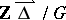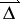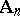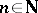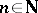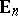In [a3], Riedtmann succeeded to prove that for any connected representation-finite finite-dimensional the stable part of the Auslander–Reiten quiver is of the shape , where is a quiver whose underlying graph is a Dynkin diagram (), (, ), or () and 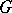is an infinite cyclic group of automorphisms of the translation quiver 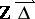. The vertices of are the pairs such that 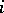is an integer and a vertex of 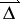. From 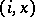to 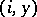there are the arrows 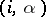with 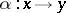an arrow of 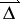. In addition, from 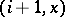to 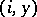there exist the arrows 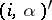with an arrow of 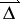. The translation maps 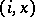to .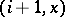

For a self-injective algebra 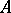, the only vertices of the Auslander–Reiten quiver that do not belong to 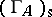are the isomorphism classes of the indecomposable projective (and injective) modules. Thus, one can reconstruct 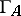from by finding in 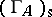the starting points of arrows of 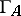ending in projective vertices. These combinatorial data are called a configuration. This shows that for finding all possible Auslander–Reiten quivers 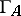of all connected representation-finite self-injective algebras one has to classify the infinite cyclic automorphism groups of 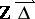and the 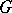-invariant configurations of 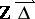for all Dynkin diagrams. For the Dynkin diagrams and 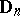this classification was carried out in [a4] and [a5].

The classification of the possible configurations for the exceptional Dynkin diagrams 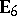, , 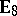turned out to be more difficult. Fortunately, the development of tilting theory offered a convenient way for a solution. Namely, it was observed in [a1] and [a2] that in order to equip 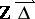with all possible configurations, one has to form the Auslander–Reiten quivers of the repetitive algebras of the tilted algebras of representation-finite hereditary algebras of type 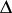(cf. also Tilted algebra). Nevertheless, the full classification of all these repetitive algebras eventually obtained in [a7] required the use of a computer for handling the huge amount of structures appearing in the case .

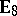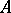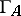If one finally wants to return from the Auslander–Reiten quiver to the algebra itself, one considers the factor of the free -linear category of by the mesh relations induced by the almost-split sequences. This factor is called the mesh category of . Forming the endomorphism algebra of the direct sum of all projective objects in this mesh category yields (up to Morita equivalence), provided that 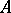is standard (i.e. the mesh category is equivalent to the category of indecomposable finite-dimensional 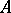-modules). Non-standard algebras appear only if the characteristic of the field is and 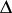is of type . They were classified in [a6] and [a9].

It is worth noting that the approach using repetitive algebras was generalized in order to classify the representation-tame self-injective standard algebras of polynomial growth in [a8]. In this case tilted algebras of representation-tame hereditary and canonical algebras replace the tilted algebras of representation-finite hereditary algebras.

References

[a1]	O. Bretscher, C. Läser, C. Riedtmann, "Selfinjective and simply connected algebras" Manuscripta Math. , 36 (1981/82) pp. 253–307
[a2]	D. Hughes, J. Waschbüsch, "Trivial extensions of tilted algebras" Proc. London Math. Soc. , 46 (1983) pp. 347–364
[a3]	C. Riedtmann, "Algebren, Darstellungen, Überlagerungen und zurück" Comment. Math. Helv. , 55 (1980) pp. 199–224
[a4]	C. Riedtmann, "Representation-finite selfinjective algebras of class " , Representation theory II , Lecture Notes in Mathematics , 832 , Springer (1981) pp. 449–520
[a5]	C. Riedtmann, "Configurations of " J. Algebra , 82 (1983) pp. 309–327
[a6]	C. Riedtmann, "Representation-finite self-injective algebras of class 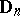" Compositio Math. , 49 (1983) pp. 231–282
[a7]	B. Roggon, "Selfinjective and iterated tilted algebras of type 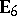, , 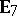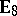" , E 95-008 SFB , 343 , Bielefeld (1995)
[a8]	A. Skowroński, "Selfinjective algebras of polynomial growth" Math. Ann. , 285 (1989) pp. 177–199
[a9]	J. Waschbüsch, "Symmetrische Algebren vom endlichen Modultyp" J. Reine Angew. Math. , 321 (1981) pp. 78–98