Colombeau generalized function algebras

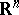Let be an open subset of , and let 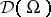be the algebra of compactly supported smooth functions. In the original definition, J.F. Colombeau [a2] started from the space 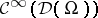of infinitely Silva-differentiable mappings from 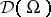into 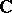. The space of distributions 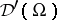is just the subspace of linear mappings 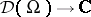. Let

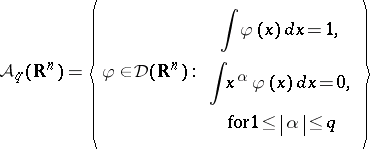and let

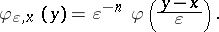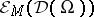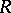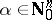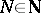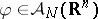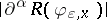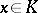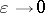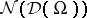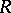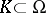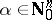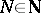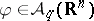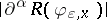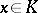The subalgebra is defined by those members such that for all compact subsets and for all multi-indices there is an such that for all , the supremum of over is of order as . The ideal is defined by those members such that for all compact subsets and all there is an such that for all and , the supremum of over is of order 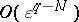as 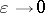. The Colombeau generalized function algebra is the factor algebra . It contains the space of distributions with derivatives faithfully extended (cf. also Generalized function, derivative of a). The asymptotic decay property expressed in 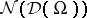together with an argument using Taylor expansion shows that 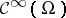is a faithful subalgebra.

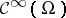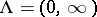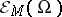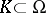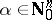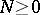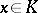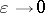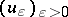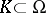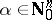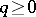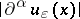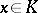Later, Colombeau [a3], [a4] replaced the construction by a reduced power of with index set : Let be the algebra of all nets such that for all compact subsets and all multi-indices there is an such that the supremum of over is of order as (cf. also Net (directed set)). Let be the ideal therein given by those such that for all compact subsets , all and all , the supremum of over is of order 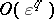as 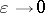. Then set 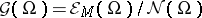. There exist versions with the infinite-order Sobolev space 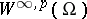in the place of , , 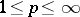or with other topological algebras.

It is possible to enlarge the class of mollifiers (hence the index set in the reduced power construction) to produce a version for which smooth coordinate changes commute with the imbedding of distributions. This way Colombeau generalized functions can be defined intrinsically on manifolds. Generalized stochastic processes with paths in have been introduced as well.

The subalgebra 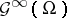is defined by interchanging quantifiers: For all compact sets 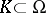there is an such that for all 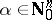, the supremum of 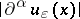on 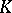is of order as 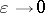. One has that 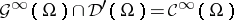, and 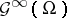plays the same role in regularity theory here as 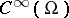does in distribution theory (for example, 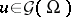and 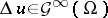implies 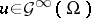, where 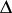denotes the Laplace operator).

For applications in a variety of fields of non-linear analysis and physics, see [a1], [a4], [a5], [a6], [a7].

See also Generalized function algebras.

References

[a1]	H.A. Biagioni, "A nonlinear theory of generalized functions" , Springer (1990)
[a2]	J.F. Colombeau, "New generalized functions and multiplication of distributions" , North-Holland (1984)
[a3]	J.F. Colombeau, "Elementary introduction to new generalized functions" , North-Holland (1985)
[a4]	J.F. Colombeau, "Multiplication of distributions. A tool in mathematics, numerical engineering and theoretical physics" , Springer (1992)
[a5]	"Nonlinear theory of generalized functions" M. Grosser (ed.) G. Hörmann (ed.) M. Kunzinger (ed.) M. Oberguggenberger (ed.) , Chapman and Hall/CRC (1999)
[a6]	M. Nedeljkov, S. Pilipović, D. Scarpalézos, "The linear theory of Colombeau generalized functions" , Longman (1998)
[a7]	M. Oberguggenberger, "Multiplication of distributions and applications to partial differential equations" , Longman (1992)