Generalized function algebras
Let  be an open subset of
be an open subset of  . A generalized function algebra is an associative, commutative differential algebra
. A generalized function algebra is an associative, commutative differential algebra  containing the space of distributions
containing the space of distributions 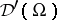 or other distribution spaces as a linear subspace (cf. also Generalized functions, space of). An early construction of a non-associative, non-commutative algebra was given by H. König [a6]. The main current (2000) direction has been to construct associative, commutative algebras as reduced powers
or other distribution spaces as a linear subspace (cf. also Generalized functions, space of). An early construction of a non-associative, non-commutative algebra was given by H. König [a6]. The main current (2000) direction has been to construct associative, commutative algebras as reduced powers  of classical function spaces
of classical function spaces 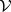 . A further approach uses analytic continuation and asymptotic series of distributions.
. A further approach uses analytic continuation and asymptotic series of distributions.
To describe the principles, consider the space 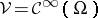 of infinitely differentiable functions on
of infinitely differentiable functions on  (cf. also Differentiable function). Let
(cf. also Differentiable function). Let 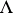 be an infinite index set,
be an infinite index set,  a differential subalgebra of
a differential subalgebra of 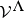 and
and 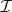 a differential ideal in
a differential ideal in  . The generalized function algebra
. The generalized function algebra 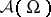 is defined as the factor algebra
is defined as the factor algebra 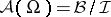 . Assuming that
. Assuming that 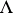 is a directed set, let
is a directed set, let  be a net in
be a net in 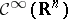 (cf. also Net (directed set)) converging to the Dirac measure in
(cf. also Net (directed set)) converging to the Dirac measure in 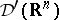 (cf. also Generalized functions, space of). Any compactly supported distribution
(cf. also Generalized functions, space of). Any compactly supported distribution  can be imbedded in
can be imbedded in  by convolution (cf. also Generalized function):
by convolution (cf. also Generalized function): 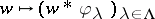 . Appropriate conditions on
. Appropriate conditions on 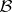 and
and 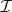 will guarantee that this extends to an imbedding of
will guarantee that this extends to an imbedding of 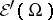 into
into 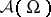 . An imbedding of
. An imbedding of 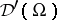 is obtained, provided the family
is obtained, provided the family 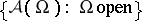 forms a sheaf of differential algebras on
forms a sheaf of differential algebras on  (the restriction mappings are defined componentwise on representatives). This imbedding preserves the derivatives of distributions. It follows from the impossibility result of L. Schwartz (see Multiplication of distributions) that it cannot retain the pointwise product of continuous functions at the same time. If
(the restriction mappings are defined componentwise on representatives). This imbedding preserves the derivatives of distributions. It follows from the impossibility result of L. Schwartz (see Multiplication of distributions) that it cannot retain the pointwise product of continuous functions at the same time. If 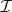 is contained in the subspace
is contained in the subspace 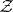 of
of 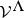 comprised by those nets which converge weakly to zero, then an equivalence relation
comprised by those nets which converge weakly to zero, then an equivalence relation  can be defined on
can be defined on 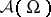 by requiring that
by requiring that 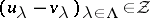 for representatives
for representatives 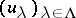 and
and 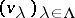 of
of  and
and  . The pointwise product of continuous functions (as well as all products obtained by multiplication of distributions) are retained up to this equivalence relation. A list of typical examples of generalized function algebras follows:
. The pointwise product of continuous functions (as well as all products obtained by multiplication of distributions) are retained up to this equivalence relation. A list of typical examples of generalized function algebras follows:
1) 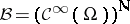 ,
, 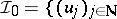 : there is
: there is  such that
such that 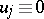 for
for 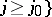 . The algebra
. The algebra  was introduced by C. Schmieden and D. Laugwitz [a10] in their foundations of infinitesimal analysis.
was introduced by C. Schmieden and D. Laugwitz [a10] in their foundations of infinitesimal analysis.
2) Let 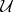 be a free ultrafilter on the infinite set
be a free ultrafilter on the infinite set 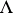 and define
and define 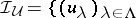 : the set of indices
: the set of indices 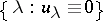 belongs to
belongs to 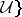 , let
, let 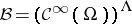 . Then
. Then  is an instance of the ultrapower construction of the algebra of internal smooth functions of non-standard analysis (A. Robinson [a8]).
is an instance of the ultrapower construction of the algebra of internal smooth functions of non-standard analysis (A. Robinson [a8]).
Neither 1) nor 2) provide sheaves on 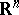 . To get a sheaf, localization must be introduced:
. To get a sheaf, localization must be introduced:
3) Let 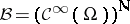 ,
, 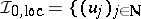 : for each compact subset
: for each compact subset 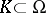 there is a
there is a 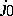 such that
such that 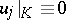 for
for  . The algebra
. The algebra 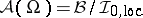 was introduced by Yu.V. Egorov [a3] (cf. also Egorov generalized function algebra).
was introduced by Yu.V. Egorov [a3] (cf. also Egorov generalized function algebra).
4) Let 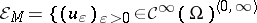 : for each compact subset
: for each compact subset 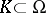 and each multi-index
and each multi-index 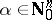 there is an
there is an 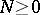 such that the supremum of
such that the supremum of  over
over  is of order
is of order 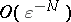 as
as 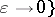 . Let
. Let 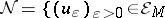 : for each compact subset
: for each compact subset 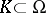 , each multi-index
, each multi-index 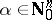 and each
and each  , the supremum of
, the supremum of 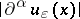 over
over 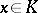 is of order
is of order 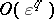 as
as  . Then
. Then 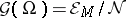 is one of the versions of the algebras of J.F. Colombeau [a1] (cf. also Colombeau generalized function algebras). It is distinguished by the fact that the imbedding of
is one of the versions of the algebras of J.F. Colombeau [a1] (cf. also Colombeau generalized function algebras). It is distinguished by the fact that the imbedding of 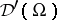 gives
gives 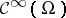 as a faithful subalgebra.
as a faithful subalgebra.
5) Let 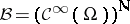 ,
, 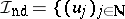 : there is a closed, nowhere-dense subset
: there is a closed, nowhere-dense subset 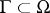 such that for all
such that for all 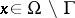 there are a
there are a  and a neighbourhood
and a neighbourhood 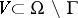 of
of  such that
such that  for
for 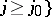 . This is the nowhere dense ideal introduced by E.E. Rosinger [a9] (cf. also Rosinger nowhere-dense generalized function algebra). The algebra
. This is the nowhere dense ideal introduced by E.E. Rosinger [a9] (cf. also Rosinger nowhere-dense generalized function algebra). The algebra  contains the algebra
contains the algebra 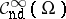 of smooth functions defined off some nowhere-dense set as a subalgebra. Since
of smooth functions defined off some nowhere-dense set as a subalgebra. Since 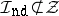 , the imbedding of
, the imbedding of 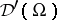 cannot be done by convolution, but uses an algebraic basis.
cannot be done by convolution, but uses an algebraic basis.
There are many variations on this theme, different sets 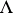 , different spaces
, different spaces 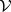 . The algebras can be defined on smooth manifolds as well. Usually, further operations can be applied to the elements of these algebras: superposition with non-linear mappings, restriction to submanifolds, pointwise evaluation (with values in the corresponding ring of constants).
. The algebras can be defined on smooth manifolds as well. Usually, further operations can be applied to the elements of these algebras: superposition with non-linear mappings, restriction to submanifolds, pointwise evaluation (with values in the corresponding ring of constants).
The algebras offer a general framework for studying all problems involving non-linear operations, differentiation, and distributional or otherwise non-smooth data and coefficients. Applications include non-linear partial differential equations, stochastic partial differential equations, Lie symmetry transformations, distributional metrics in general relativity, quantum field theory. For a survey of current applications, see [a4].
A second approach is based on the algebras constructed by V.K. Ivanov [a5] by means of analytic or harmonic regularization of homogeneous distributions and on the weak asymptotic expansions of V.P. Maslov (see e. g. [a7]). A simple, specific example is given by the space 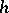 of distributions spanned by
of distributions spanned by 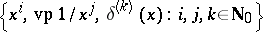 in one dimension, where
in one dimension, where 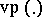 denotes the principal value distribution and
denotes the principal value distribution and 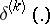 the
the 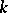 th derivative of the Dirac measure (cf. also Generalized function). Their harmonic regularizations generate a function algebra
th derivative of the Dirac measure (cf. also Generalized function). Their harmonic regularizations generate a function algebra 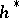 of smooth functions
of smooth functions 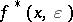 defined on
defined on 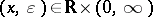 . Each
. Each 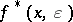 has a unique weak asymptotic expansion of the form
has a unique weak asymptotic expansion of the form 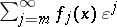 as
as 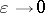 with coefficients
with coefficients  in the original space
in the original space  ; the summation starts at some, possibly negative,
; the summation starts at some, possibly negative, 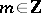 . The approach was extended [a2] to the class of associated homogeneous distributions. This way the structure of an algebra may be introduced on certain subspaces of the space of asymptotic series with distribution coefficients. As an application, asymptotic solutions to non-linear partial differential equations can be constructed by direct computation with the asymptotic series.
. The approach was extended [a2] to the class of associated homogeneous distributions. This way the structure of an algebra may be introduced on certain subspaces of the space of asymptotic series with distribution coefficients. As an application, asymptotic solutions to non-linear partial differential equations can be constructed by direct computation with the asymptotic series.
A relation with the previous construction of generalized function algebras is obtained by observing that harmonic regularization amounts to convolution with the kernel
 |
References
| [a1] | J.F. Colombeau, "New generalized functions and multiplication of distributions" , North-Holland (1984) MR0738781 Zbl 0532.46019 |
| [a2] | V.G. Danilov, V.P. Maslov, V.M. Shelkovich, "Algebras of singularities of singular solutions to first-order quasilinear strictly hyperbolic systems" Theoret. Math. Phys. , 114 : 1 (1998) pp. 3–55 MR1756560 |
| [a3] | Yu.V. Egorov, "A contribution to the theory of generalized functions" Russian Math. Surveys , 45 : 5 (1990) pp. 1–49 Zbl 0754.46034 |
| [a4] | "Nonlinear theory of generalized functions" M. Grosser (ed.) G. Hörmann (ed.) M. Kunzinger (ed.) M. Oberguggenberger (ed.) , Chapman and Hall/CRC (1999) MR1699842 Zbl 0918.00026 |
| [a5] | V.K. Ivanov, "An associative algebra of the simplest generalized functions" Sib. Math. J. , 20 (1980) pp. 509–516 |
| [a6] | H. König, "Multiplikation von Distributionen I" Math. Ann. , 128 (1955) pp. 420–452 MR0068745 Zbl 0064.11303 |
| [a7] | V.P. Maslov, G.A. Omel'yanov, "Asymptotic soliton-form solutions of equations with small dispersion" Russian Math. Surveys , 36 : 3 (1981) pp. 73–149 Zbl 0494.35080 |
| [a8] | A. Robinson, "Non-standard analysis" , North-Holland (1966) MR0205854 Zbl 0151.00803 |
| [a9] | E.E. Rosinger, "Nonlinear partial differential equations. Sequential and weak solutions" , North-Holland (1980) MR0590891 Zbl 0447.35001 |
| [a10] | C. Schmieden, D. Laugwitz, "Eine Erweiterung der Infinitesimalrechnung" Math. Z. , 69 (1958) pp. 1–39 MR0095906 Zbl 0082.04203 |
Generalized function algebras. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Generalized_function_algebras&oldid=49997