Frame

A set of linearly independent vectors taken in a definite order and placed at a common origin. Any three non-parallel vectors not lying in one plane can serve as a frame for the vectors in space. If the vectors building the frame are mutually orthogonal, then the frame is called orthogonal; if in this case the length of the vectors is equal to one, the frame is called orthonormal.

Comments

Usually a frame is called a basis (of vectors in space). In this sense, the word "frame" is also used in physics (frame of reference, cf. Reference system). For Frénet frame see Frénet trihedron.

A framing of an -dimensional differentiable manifold is a vector bundle isomorphism of its tangent bundle 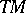with the trivial bundle (so that is parallelizable). Using the standard basis 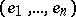of 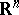such an isomorphism defines a frame field: it assigns to every 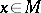a frame, or basis, of the tangent space at that point.

The frame bundle over a manifold 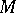is the principal fibre bundle with structure group 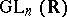whose fibre over 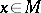is the collection of all bases (frames) of 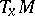, the tangent space at that point.

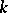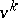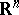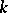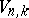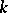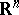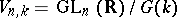A -frame in is an ordered set of linearly independent vectors. Let denote the set of all -frames in . Let be the subgroup of leaving a fixed frame invariant. Then . Thus, has a real-analytic structure. It is called the Stiefel manifold of 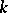-frames in -space.

References

[a1]	N.E. Steenrod, "The topology of fibre bundles" , Princeton Univ. Press (1951)