Compactification
compact extension
An extension of a topological space which is a compact space. A compactification exists for any topological space, and any 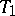 -space has a compactification which is a
-space has a compactification which is a 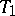 -space, but Hausdorff compactifications of completely-regular spaces (cf. Completely-regular space) are of the greatest interest. A compactification usually means a Hausdorff compactification, but arbitrary compactifications may also be considered. It was proved by P.S. Aleksandrov [1] that all locally compact Hausdorff spaces may be completed to a
-space, but Hausdorff compactifications of completely-regular spaces (cf. Completely-regular space) are of the greatest interest. A compactification usually means a Hausdorff compactification, but arbitrary compactifications may also be considered. It was proved by P.S. Aleksandrov [1] that all locally compact Hausdorff spaces may be completed to a 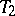 -compactum by the addition of one point (cf. Aleksandrov compactification). P.S. Urysohn [2] proved that every normal space with a countable base can be imbedded in the Hilbert cube, which implies that it has a compactification of countable weight [2]. The term "compactification" was first introduced by A.N. Tikhonov [3], who defined the class of completely-regular spaces and proved that completely-regular spaces and only such spaces have a Hausdorff compactification, a completely-regular space of weight
-compactum by the addition of one point (cf. Aleksandrov compactification). P.S. Urysohn [2] proved that every normal space with a countable base can be imbedded in the Hilbert cube, which implies that it has a compactification of countable weight [2]. The term "compactification" was first introduced by A.N. Tikhonov [3], who defined the class of completely-regular spaces and proved that completely-regular spaces and only such spaces have a Hausdorff compactification, a completely-regular space of weight  having a Hausdorff compactification of weight
having a Hausdorff compactification of weight  .
.
Two compactifications 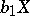 and
and  of a space
of a space 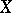 are said to be equivalent (
are said to be equivalent (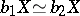 ) if there exists a homeomorphism
) if there exists a homeomorphism  which is the identity on
which is the identity on 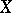 . Often it is the imbedding
. Often it is the imbedding 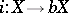 itself which is called a compactification. If this definition is accepted, two extensions
itself which is called a compactification. If this definition is accepted, two extensions 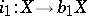 and
and  are equivalent if there exists a homeomorphism
are equivalent if there exists a homeomorphism  such that
such that 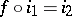 . Equivalent compactifications are usually not distinguished, and a class of mutually equivalent compactifications of a space
. Equivalent compactifications are usually not distinguished, and a class of mutually equivalent compactifications of a space  is viewed as a compactification of that space. In such a case one can speak of the set
is viewed as a compactification of that space. In such a case one can speak of the set 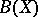 of Hausdorff compactifications of a given (completely-regular) space
of Hausdorff compactifications of a given (completely-regular) space  , since the cardinality of any Hausdorff extension of
, since the cardinality of any Hausdorff extension of  is at most
is at most  , while the topologies on a given set
, while the topologies on a given set 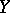 also form a set of cardinality
also form a set of cardinality  .
.
A compactification 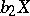 follows a compactification
follows a compactification 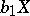 (
(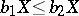 ) if there exists a continuous mapping
) if there exists a continuous mapping  which is the identity on
which is the identity on 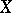 . The successor relation converts
. The successor relation converts 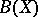 into a partially ordered set. E. Čech [4] and M.H. Stone [5] showed that the set
into a partially ordered set. E. Čech [4] and M.H. Stone [5] showed that the set 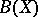 contains a largest element
contains a largest element 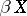 , the Stone–Čech compactification (or maximal compactification).
, the Stone–Čech compactification (or maximal compactification).
The problem of the intrinsic description of all Hausdorff compactifications of a given completely-regular space 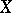 has been solved [6] by constructing the compactifications of an arbitrary proximity space, thus proving that to each proximity
has been solved [6] by constructing the compactifications of an arbitrary proximity space, thus proving that to each proximity  on
on  which is compatible with the topology there corresponds a unique compactification
which is compatible with the topology there corresponds a unique compactification 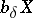 which induces the initial proximity
which induces the initial proximity 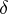 on
on 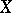 , i.e.
, i.e.
 |
The maximal compactification 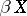 is generated by the following proximity
is generated by the following proximity  :
:
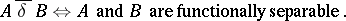 |
The Aleksandrov compactification 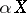 of a locally compact Hausdorff space
of a locally compact Hausdorff space 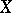 is generated by the proximity
is generated by the proximity 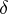 :
:
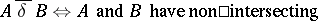 |
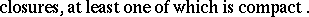 |
The correspondence  is an isomorphism between the partially ordered set of proximities on
is an isomorphism between the partially ordered set of proximities on 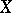 which are compatible with the topology, and the set
which are compatible with the topology, and the set 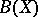 . The correspondence
. The correspondence 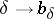 is extended to a functor from the category of spaces with a proximity that is compatible with the topology, with proximally continuous mappings, into the category of
is extended to a functor from the category of spaces with a proximity that is compatible with the topology, with proximally continuous mappings, into the category of 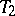 -compacta, with continuous mappings.
-compacta, with continuous mappings.
A major part of the theory of compactifications is concerned with methods of constructing them. It was shown by Tikhonov that on each completely-regular space 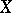 of weight
of weight  there exists a set of functions
there exists a set of functions 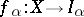 of cardinality
of cardinality  such that their diagonal product realizes an imbedding of
such that their diagonal product realizes an imbedding of 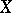 into the cube
into the cube 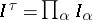 (cf. Tikhonov cube). After this, a compactification in
(cf. Tikhonov cube). After this, a compactification in 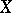 of weight
of weight  is obtained as the closure of
is obtained as the closure of 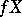 in
in 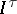 . Čech constructed the maximal compactification of a space
. Čech constructed the maximal compactification of a space 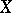 using the diagonal product of all continuous functions
using the diagonal product of all continuous functions 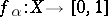 . Stone constructed the maximal compactification by using Boolean algebras and rings of continuous functions.
. Stone constructed the maximal compactification by using Boolean algebras and rings of continuous functions.
One of the fundamental methods in compactification theory is Aleksandrov's method of centred systems of open sets [7], which was initially used for the construction of the maximal compactification, and was subsequently extensively utilized by many mathematicians. Thus, it was found that any Hausdorff extension of an arbitrary Hausdorff space  can be realized as a space of centred systems of sets open in
can be realized as a space of centred systems of sets open in  . The method of centred systems was utilized to construct an isomorphism between the set of proximities on a completely-regular space and the set of all its Hausdorff compactifications. The method was applied to the construction of Hausdorff compactifications of
. The method of centred systems was utilized to construct an isomorphism between the set of proximities on a completely-regular space and the set of all its Hausdorff compactifications. The method was applied to the construction of Hausdorff compactifications of 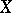 from a subordination given on it.
from a subordination given on it.
H. Wallman [9] constructed the maximal compactification of a normal space 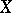 as the space of maximal centred systems of closed sets of this space. The space
as the space of maximal centred systems of closed sets of this space. The space 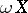 of maximal centred systems of closed sets of a
of maximal centred systems of closed sets of a 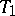 -space
-space 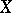 is its
is its 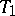 -compactification and is called the Wallman compactification. This compactification, like the Stone–Čech compactification, differs from other compactifications by the similarity between the combinatorial construction and the extendable space, the maximality (in a certain sense), and the possibility of extending continuous mappings.
-compactification and is called the Wallman compactification. This compactification, like the Stone–Čech compactification, differs from other compactifications by the similarity between the combinatorial construction and the extendable space, the maximality (in a certain sense), and the possibility of extending continuous mappings.
The method of centred systems of closed sets makes it possible to generalize the Wallman compactification. In a completely-regular space 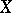 let there be given a base of closed sets
let there be given a base of closed sets 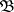 which is a ring of sets, i.e. contains the intersection and the union of any two elements in it. The base
which is a ring of sets, i.e. contains the intersection and the union of any two elements in it. The base 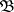 is said to be normal if: 1) for any point
is said to be normal if: 1) for any point 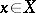 and any element
and any element 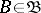 not containing this point there exist elements
not containing this point there exist elements 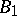 and
and 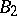 of the base such that
of the base such that 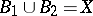 ,
, 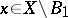 ,
, 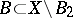 ; and 2) for any two elements
; and 2) for any two elements 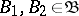 there exist elements
there exist elements  such that
such that 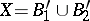 ,
, 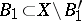 ,
, 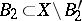 . The space of maximal centred systems of a normal base-ring with the standard given base of closed sets on it is a Hausdorff compactification of
. The space of maximal centred systems of a normal base-ring with the standard given base of closed sets on it is a Hausdorff compactification of 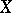 , known as a compactification of Wallman type; all Hausdorff compactifications are of Wallman type (Ul'yanov's theorem, cf. [22]).
, known as a compactification of Wallman type; all Hausdorff compactifications are of Wallman type (Ul'yanov's theorem, cf. [22]).
Other methods of constructing compactifications include: the method of maximal ideals of the rings of continuous functions [11]; the method of completion of pre-compact uniform structures (cf. [12] and Completion of a uniform space); and the method of projective spectra (cf. Projective spectrum of a ring) [10]. It has been shown in this connection that the least upper bound of the maximal finite spectrum of any  -space
-space 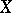 is its Wallman compactification
is its Wallman compactification 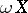 , and this bound coincides with the maximal compactification
, and this bound coincides with the maximal compactification  if and only if
if and only if 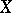 is a quasi-normal space.
is a quasi-normal space.
The importance of the theory of compactifications is explained by the fundamental role of compact spaces in topology and functional analysis. The possibility of imbedding a topological space in a  -compactum makes it possible to describe many properties of completely-regular spaces in terms of properties of
-compactum makes it possible to describe many properties of completely-regular spaces in terms of properties of 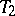 -compacta, which are usually simpler. Thus, normal spaces satisfying the first axiom of countability are homeomorphic if and only if their maximal compactifications are homeomorphic. Hence, the study of normal spaces satisfying the first axiom of countability can be reduced, in principle, to the study of
-compacta, which are usually simpler. Thus, normal spaces satisfying the first axiom of countability are homeomorphic if and only if their maximal compactifications are homeomorphic. Hence, the study of normal spaces satisfying the first axiom of countability can be reduced, in principle, to the study of  -compacta. The topological invariants of an extendable space can very often be expressed in a simple manner in terms of imbeddings of the space in its compactifications (cf. Feathered space; Completeness (in topology); Normally-imbedded subspace). Thus, for a space
-compacta. The topological invariants of an extendable space can very often be expressed in a simple manner in terms of imbeddings of the space in its compactifications (cf. Feathered space; Completeness (in topology); Normally-imbedded subspace). Thus, for a space 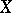 to be a space of countable type, i.e. a space in which any
to be a space of countable type, i.e. a space in which any 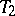 -compactum is contained in a
-compactum is contained in a 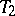 -compactum of countable character, it is necessary and sufficient for some (and hence, for all) compactifications
-compactum of countable character, it is necessary and sufficient for some (and hence, for all) compactifications 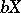 that the remainder
that the remainder 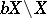 be finally compact. The spaces
be finally compact. The spaces 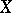 of countable type are also interesting because they are normally adjacent to the remainder in all their compactifications
of countable type are also interesting because they are normally adjacent to the remainder in all their compactifications 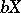 , which means that any two non-intersecting sets, closed in the remainder, have neighbourhoods which do not intersect in
, which means that any two non-intersecting sets, closed in the remainder, have neighbourhoods which do not intersect in 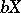 . As regards imbedding in compactifications, it is finally-compact spaces which are dual with spaces of countable type. A space
. As regards imbedding in compactifications, it is finally-compact spaces which are dual with spaces of countable type. A space 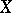 is finally compact if and only if one (and hence all) of its compactifications
is finally compact if and only if one (and hence all) of its compactifications 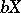 have the following property: For any
have the following property: For any 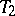 -compactum
-compactum 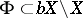 there exists in the remainder a
there exists in the remainder a 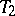 -compactum
-compactum 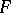 containing it, which has countable character in
containing it, which has countable character in 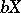 .
.
Compactifications are particularly important in dimension theory. This is explained, in particular, by the equations
 |
which are valid for every normal space, and by the equation
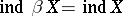 |
for a perfectly-normal space 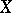 . One of the first theorems regarding the dimensional properties of compactifications was the theorem according to which any
. One of the first theorems regarding the dimensional properties of compactifications was the theorem according to which any  -dimensional normal space with a countable base has a Hausdorff compactification of the same (countable) weight and the same dimension [16]. It has been proved [20] that only the peripherally-compact spaces (cf. Peripherically-compact space) among the normal spaces
-dimensional normal space with a countable base has a Hausdorff compactification of the same (countable) weight and the same dimension [16]. It has been proved [20] that only the peripherally-compact spaces (cf. Peripherically-compact space) among the normal spaces 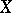 with a countable base have a compactification
with a countable base have a compactification 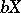 with zero-dimensional (in the sense of the dimension ind) remainder
with zero-dimensional (in the sense of the dimension ind) remainder 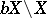 (cf. Freudenthal compactification). Under such compactifications of this space there is a largest. These two results were the starting point of a large number of studies. Thus, it was proved [8] that for any completely-regular space
(cf. Freudenthal compactification). Under such compactifications of this space there is a largest. These two results were the starting point of a large number of studies. Thus, it was proved [8] that for any completely-regular space 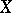 of weight
of weight  with
with 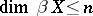 , in particular for any normal space
, in particular for any normal space 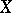 of weight
of weight  with
with  , there exists a compactification
, there exists a compactification 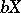 of weight
of weight  and dimension
and dimension 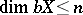 . On the other hand, a completely-regular space
. On the other hand, a completely-regular space 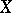 is peripherally compact if and only if
is peripherally compact if and only if 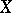 has a compactification with its remainder zero-dimensionally imbedded in it [8]. The remainder
has a compactification with its remainder zero-dimensionally imbedded in it [8]. The remainder 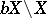 is said to be zero-dimensionally imbedded in
is said to be zero-dimensionally imbedded in 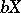 (or, relatively zero-dimensional in
(or, relatively zero-dimensional in 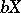 ) whenever there exists a base
) whenever there exists a base 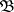 of
of 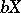 such that
such that
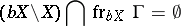 |
for all 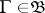 , where
, where 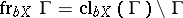 , the frontier (or boundary) of
, the frontier (or boundary) of 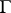 .
.
Of obvious importance in the theory of compactifications are perfect compactifications (cf. Perfect compactification) . All perfect compactifications 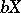 of a space
of a space  are monotone images of the maximal compactification
are monotone images of the maximal compactification  (in particular,
(in particular,  itself is perfect as well) and have, like
itself is perfect as well) and have, like 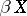 , a combinatorial structure similar to
, a combinatorial structure similar to  , but, unlike in the case of
, but, unlike in the case of 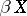 ,
, 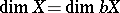 is not always valid, not even for metric spaces
is not always valid, not even for metric spaces  . Whereas
. Whereas 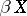 is the largest perfect compactification, a minimal perfect compactification exists only if
is the largest perfect compactification, a minimal perfect compactification exists only if 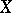 has a compactification with a punctiform remainder (in particular, if
has a compactification with a punctiform remainder (in particular, if 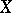 is peripherally compact). The minimal perfect compactification is unique in having a punctiform remainder, and is the largest of all extensions with a punctiform remainder.
is peripherally compact). The minimal perfect compactification is unique in having a punctiform remainder, and is the largest of all extensions with a punctiform remainder.
The concept of a compactification is useful in the study of the dimension of the remainder. If a metric space 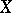 with a countable base is imbedded in a compactum
with a countable base is imbedded in a compactum 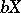 with remainder
with remainder 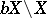 of dimension
of dimension 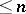 , there exists in
, there exists in 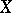 an (open) base such that the intersection of the boundaries of any of its
an (open) base such that the intersection of the boundaries of any of its 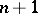 elements is compact . This condition is not sufficient for the space
elements is compact . This condition is not sufficient for the space  to have a compactification
to have a compactification 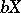 with
with 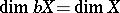 and
and 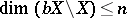 . Moreover, if
. Moreover, if  is a perfect compactification of
is a perfect compactification of 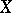 , if
, if 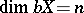 , and if
, and if  for any compact set
for any compact set  , then
, then 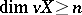 for any compactification
for any compactification 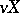 with a punctiform remainder . Both perfect and maximal compactifications are of interest in the context of possible extensions of mappings. Thus, in particular, if the spaces
with a punctiform remainder . Both perfect and maximal compactifications are of interest in the context of possible extensions of mappings. Thus, in particular, if the spaces 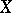 and
and 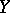 have minimal perfect extensions
have minimal perfect extensions 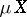 and
and  , any perfect mapping
, any perfect mapping  can be extended to yield a mapping
can be extended to yield a mapping 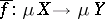 .
.
A topological space 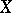 of weight
of weight  is zero-dimensional (i.e.
is zero-dimensional (i.e. 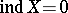 ) if and only if [16] it has a zero-dimensional compactification
) if and only if [16] it has a zero-dimensional compactification 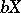 of weight
of weight  , so that a space
, so that a space 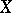 of weight
of weight  with
with 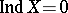 has a compactification of equal weight and equal dimension. In the case of a completely-regular space
has a compactification of equal weight and equal dimension. In the case of a completely-regular space 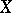 with
with 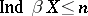 there exists a compactification
there exists a compactification  with
with 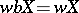 and
and 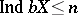 , and this statement is valid for transfinite values of
, and this statement is valid for transfinite values of 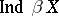 as well (
as well (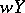 denotes the weight of
denotes the weight of 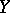 ). It follows that a strongly-paracompact metric space
). It follows that a strongly-paracompact metric space 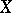 has a compactification
has a compactification 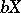 such that
such that 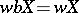 ,
, 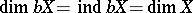 , and there exists a space
, and there exists a space  such that
such that  for all its compactifications in
for all its compactifications in 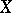 [21].
[21].
There are several theorems concerning compactifications of infinite-dimensional spaces. Thus, the maximal compactification 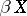 of a normal
of a normal 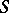 -weakly infinite-dimensional space
-weakly infinite-dimensional space 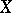 is weakly infinite-dimensional [16]. Any completely-regular space
is weakly infinite-dimensional [16]. Any completely-regular space  of weight
of weight  with a weakly infinite-dimensional compactification (in particular, any normal
with a weakly infinite-dimensional compactification (in particular, any normal  -weakly infinite-dimensional space
-weakly infinite-dimensional space 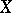 of weight
of weight 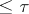 ) has a weakly infinite-dimensional compactification of weight
) has a weakly infinite-dimensional compactification of weight 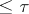 . In these theorems it is not allowed to replace the
. In these theorems it is not allowed to replace the 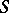 -weak infinite dimension by the
-weak infinite dimension by the 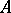 -weak infinite dimension (cf. Weakly infinite-dimensional space). Thus, all compactifications of increasing sums of cubes
-weak infinite dimension (cf. Weakly infinite-dimensional space). Thus, all compactifications of increasing sums of cubes 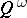 (subsets of the Hilbert cube
(subsets of the Hilbert cube  , consisting of points having only a finite number of non-zero coordinates) are strongly infinite-dimensional spaces .
, consisting of points having only a finite number of non-zero coordinates) are strongly infinite-dimensional spaces .
Yu.M. Smirnov
studied the problems connected with the dimension dim of the remainders of compactifications of proximity spaces and completely-regular spaces. If a proximity space 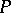 is normally adjacent to
is normally adjacent to 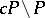 , where
, where  is the (unique) compactification of
is the (unique) compactification of 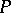 , then
, then 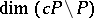 is equal to the smallest of the numbers
is equal to the smallest of the numbers 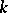 such that a bordering of multiplicity
such that a bordering of multiplicity 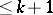 can be inscribed into each extended bordering (cf. Bordering of a space). A space
can be inscribed into each extended bordering (cf. Bordering of a space). A space 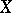 of countable type has a compactification
of countable type has a compactification 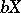 with a remainder of dimension
with a remainder of dimension 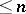 if and only if a bordering structure of multiplicity
if and only if a bordering structure of multiplicity 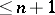 , with the basis property, exists in
, with the basis property, exists in  . Moreover, a consequence of the existence of a compactification with a remainder of dimension
. Moreover, a consequence of the existence of a compactification with a remainder of dimension  in a given space
in a given space 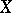 is the existence of a compactification
is the existence of a compactification 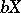 of weight
of weight  with a remainder of dimension
with a remainder of dimension 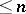 .
.
The partially ordered set 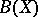 of all Hausdorff compactifications of a space
of all Hausdorff compactifications of a space 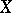 is a complete semi-lattice (with respect to the operation of taking the supremum). The set
is a complete semi-lattice (with respect to the operation of taking the supremum). The set 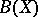 is a complete lattice if and only if
is a complete lattice if and only if 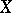 is a locally compact space. If the spaces
is a locally compact space. If the spaces 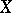 and
and 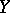 are locally compact, the lattices
are locally compact, the lattices  and
and 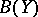 are isomorphic if and only if the remainders
are isomorphic if and only if the remainders 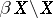 and
and 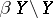 are homeomorphic [18]. Conditions to be met by a (perfect) mapping
are homeomorphic [18]. Conditions to be met by a (perfect) mapping 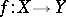 for the lattices
for the lattices  and
and 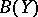 to be isomorphic, are unknown. The compactifications of perfect irreducible inverse images of a space
to be isomorphic, are unknown. The compactifications of perfect irreducible inverse images of a space  are described by
are described by 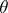 -proximities (cf. Proximity) on the space
-proximities (cf. Proximity) on the space 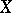 and form a complete semi-lattice with respect to a naturally definable order [19]. Compactifications of perfect irreducible inverse images of a space
and form a complete semi-lattice with respect to a naturally definable order [19]. Compactifications of perfect irreducible inverse images of a space 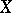 are also connected with
are also connected with 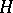 -closed extensions of
-closed extensions of 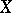 .
.
References
| [1] | P.S. Aleksandrov, P. Urysohn, "Mémoire sur les espaces topologiques compacts" , Koninkl. Nederl. Akad. Wetensch. , Amsterdam (1929) |
| [2] | P.S. Urysohn, "Works on topology and other areas of mathematics" , 1–2 , Moscow-Leningrad (1951) (In Russian) |
| [3] | A.N. [A.N. Tikhonov] Tikhonoff, "Ueber die topologische Erweiterung von Räumen" Math. Ann. , 102 (1929) pp. 544–561 |
| [4] | E. Čech, "On bicompact spaces" Ann. of Math. (2) , 38 : 4 (1937) pp. 823–844 |
| [5] | M.H. Stone, "Applications of the theory of Boolean rings to general topology" Trans. Amer. Math. Soc. , 41 (1937) pp. 375–481 |
| [6] | Yu.M. Smirnov, "On proximity spaces" Mat. Sb. , 31 (73) : 3 (1952) pp. 543–574 (In Russian) |
| [7] | P.S. Aleksandrov, "On bicompact extensions of topological spaces" Mat. Sb. , 5 (47) : 2 (1939) pp. 403–424 (In Russian) (German abstract) |
| [8] | P.S. Aleksandrov, "Some results in the theory of topological spaces, obtained within the last twenty-five years" Russian Math. Surveys , 15 : 2 (1960) pp. 23–83 Uspekhi Mat. Nauk , 15 : 2 (1960) pp. 25–95 |
| [9] | H. Wallman, "Separation spaces" Ann. of Math. (2) , 42 : 3 (1941) pp. 687–697 |
| [10] | V.I. [V.I. Zaitsev] Zaicev, "Projection spectra" Trans. Moscow Math. Soc. , 27 (1972) pp. 135–200 Trudy Moskov. Mat. Obshch. , 27 (1972) pp. 129–193 |
| [11] | I.M. Gel'fand, D.A. Raikov, G.E. Shilov, "Commutative normed rings" Uspekhi Mat. Nauk , 1 : 2 (1946) pp. 48–146 (In Russian) |
| [12] | P. Samuel, "Ultrafilters and compactifications of uniform spaces" Trans. Amer. Math. Soc. , 64 (1948) pp. 100–132 |
| [13] | A.V. Arkhangel'skii, " -compactifications of metric spaces" Math. USSR-Sb. , 20 : 1 (1973) pp. 85–94 Mat. Sb. , 91 (132) : 1 (1973) pp. 78–87 -compactifications of metric spaces" Math. USSR-Sb. , 20 : 1 (1973) pp. 85–94 Mat. Sb. , 91 (132) : 1 (1973) pp. 78–87 |
| [14] | V. Malykhin, "On countable spaces having no bicompactification of countable tightness" Soviet Math. Dokl. , 13 : 5 (1972) pp. 1407–1411 Dokl. Akad. Nauk SSSR , 206 : 6 (1972) pp. 1293–1296 |
| [15a] | P.S. Aleksandrov, "Some basic directions in general topology" Russian Math. Surveys , 19 : 6 (1964) pp. 1–40 Uspekhi Mat. Nauk , 19 : 6 (1964) pp. 3–46 |
| [15b] | P.S. Aleksandrov, "Corrections to "Some basic directions in general topology" " Russian Math. Surveys , 20 : 1 (1965) pp. 177 Uspekhi Mat. Nauk , 20 : 1 (1965) pp. 253–254 |
| [16] | P.S. Aleksandrov, B.A. Pasynkov, "Introduction to dimension theory" , Moscow (1973) (In Russian) |
| [17a] | Yu.M. Smirnov, "On the dimension of remainders of compact Hausdorff extensions of proximity and topological spaces" Mat. Sb. , 69 : 1 (1966) pp. 141–160 (In Russian) |
| [17b] | Yu.M. Smirnov, "On the dimension of remainders of compact Hausdorff extensions of proximity and topological spaces" Mat. Sb. , 71 : 4 (1966) pp. 454–482 (In Russian) |
| [18] | K.D. Magill, jr, "The lattice of compactifications of a locally compact group" Proc. London Math. Soc. (3) , 18 (1968) pp. 231–244 |
| [19] | V.V. Fedorchuk, "Perfect irreducible mappings and generalized proximities" Math. USSR-Sb. , 5 : 4 (1968) pp. 489–508 Mat. Sb. , 76 (118) : 4 (1968) pp. 513–536 |
| [20] | H. Freudenthal, "Neuaufbau der Endentheorie" Ann. of Math. (2) , 43 : 2 (1942) pp. 261–279 |
| [21] | Yu.M. Smirnov, "An instance of a one-dimensional normal space contained in no one-dimensional bicompact space" Dokl. Akad. Nauk SSSR , 117 : 6 (1957) pp. 939–942 (In Russian) |
| [22] | V.M. Ul'yanov, "Solution of a basic problem on compactifications of Wallman type" Soviet Math. Dokl. , 18 (1977) pp. 567–571 Dokl. Akad. Nauk SSSR , 233 : 6 (1977) pp. 1056–1059 |
Comments
A space is called punctiform if no compact connected subset of it has more than one point. A centred system of closed sets is a collection of closed sets such that any finite intersection is non-empty; such a family is also called a filtered system of sets or simply a filter.
References
| [a1] | A.V. Arkhangel'skii, V.I. Ponomarev, "Fundamentals of general topology: problems and exercises" , Reidel (1984) (Translated from Russian) |
Compactification. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Compactification&oldid=46408