Bruhat-Tits building
A building (cf. also Tits building) which is defined for a connected reductive group over a field which is complete with respect to a non-trivial discrete valuation and has a perfect residue class field. It is the topological realization of the (poly-) simplicial complex of all parahoric subgroups. One way to define it more precisely is as follows.
Let 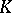 be a field which is complete with respect to the non-trivial valuation
be a field which is complete with respect to the non-trivial valuation 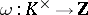 and has a perfect residue class field. Let
and has a perfect residue class field. Let 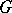 be a connected, reductive
be a connected, reductive 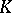 -group. First, assume
-group. First, assume 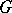 to be semi-simple (cf. Semi-simple group).
to be semi-simple (cf. Semi-simple group).
Apartments.
Let 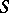 be a maximal
be a maximal 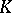 -split torus in
-split torus in 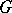 and denote by
and denote by 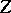 (respectively,
(respectively, 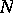 ) the centralizer (respectively, normalizer; cf. Normalizer of a subset) of
) the centralizer (respectively, normalizer; cf. Normalizer of a subset) of  in
in 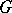 . Let
. Let 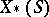 (respectively,
(respectively, 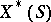 ) denote the group of cocharacters (respectively, characters) of
) denote the group of cocharacters (respectively, characters) of  and let
and let 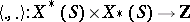 be the canonical perfect pairing. Then there is a unique group homomorphism
be the canonical perfect pairing. Then there is a unique group homomorphism 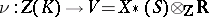 such that
such that  for all
for all 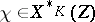 (i.e., the group of
(i.e., the group of 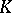 -rational characters of
-rational characters of 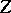 ). One can show that there is a unique affine
). One can show that there is a unique affine 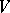 -space
-space 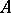 together with a group homomorphism
together with a group homomorphism 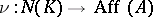 (i.e., the affine bijections
(i.e., the affine bijections 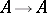 ) extending
) extending 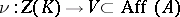 , called the (empty) apartment associated with
, called the (empty) apartment associated with 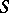 .
.
Filtrations of the root subgroups.
Denote by 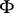 the root system of
the root system of  with respect to
with respect to 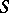 and, for
and, for 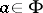 , by
, by  the root subgroup of
the root subgroup of 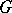 associated with
associated with  . Then, for
. Then, for 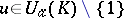 , the set
, the set 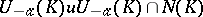 contains exactly one element, denoted by
contains exactly one element, denoted by  . An affine mapping
. An affine mapping 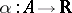 is called an affine root if the vector part
is called an affine root if the vector part  of
of  is contained in
is contained in 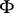 and if there exists a
and if there exists a  such that
such that  . In that case
. In that case 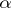 is abbreviated as
is abbreviated as 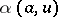 . For
. For 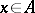 and
and 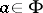 , let
, let 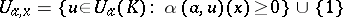 and let
and let  be the subgroup of
be the subgroup of 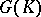 generated by all
generated by all  for
for 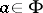 .
.
Simplicial structures.
Two points 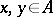 are called equivalent if
are called equivalent if 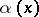 and
and 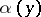 have the same sign or are both equal to
have the same sign or are both equal to  for all affine roots
for all affine roots  . One obtains a (poly-) simplicial complex (i.e., a direct product of simplicial complexes) in
. One obtains a (poly-) simplicial complex (i.e., a direct product of simplicial complexes) in  by defining the faces to be the equivalence classes.
by defining the faces to be the equivalence classes.
Building.
Let  , where
, where 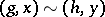 if there exists an
if there exists an  such that
such that 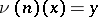 and
and 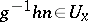 . There is a canonical
. There is a canonical 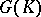 -action on
-action on 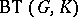 induced by left-multiplication on the first factor of
induced by left-multiplication on the first factor of  . One can identify
. One can identify 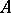 with its canonical image in
with its canonical image in 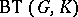 . The subsets of the form
. The subsets of the form 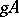 , for
, for 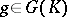 , are called apartments and the subsets of the form
, are called apartments and the subsets of the form  , for
, for 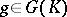 and
and 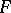 a face in
a face in 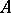 , are called faces. One can equip
, are called faces. One can equip 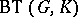 with a metric which is
with a metric which is  -invariant. This metric coincides on
-invariant. This metric coincides on  with the metric induced by the scalar product on
with the metric induced by the scalar product on 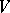 which is invariant under the Weyl group of
which is invariant under the Weyl group of 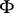 . The metric space
. The metric space 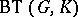 together with these structures is called the Bruhat–Tits building of
together with these structures is called the Bruhat–Tits building of 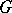 .
.
If 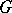 is not semi-simple, the Bruhat–Tits building of
is not semi-simple, the Bruhat–Tits building of 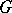 is, by definition, the Bruhat–Tits building of the derived group (cf. Commutator subgroup) of
is, by definition, the Bruhat–Tits building of the derived group (cf. Commutator subgroup) of 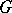 .
.
Example.
Assume 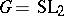 , and denote by
, and denote by 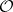 and
and  the valuation ring of
the valuation ring of 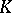 and a uniformizer of
and a uniformizer of  , respectively. An
, respectively. An  -lattice is a free
-lattice is a free 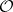 -submodule of
-submodule of  of rank
of rank  . Then the Bruhat–Tits building of
. Then the Bruhat–Tits building of 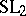 is the topological realization of the following simplicial complex: the
is the topological realization of the following simplicial complex: the  -simplices are the
-simplices are the 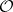 -lattices in
-lattices in  up to homothety and the
up to homothety and the 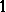 -simplices are pairs
-simplices are pairs 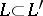 of
of 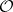 -lattices in
-lattices in 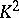 up to homothety with
up to homothety with 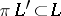 .
.
General references for Bruhat–Tits buildings are [a1] and [a2]. (The situations considered there are more general.) A good overview can be found in [a3].
Originally, the Bruhat–Tits building was the essential technical tool for the classification of reductive groups over local fields (cf. Reductive group). There are further applications, e.g. in the representation theory of reductive groups over local fields, in the theory of p-adic symmetric spaces and in the theory of Shimura varieties.
References
| [a1] | F. Bruhat, J. Tits, "Groupes réductifs sur un corps local I, II" IHES Publ. Math. , 41,50 (1972–1984) |
| [a2] | E. Landvogt, "A compactification of the Bruhat–Tits building" , Lecture Notes in Mathematics , 1619 , Springer (1996) |
| [a3] | J. Tits, "Reductive groups over local fields" , Proc. Symp. Pure Math. , 33 , Amer. Math. Soc. (1979) pp. 29–69 |
Bruhat-Tits building. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Bruhat-Tits_building&oldid=46168