A module 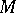 over a ring of Witt vectors
over a ring of Witt vectors 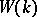 (cf. Witt vector), where
(cf. Witt vector), where 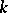 is a perfect field of characteristic
is a perfect field of characteristic  , provided with two endomorphisms
, provided with two endomorphisms 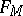 and
and 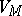 which satisfy the following relationships:
which satisfy the following relationships:
Here 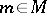 ,
, 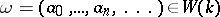 ,
, 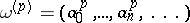 . In an equivalent definition,
. In an equivalent definition, 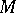 is a left module over the ring
is a left module over the ring 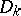 (the Dieudonné ring) generated by
(the Dieudonné ring) generated by 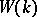 and two variables
and two variables 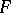 and
and 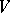 connected by the relations
connected by the relations
For any positive integer  there exists an isomorphism
there exists an isomorphism
where 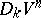 is the left ideal generated by
is the left ideal generated by  and
and 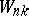 is the
is the  -scheme of truncated Witt vectors. Dieudonné modules play an important part in the classification of unipotent commutative algebraic groups [1]. Dieudonné modules is also the name given to left modules over the completion
-scheme of truncated Witt vectors. Dieudonné modules play an important part in the classification of unipotent commutative algebraic groups [1]. Dieudonné modules is also the name given to left modules over the completion 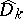 of
of 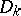 with respect to the topology generated by the powers of the two-sided ideal
with respect to the topology generated by the powers of the two-sided ideal 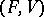 of
of 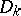 .
.
References
| [1] | J. Dieudonné, "Lie groups and Lie hyperalgebras over a field of characteristic  . VI" Amer. J. Math. , 79 : 2 (1957) pp. 331–388 . VI" Amer. J. Math. , 79 : 2 (1957) pp. 331–388 |
| [2] | M. Demazure, P. Gabriel, "Groupes algébriques" , 1 , Masson (1970) MR0302656 MR0284446 Zbl 0223.14009 Zbl 0203.23401 |
| [3] | Yu.I. Manin, "The theory of commutative formal groups over fields of finite characteristic" Russian Math. Surveys , 28 : 5 (1963) pp. 1–83 Uspekhi Mat. Nauk , 18 : 6 (1963) pp. 3–90 MR157972 Zbl 0128.15603 |
Dieudonné modules also play a role in different cohomology theories of algebraic varieties over fields of positive characteristic, [a1], and in the (classification) theory of formal groups [3], [a2]. Cartier duality [a2], [a3] (cf. Formal group) provides the link between the use of Dieudonné modules in formal group theory (historically the first) and its use in the classification theory of commutative unipotent algebraic groups [2].
References
| [a1] | P. Berthelot, A. Ogus, "Notes on crystalline cohomology" , Princeton Univ. Press (1978) MR0491705 Zbl 0383.14010 |
| [a2] | M. Hazewinkel, "Formal groups and applications" , Acad. Press (1978) MR0506881 MR0463184 Zbl 0454.14020 |
| [a3] | P. Cartier, "Groups algébriques et groupes formels" , Coll. sur la théorie des groupes algébriques. Bruxelles, 1962 , CBRM (1962) pp. 87–111 |
How to Cite This Entry:
Dieudonné module. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dieudonn%C3%A9_module&oldid=44298
This article was adapted from an original article by I.V. Dolgachev (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098.
See original article over a ring of Witt vectors
over a ring of Witt vectors 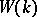 (cf. Witt vector), where
(cf. Witt vector), where 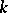 is a perfect field of characteristic
is a perfect field of characteristic  , provided with two endomorphisms
, provided with two endomorphisms 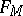 and
and 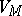 which satisfy the following relationships:
which satisfy the following relationships:

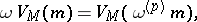
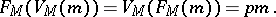
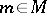 ,
, 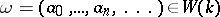 ,
, 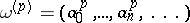 . In an equivalent definition,
. In an equivalent definition, 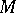 is a left module over the ring
is a left module over the ring 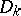 (the Dieudonné ring) generated by
(the Dieudonné ring) generated by 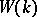 and two variables
and two variables 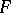 and
and 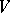 connected by the relations
connected by the relations


 there exists an isomorphism
there exists an isomorphism

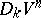 is the left ideal generated by
is the left ideal generated by  and
and 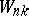 is the
is the  -scheme of truncated Witt vectors. Dieudonné modules play an important part in the classification of unipotent commutative algebraic groups [1]. Dieudonné modules is also the name given to left modules over the completion
-scheme of truncated Witt vectors. Dieudonné modules play an important part in the classification of unipotent commutative algebraic groups [1]. Dieudonné modules is also the name given to left modules over the completion 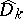 of
of 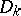 with respect to the topology generated by the powers of the two-sided ideal
with respect to the topology generated by the powers of the two-sided ideal 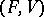 of
of 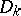 .
.
 . VI" Amer. J. Math. , 79 : 2 (1957) pp. 331–388
. VI" Amer. J. Math. , 79 : 2 (1957) pp. 331–388