Complete algebraic variety

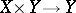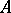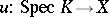A generalization of the concept of a compact complex algebraic variety. A separated variety is called complete if for any variety the projection is a closed morphism, i.e. it maps closed subsets of (in the Zariski topology) into closed subsets of . There is what is called the valuative completeness criterion: For any discrete valuation ring with field of fractions and any morphism there should be a unique morphism 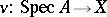that extends . This condition is an analogue of the requirement that any sequence in 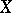has a limit point.

Any projective variety is complete, but not vice versa. For any complete algebraic variety 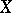there exists a projective variety 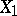and a projective birational morphism 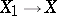(Chow's lemma). For any algebraic variety 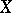there exists an open imbedding into a complete variety (Nagata's theorem). A generalization of the concept of a complete algebraic variety to the relative case is that of a proper morphism of schemes.

References

[1]	R. Hartshorne, "Algebraic geometry" , Springer (1977) MR0463157 Zbl 0367.14001
[2]	I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) MR0447223 Zbl 0362.14001