Primitive ideal
From Encyclopedia of Mathematics
right primitive ideal
A two-sided ideal 
of an associative ring 
(cf. Associative rings and algebras) such that the quotient ring 
is a (right) primitive ring. Analogously, by using left primitive rings one can define left primitive ideals. The set 
of all primitive ideals of a ring, endowed with some topology, is useful in the study of various classes of rings. Usually 
is topologized using the following closure relation:
 |
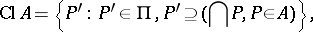where 
is a subset in 
. The set of all primitive ideals of a ring endowed with this topology is called the structure space of this ring.
References
| [1] | N. Jacobson, "Structure of rings" , Amer. Math. Soc. (1956) |
How to Cite This Entry:
Primitive ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Primitive_ideal&oldid=39352
Primitive ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Primitive_ideal&oldid=39352
This article was adapted from an original article by K.A. Zhevlakov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article