Heaps and semi-heaps

Algebras with one ternary operation that satisfies certain identities. Heaps are defined by the identities

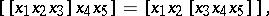while semi-heaps are defined by the identities

All heaps are also semi-heaps.

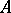If, in the set of all one-to-one mappings of a set into a set 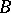, one defines the ternary operation that puts an ordered triplet of mappings 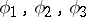into correspondence with the mapping that is the composite of 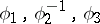, then 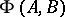is a heap. Any heap is isomorphic to some heap of one-to-one mappings. If a ternary operation is introduced into an arbitrary group 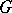by putting 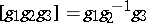, a heap is obtained (the heap associated with the given group). The concept of a heap was introduced in the study of the above ternary operation on an Abelian group [1]. Heaps have been studied from their abstract aspect [2], [3]. In particular, it was shown by R. Baer [2] that if an arbitrary given element 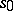is fixed in a heap 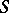, then the operations defined by the equations 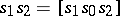, 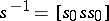define a group structure on in which 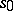is the unit; the heap associated with this group coincides with the initial heap, while the groups obtained from a heap by fixing various elements of it are isomorphic. In other words, the variety of all heaps is equivalent to the variety of all groups.

The set of all binary relations (cf. Binary relation) between the elements of two sets 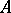and 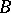is a semi-heap with respect to the triple multiplication 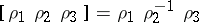. The set of all invertible partial mappings of 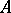into is also closed with respect to the triple multiplication and is a generalized heap [4], i.e. a semi-heap with the identities

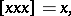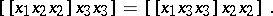Generalized heaps find application in the foundations of differential geometry in the study of coordinate atlases [5]. Heaps are closely connected with semi-groups with involution. If an involution 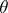, which is an anti-automorphism, is defined on a semi-group 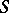, then the ternary operation 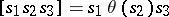converts into a semi-heap. Any semi-heap is isomorphic to a sub-semi-heap of a semi-group with involution, [4].

References

[1]	H. Prüfer, "Theorie der Abelschen Gruppen" Math. Z. , 20 (1924) pp. 165–187
[2]	R. Baer, "Zur Einführung des Scharbegriffs" J. Reine Angew. Math. , 160 (1929) pp. 199–207
[3]	J. Certaine, "The ternary operation of a group" Bull. Amer. Math. Soc. , 49 (1943) pp. 869–877
[4]	V.V. Vagner, "The theory of generalized heaps and of generalized groups" Mat. Sb. , 32 : 3 (1953) pp. 545–632 (In Russian)
[5]	V.V. Vagner, "Foundations of differential geometry and contemporary algebra" , Proc. 4-th All-Union. Mat. Conf. , 1 , Leningrad (1963) pp. 17–29 (In Russian)
[6]	M. Flato, "Deformation view of physical theories" Czechoslovak J. Phys. , B32 (1982) pp. 472–475