Primitive ring

right primitive ring

An associative ring (cf. Associative rings and algebras) with a right faithful irreducible module. Analogously (using a left irreducible module) one defines a left primitive ring. The classes of right and left primitive rings do not coincide. Every commutative primitive ring is a field. Every semi-simple (in the sense of the Jacobson radical) ring is a subdirect product of primitive rings. A simple ring is either primitive or radical. The primitive rings with non-zero minimal right ideals can be described by a density theorem. The primitive rings with minimum condition for right ideals (i.e. the Artinian primitive rings) are simple.

A ring 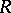is primitive if and only if it has a maximal modular right ideal 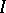(cf. Modular ideal) that does not contain any two-sided ideal of 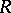distinct from the zero ideal. This property can be taken as the definition of a primitive ring in the class of non-associative rings.

References

[1]	N. Jacobson, "Structure of rings" , Amer. Math. Soc. (1956)
[2]	I.N. Herstein, "Noncommutative rings" , Math. Assoc. Amer. (1968)

Comments

Semi-simple rings in the sense of the Jacobson radical are now called semi-primitive rings. Primitive rings with polynomial identities are central simple finite-dimensional algebras. Primitive rings with minimal one-sided ideals have a socle which can be described completely [a1].

References

[a1]	L.H. Rowen, "Ring theory" , I, II , Acad. Press (1988)