Vector bundle, algebraic

A morphism of varieties 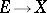which locally (in the Zariski topology) has the structure of a projection of a direct product 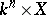to , such that the glueing preserves the linear structure of the vector space. Here, 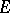is said to be the fibre space (bundle space), is the base and is the rank or dimension of the bundle. The morphisms of an algebraic vector bundle are defined in the same manner as in topology. A more general definition, which is suitable for any scheme, involves the concept of a sheaf. Let 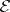be a locally free sheaf of 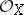-modules of finite (constant) rank; then the affine morphism 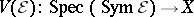, where 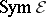is a sheaf of symmetric algebras of 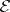, is said to be the vector bundle associated with . This terminology is sometimes also retained when 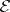is an arbitrary quasi-coherent sheaf. The sheaf 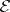can be uniquely reconstructed from the algebraic vector bundle 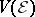, and the category of algebraic vector bundles on 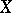is dual to the category of locally free sheaves of -modules. Moreover, for an 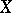-scheme 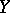the set of 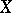-morphisms bijectively corresponds to the set of 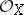-module homomorphisms 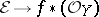, where is a structure morphism of the 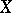-scheme . In particular, the sheaf of germs of cross-sections of the algebraic vector bundle 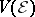is identified with the sheaf dual to 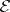. The algebraic vector bundle 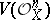is said to be the trivial vector bundle of rank . The set of all algebraic vector bundles of rank on the scheme is in one-to-one correspondence with the cohomology set , where is the sheaf of automorphisms of the trivial vector bundle of rank . Algebraic vector bundles of rank 1 are said to be line bundles; they correspond to invertible sheaves of 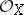-modules and are closely connected with divisors (cf. Divisor) on 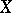; the set of line bundles with the tensor product operation forms a group 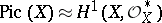(cf. Picard group).

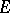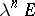As in topology, the direct sum, tensor product, dual bundle, symmetric and exterior power, induced algebraic vector bundle, etc., are defined for algebraic vector bundles. In the case of an algebraic vector bundle of rank , the line bundle is said to be the determinant bundle. To an algebraic vector bundle 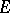one can associate the projective bundle , just like to a vector space one can associate a projective space (see Projective scheme).

Examples of non-trivial algebraic vector bundles include canonical algebraic vector bundles on a Grassmann manifold; in particular, there exists a canonical line bundle on the projective space which corresponds to the sheaf 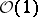. If the algebraic vector bundle 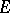on the scheme is a subbundle of a trivial algebraic vector bundle, such an imbedding will define a morphism from 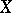to the corresponding Grassmann manifold, the canonical algebraic vector bundle on the Grassmann manifold being used to induce this morphism. Line bundles which determine an imbedding of in 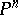are said to be very ample (see Ample vector bundle).

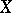Other examples of algebraic vector bundles include the tangent bundle on a smooth variety and bundles constructed from it by different operations (see Tangent bundle; Canonical class; Normal bundle).

An algebraic vector bundle on a variety defined over the field of complex numbers 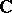may be regarded both as an analytic and as a topological (in the complex topology) algebraic vector bundle. Analytic and algebraic vector bundles are equivalent on a complete algebraic variety (see Comparison theorem (algebraic geometry); Vector bundle, analytic). Topological vector bundles do not always allow an algebraic structure, and even when they do, such a structure is usually not unique. If an algebraic vector bundle is regarded as topological, topological methods may be used; in particular, the Chern classes (cf. Chern class) of algebraic vector bundles may be introduced. There also exists an abstract definition of Chern classes which involves the 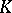-functor or one of the variants of étale cohomology.

The properties of an algebraic vector bundle will depend on whether its base is a complete or an affine scheme. If the base is affine, 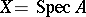, algebraic vector bundles correspond to projective modules of finite type over the ring 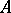(cf. Projective module). If the rank of the algebraic vector bundle 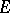is higher than the dimension of the base 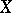, 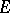may be represented as 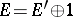, where 1 is the one-dimensional trivial bundle. 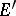is usually not uniquely defined. Moreover, if the rank of 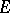is higher than the dimension of the base and 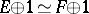, then [4]. If is a non-singular one-dimensional scheme (i.e. 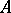is a Dedekind ring), any algebraic vector bundle is the direct sum of a trivial and a line bundle. This also applies to algebraic vector bundles on a non-singular affine surface over an algebraically closed field which is birationally equivalent to a ruled surface.

The case of a projective base.

The study of line bundles on projective varieties is a classical problem in algebraic geometry (cf. Picard group; Picard scheme). The study of algebraic vector bundles of higher ranks began in 1957, when it was shown by A. Grothendieck that algebraic vector bundles on the projective line are direct sums of line bundles. M. Atiyah classified algebraic vector bundles on an elliptic curve 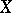: If denotes the set of algebraic vector bundles of non-decomposable (into a direct sum) algebraic vector bundles of rank and degree 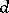( "degree" is to be understood as the degree of the determinant of the bundle), then 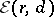is identical with the points of the curve 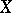itself [3].

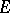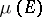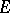The concept of stable algebraic vector bundles proved useful in the study of algebraic vector bundles on curves. For a given algebraic vector bundle , let be equal to ; the bundle is then said to be stable (or semi-stable) if for any subbundle 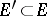one has 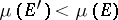(or 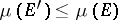). A stable bundle is simple (i.e. 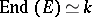) and, in particular, not decomposable. An algebraic vector bundle of degree 0 on an algebraic curve of genus 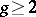is stable if and only if it is associated with an irreducible unitary representation of the fundamental group 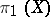[1]. Let 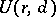be the set of all semi-stable algebraic vector bundles of rank and degree 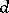which are direct sums of stable algebraic vector bundles, and let 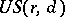be the subset of stable algebraic vector bundles. 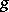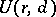If the genus of a smooth curve is higher than 1, has the natural structure of a normal projective variety of dimension 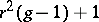, while 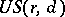is an open smooth subvariety of 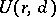[1]. 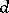If and are coprime, 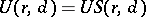and is therefore smooth. The moduli space of semi-stable algebraic vector bundles has been studied extensively. 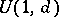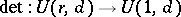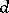It is known, in fact, that is a component of the Picard scheme for ; the fibres of the determinant mapping are unirational varieties; if and are coprime, 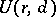uniquely determines the original curve 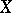. Since the universal family of algebraic vector bundles does not always exist over , 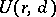is not a representing object of a suitable functor [1]. Most of these results were obtained for the field 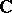, even though many of them are also valid for an arbitrary algebraically closed field. Certain special facts are known for algebraic vector bundles on algebraic surfaces and projective spaces [5].

References

[1]	M. Narasimhan, C.S. Seshadri, "Stable and unitary vector bundles on a compact Riemann surface" Ann. of Math. , 82 (1965) pp. 540–567
[2]	A.N. Tyurin, "On the classification of -dimensional vector bundles over an algebraic curve of arbitrary genus" Izv. Akad. Nauk SSSR Ser. Mat. , 30 (1966) pp. 1353–1366 (In Russian)
[3]	M.F. Atiyah, "Vector bundles over an elliptic curve" Proc. London Math. Soc. (3) , 7 (1957) pp. 414–452
[4]	H. Bass, "Algebraic 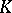-theory" , Benjamin (1968)
[5]	I.V. Dolgachev, V.A. Iskovskikh, "Geometry of algebraic varieties" J. Soviet Math. , 5 : 6 (1976) pp. 803–864 Itogi Nauk. i Tekhn. Algebra Topol. Geom. , 12 (1974) pp. 77–170

Comments

In recent work of S. Donaldson (cf. [a2]–[a3]) the moduli space of stable rank- vector bundles on a compact complex algebraic surface was shown to be isomorphic to the moduli space of instanton unitary vector bundles on the corresponding 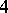-dimensional smooth manifold. An instanton vector bundle on a Riemannian manifold is a differential bundle together with a connection whose curvature form satisfies a certain system of non-linear differential equations. The moduli space of such instantons is independent of the choice of a Riemannian metric and is a new invariant of smooth 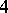-manifolds. The theory of algebraic vector bundles allows one to compute these invariants in some cases. In this way there were given the first examples of homeomorphic but not diffeomorphic compact simply-connected smooth -manifolds.

Many new ideas in the theory of algebraic vector bundles on algebraic curves and projective spaces were inspired by theoretical physics (twistor theory, Yang–Mills theory and string theory).

References

[a1]	M.F. Atiyah, R. Bott, "The Yang–Mills equations over Riemann surfaces" Philos. Trans. Roy. Soc. London Ser. A , 308 (1982) pp. 523–615
[a2]	S.K. Donaldson, "Instantons in Yang–Mills theory" D. Quillen (ed.) G. Segal (ed.) S. Tsou (ed.) , The Interface of Mathematics and Particle Physics , Oxford Univ. Press (1990)
[a3]	S. Donaldson, P. Kronkheimer, "The geometry of four manifolds" , Oxford Science Publ. (1990)
[a4]	R. Friedman, J. Morgan, "Algebraic surfaces and 4-manifolds: some conjectures and speculations" Bull. Amer. Math. Soc. , 18 (1988) pp. 1–15
[a5]	C. Okonek, A. van de Ven, "Stable bundles, instantons and 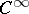-structures on algebraic surfaces" A.G. Vitushkin (ed.) et al. (ed.) , Several Complex Variables VI , Encycl. Math. Sci. , 69 , Springer (1990) pp. 197–249
[a6]	C. Okonek, M. Schneider, H. Spindler, "Vector bundles on complex projective spaces" , Birkhäuser (1987)
[a7]	A. Rudakov, et al., "Helices and vector bundles" , Cambridge Univ. Press (1990)
[a8]	C. Seshadri, "Fibrés vectoriels sur les courbes algebriques" Astérisque , 96 (1982)
[a9]	A. Tyurin, "Algebraic geometric aspects of smooth structure I. The Donaldson polynomials" Russian Math. Surveys , 44 (1989) pp. 113–178 Uspekhi Mat. Nauk , 44 (1989) pp. 93–143
[a10]	D. Mumford, J. Fogarty, "Geometric invariant theory" , Springer (1982)
[a11]	R. Hartshorne, "Algebraic vector bundles on projective spaces: a problem list" Topology , 18 (1979) pp. 117–128
[a12]	A. van de Ven, "Twenty years of classifying vector bundles" A. Beauville (ed.) , Algebraic geometry (Angers, 1979) , Sijthoff & Noordhoff (1980) pp. 3–20
[a13]	R. Harshorne, "Four years of algebraic vector bundles" A. Beauville (ed.) , Journées de géometrie algébrique d'Angers (1979) , Sijthoff & Noordhoff (1980) pp. 21–28