Poisson process

2020 Mathematics Subject Classification: Primary: 60G55 Secondary: 60K25 [MSN][ZBL]

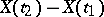A stochastic process with independent increments , , having a Poisson distribution. In the homogeneous Poisson process

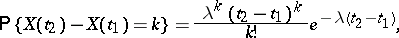(1)

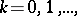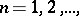for any . The coefficient is called the intensity of the Poisson process . The trajectories of the Poisson process are step-functions with jumps of height 1. The jump points form an elementary flow describing the demand flow in many queueing systems. The distributions of the random variables are independent for and have exponential density , .

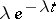One of the properties of a Poisson process is that the conditional distribution of the jump points when is the same as the distribution of the variational series of independent samples with uniform distribution on . On the other hand, if is the variational series described above, then as , and 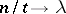one obtains in the limit the distribution of the jumps of the Poisson process.

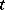In an inhomogeneous process the intensity depends on the time and the distribution of is defined by the formula

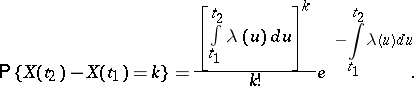Under certain conditions a Poisson process can be shown to be the limit of the sum of a number of independent "sparse" flows of fairly general form as this number increases to infinity. For certain paradoxes which have been obtained in connection with Poisson processes see [3].

References

[1]	A.A. Borovkov, "Wahrscheinlichkeitstheorie" , Birkhäuser (1976) (Translated from Russian)
[2]	I.I. Gikhman, A.V. Skorokhod, M.I. Yadrenko, "Probability theory and mathematical statistics" , Kiev (1979) (In Russian)
[3]	W. Feller, "An introduction to probability theory and its applications" , 2 , Wiley (1971) pp. Chapt. 1

References

[a1]	J.W. Cohen, "The single server queue" , North-Holland (1982)
[a2]	G.G. Székely, "Paradoxes in probability theory and mathematical statistics" , Reidel (1986)