Kempf vanishing theorem

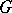Let denote an algebraically closed field and let be a semi-simple linear algebraic group over 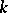. Cohomology will always refer to the coherent cohomology on an algebraic variety, that is, Čech cohomology with coefficients in a coherent sheaf of modules. Let 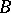be a Borel subgroup of , i.e., a maximal connected solvable subgroup of 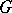. The homogeneous space 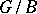is called the generalized flag variety of 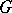and it is a projective algebraic variety (cf. also Projective algebraic set). Let } be the projection.

For each character 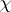of 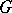(cf. Character of a group) one may construct an invertible sheaf on 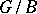, denoted by 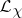. Its value on the open subset 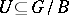is

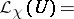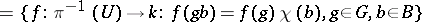By the Borel–Weil theorem, its space of global sections is a finite-dimensional representation of 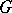containing a unique irreducible submodule and a unique highest weight vector. The 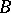-weight of the highest weight vector is 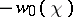, where 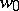is the inversion in the Weyl group of 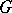(cf. also Weight of a representation of a Lie algebra). This space of global sections is non-zero if and only if 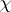is dominant, i.e., if 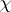is a non-negative integral linear combination of the fundamental dominant weights of 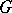. Then the Kempf vanishing theorem states: Let be semi-simple, let 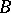be a Borel subgroup and let 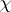be a dominant character of 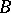. Then for all , .

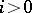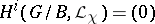Over fields of characteristic zero, this is a simple consequence of the Bott theorem (see [a2]), an algebraic proof of which has been given by M. Demazure in 1968 (see [a3] and [a6]). An algebraic proof was needed at the time because no proof of the Kodaira vanishing theorem (cf. Kodaira theorem) over fields of characteristic zero in the algebraic category was then known. (It is known to fail in positive characteristic.) At the time there were two pressing motives for establishing it over fields of positive characteristic. The first was purely rooted in the theory of algebraic groups. Over fields of characteristic zero, algebraic proofs that the character of the -module 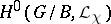for dominant 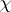is given by the Weyl character formula had been given by T.A. Springer [a17] and M. Demazure [a4]. These proofs imply that the Weyl character gives the Euler characteristic of 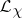even over fields of positive characteristic. Hence Kempf vanishing would have established that is described by the Weyl character.

For G. Kempf, the result was important to his path-breaking work on the theta-divisors in Jacobians of curves (see [a8]). This work involved computations of Chern classes in which they were represented as pull-backs of Schubert cells. Hence, controlling the cohomological behaviour of line bundles on flag varieties and Schubert cells was critical to his results. Consequently, he established the theorem for special linear groups in [a8]. His methods involved a careful examination of the geometry of Schubert cells and induction on the dimensions of Schubert cells.

Kempf's work inspired the attempt by Lakshmi Bai, C. Musili and C.S. Seshadri in [a12] and subsequent papers to generalize Kempf's methods and to prove vanishing. They failed to obtain vanishing, but that work, along with Demazure's paper [a5] on desingularizing generalized Schubert cells, established the basic geometrical understanding of the geometry of generalized flag varieties which is used by all contemporary researchers in the area.

Kempf continued his work on the problem, [a9], [a10], finally giving the first general proof of vanishing in [a11]. The paper contains a masterful examination of the geometry of the singularities of Schubert cells, the construction of certain special desingularizations and ingenious inductive arguments. It represents the high point of the use of geometrical methods in the area.

The modern approach to the proof of this theorem begins with [a7]. In this paper, W.J. Haboush gave a proof which completely circumvented the geometrical problems and which was based on the observation that the direct image of 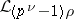under the th Frobenius power is free of rank 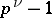. This same proof was included in H. Andersen's paper [a1], in which the method is also somewhat extended.

The Frobenius methods of [a7] inspired the extremely important series of papers by V.B. Mehta, S. Ramanan and above all A. Ramanathan [a13], [a14], [a15], [a16]. They used the notion of Frobenius splitting to establish the basic geometric vanishing properties of line bundles on generalized flag varieties and generalized Schubert varieties. These have turned out to be the most efficient proofs even of the characteristic zero results, which follows from the results over fields of positive characteristic. In fact, Ramanathan was able to use Frobenius splitting methods in [a16] to repair a serious error in [a5] discovered by V. Kac in the early 1980s.

See also Flag structure; Schubert variety; Representation theory; Character formula.

References

[a1]	H.H. Andersen, "The Frobenius morphism on the cohomology of homogeneous vector bundles on " Ann. of Math. (2) , 112 (1980) pp. 113–121
[a2]	R. Bott, "Homogeneous vector bundles" Ann. of Math. (2) , 66 (1957) pp. 203–248
[a3]	M. Demazure, "Une démonstration algébrique d'une théorème de Bott" Invent. Math. , 5 (1968) pp. 349–356
[a4]	M. Demazure, "Sur la formule des charactères de H. Weyl" Invent. Math. , 9 (1969) pp. 249–252
[a5]	M. Demazure, "Desingularization des varietés de Schubert généralisées" Ann. Sci. Ecole Norm. Sup. Ser. 4 , 7 (1974) pp. 53–88
[a6]	M. Demazure, "A very simple proof of Bott's theorem" Invent. Math. , 33 (1976) pp. 271–272
[a7]	W.J. Haboush, "A short proof of the Kempf vanishing theorem" Invent. Math. , 56 (1980) pp. 109–112
[a8]	G. Kempf, "Schubert methods with an application to algebraic curves" , Math. Centre , Amsterdam (1971) (mimeographed notes)
[a9]	G. Kempf, D. Laksov, "The determinental formula of Schubert calculus" Acta Math. , 132 (1974) pp. 153–162
[a10]	G. Kempf, etAAsal., "Vanishing theorems for flag manifolds" Amer. J. Math. , 98 (1976) pp. 325–331
[a11]	G. Kempf, etAAsal., "Linear systems on homogeneous spaces" Ann. of Math. , 103 (1976) pp. 557–591
[a12]	Lakshmi Bai, C. Musili, C.S. Seshadri, "Cohomology of line bundles on 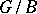" Ann. Sci. Ecole Norm. Sup. Ser. 4 , 7 (1974) pp. 89–137
[a13]	V.B. Mehta, A. Ramanathan, etAAsal., "Frobenius splitting and cohomology vanishing for Schubert varieties" Ann. of Math. (2) , 122 (1985) pp. 27–40
[a14]	V.B. Mehta, A. Ramanathan, etAAsal., "Schubert varieties in 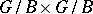" Compositio Math. , 67 (1988) pp. 355–358
[a15]	S. Ramanan, A. Ramanathan, "Projective normality of flag varieties and Schubert varieties" Invent. Math. , 79 (1985) pp. 217–114
[a16]	A. Ramanathan, "Schubert varieties are arithmetically Cohen–Macaulay" Invent. Math. , 80 (1985) pp. 283–294
[a17]	T.A. Springer, "Weyl's character formula for algebraic groups" Invent. Math. , 5 (1968) pp. 85–105