Complex torus

A complex Abelian Lie group obtained from the -dimensional complex space by factorizing with respect to a lattice 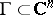of rank 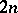. Every connected compact complex Lie group is a complex torus [1]. Every Hermitian scalar product in 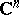defines on 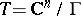a translation-invariant Kähler metric. Complex tori can also be characterized as the only compact parallelizable Kähler manifolds [2]. The group of automorphisms of the complex manifold 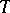is the same as the holomorph of the group 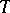as a complex Lie group (cf. Holomorph of a group).

Holomorphic 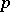-forms on a complex torus have the form

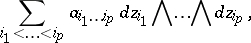where , are the coordinates in 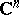and the Dolbeault cohomology ring 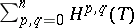is naturally isomorphic to 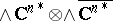(see [1]).

As real Lie groups, all -dimensional complex tori are 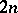-dimensional tori and are isomorphic for fixed . From the point of view of their complex structure their behaviour is extremely complicated. A basis for a lattice 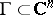can be given by a matrix of dimension , called the period matrix of the torus 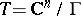. Tori 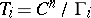with period matrices 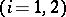are isomorphic (as complex Lie groups or as complex manifolds) if and only if there exist matrices and such that .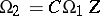

The period matrix of an -dimensional torus can be reduced to the form , where 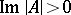. Tori with matrices of this form generate a holomorphic family that gives an effectively-parametrized versal deformation of any -dimensional complex torus depending on 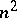parameters . In particular, for 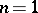, the parameter space is the upper half-plane , and the set of isomorphism classes of one-dimensional complex tori can be identified with the quotient where is the modular group.

Complex tori that are algebraic varieties are called Abelian varieties (cf. Abelian variety). A complex torus is an Abelian variety if and only there exists in 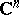a Hermitian scalar product whose imaginary part is integer-valued on [1]. In terms of the period matrix this is the Riemann–Frobenius condition: There should exist a skew-symmetric matrix 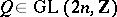such that and is positive definite. When 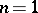this condition always holds; the corresponding algebraic curves are elliptic (cf. Elliptic curve). The period matrix

provides an example of a two-dimensional complex torus that is not an algebraic variety. On this torus there are not even non-constant meromorphic functions [5]. A necessary and sufficient condition that an -dimensional complex torus be algebraic is the existence on it of algebraically-independent meromorphic functions.

Interest in complex tori originated in the 19th century in connection with the study of Abelian functions (cf. Abelian function) and Jacobi varieties of algebraic curves (cf. Jacobi variety). To any -dimensional compact Kähler manifold there is related a collection of complex tori, its intermediate Jacobi varieties [7].

References

[1]	D. Mumford, "Abelian varieties" , Oxford Univ. Press (1974)
[2]	H.C. Wang, "Complex parallisable manifolds" Proc. Amer. Math. Soc. , 5 (1954) pp. 771–776
[3a]	K. Kodaira, D.C. Spencer, "On deformations of complex analytic structures I" Ann. of Math. , 67 (1958) pp. 328–400
[3b]	K. Kodaira, D.C. Spencer, "On deformations of complex analytic structures II" Ann. of Math. , 67 (1958) pp. 403–466
[4]	A. Weil, "Introduction à l'Aeetude des variétés kahlériennes" , Hermann (1958)
[5]	C.L. Siegel, "Automorphe Funktionen in mehrerer Variablen" , Math. Inst. Göttingen (1955)
[6]	R.O. Wells jr., "Differential analysis on complex manifolds" , Springer (1980)
[7]	S.S. Chern, "Complex manifolds without potential theory" , Springer (1979)