Primary ideal
of a commutative ring 
An ideal 
such that if 
and 
, then either 
or 
for some natural number 
. In the ring 
of integers a primary ideal is an ideal of the form 
, where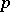 
is a prime number and 
is a natural number. In commutative algebra an important role is played by the representation of an arbitrary ideal of a commutative Noetherian ring as an intersection of a finite number of primary ideals — a primary decomposition. More generally, let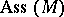 
denote the set of prime ideals of a ring 
that are annihilators of non-zero submodules of a module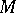 
. A submodule 
of a module 
over a Noetherian ring 
is called primary if 
is a one-element set. If 
is commutative, then every proper submodule of a Noetherian 
-module that cannot be represented as an intersection of submodules strictly containing it is primary. In the non-commutative case this is not true and therefore attempts have been undertaken to construct various non-commutative generalizations of the notion of primarity. E.g., a proper submodule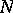 
of a module 
is called primary if for every non-zero injective submodule 
of the injective hull 
of the module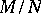 
(cf. Injective module) the intersection of the kernels of the homomorphisms from 
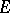into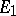 
is trivial. Another successful generalization is the notion of a tertiary ideal [4]: A left ideal 
of a left Noetherian ring 
is called tertiary if, for any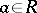 
, 
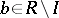, it follows from 
that, for any 
, there is an element 
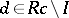such that 
. Both these generalizations lead to a non-commutative analogue of primary decomposition. Every tertiary ideal of a Noetherian ring 
is primary if and only if 
satisfies the Artin–Rees condition: For arbitrary left ideals 
of 
there is a natural number 
such that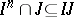 
(cf. [3]).
References
| [1] | N. Bourbaki, "Elements of mathematics. Commutative algebra" , Addison-Wesley (1972) (Translated from French) |
| [2] | O. Zariski, P. Samuel, "Commutative algebra" , 1 , Springer (1975) |
| [3] | O. Goldman, "Rings and modules of quotients" J. of Algebra , 13 : 1 (1969) pp. 10–47 |
| [4] | L. Lesieur, R. Croisot, "Algèbre noethérienne noncommutative" , Gauthier-Villars (1963) |
Primary ideal. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Primary_ideal&oldid=39565