Witch of Agnesi
versiera
A plane curve, given in the Cartesian orthogonal coordinate system by the equation
$$y(a^2+x^2)=a^3,\quad a>0.$$
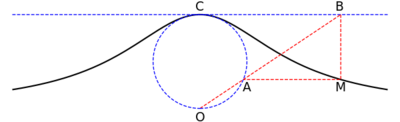
If $a$ is the diameter of a circle with centre at the point $(0,a/2)$, $OA$ is a secant, $CB$ and $AM$ are parallel to the $x$-axis, and $BM$ is parallel to the $y$-axis (see Fig.), then the witch of Agnesi is the locus of the points $M$. If the centre of the generating circle and the tangent $CB$ are shifted along the $y$-axis, the curve thus obtained is called Newton's aguinea and is a generalization of the witch of Agnesi. The curve is named after Maria Gaetana Agnesi (1718-1799), who studied it.
Comments
The unusual name derives from a misreading of the term la versiera (from Latin versoria) "rope that turns a sail" as l'aversiera, "witch".
References
- [1] A.A. Savelov, "Planar curves" , Moscow (1960) (In Russian)
- [a1] J.D. Lawrence, "A catalog of special plane curves" , Dover, reprint (1972)
- [b1] Ian Stewart, Professor Stewart's Cabinet of Mathematical Curiosities, Profile Books (2010) ISBN 1846683459
Witch of Agnesi. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Witch_of_Agnesi&oldid=34039