Fréchet algebra

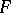-algebra, algebra of type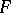

A completely metrizable topological algebra. The joint continuity of multiplication need not be demanded since it follows from the separate continuity (see Fréchet topology). The 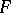-algebras can be classified similarly as the 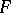-spaces (see Fréchet topology), so one can speak about complete locally bounded algebras, algebras of type 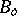(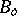-algebras), and locally pseudo-convex 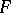-algebras, i.e. 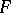-algebras whose underlying topological vector space is a locally bounded space, etc.

Locally bounded algebras of type .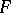

These are also called 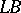-algebras. The topology of an 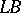-algebra 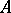can be given by means of a 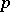-homogeneous norm, 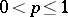, satisfying , 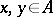(the submultiplicativity condition) and, if 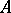has a unity , . The theory of these algebras is analogous to that of Banach algebras (cf. Banach algebra). In particular, a Gel'fand–Mazur-type theorem holds (even if completeness and joint continuity of multiplication is not assumed). For commutative complex algebras there is a Gelfand-type theory (including a holomorphic functional calculus). That means that local boundedness, and not local convexity, is responsible for the theory of Banach algebras. 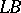For more information on -algebras see [a5], [a6], [a7].

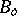-algebras.

The topology of such an algebra can be given by means of a sequence 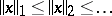of semi-norms (cf. Semi-norm) satisfying

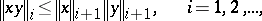(a1)

and, if has a unit , 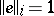for all 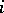. Such an algebra is said to be multiplicatively-convex (-convex) if its topology can be given by means of semi-norms satisfying 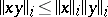instead of (a1) (some authors give the name "Fréchet algebra" to 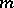-convex 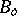-algebras). Each 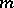-convex 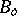-algebra is an inverse (projective) limit of a sequence of Banach algebras. Each complete locally convex topological algebra is an inverse limit of a directed system of 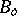-algebras. A Gelfand–Mazur-type theorem holds for -algebras; however, completeness is essential, and a 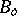-algebra can contain a dense subalgebra isomorphic to the field of all rational functions. The latter is impossible for -convex algebras. 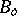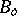The operation of taking an inverse is not continuous on arbitrary -algebras, but it is continuous on -convex -algebras (the operation of taking an inverse is continuous for a general 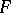-algebra 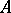if and only if the group 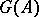of its invertible elements is a 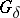-set). A commutative unital 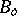-algebra can have dense maximal ideals of infinite codimension also if it is 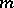-convex, but in the latter case there is always a closed maximal ideal of codimension one, which is the kernel of a multiplicative linear functional (this is not true in general). Every -convex algebra has a functional calculus of several complex variables, but in the non--convex case it is possible that there operate only the polynomials. If a commutative 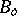-algebra is such that its set 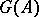of invertible elements is open, then it must be 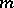-convex. This fails in the non-commutative case, so that a non-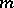-convex 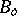-algebra can have all its commutative subalgebras 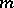-convex. Also, a non-Banach 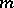-convex algebra can have the property that all its closed commutative subalgebras are Banach algebras. One of most challenging open questions in the theory of topological algebras (see [a2], [a4]) is the question whether for an 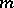-convex 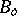-algebra all its multiplicative linear functionals are continuous (this question is also open for algebras of type 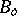and 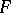). This famous Michael–Mazur problem has been positively solved by R. Arens for finitely generated 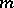-convex algebras. For more on these algebras see [a1], [a3], [a4], [a5], [a6], [a7].

Locally pseudo-convex 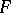-algebras.

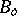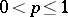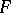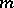These are analogous to -algebras, but with semi-norms replaced by -homogeneous semi-norms, . Their theories are similar; however, there are some differences. E.g., a commutative locally pseudo-convex algebra of type with open set need not be -pseudo-convex. Every -pseudo-convex algebra of type 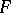is an inverse limit of a sequence of 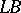-algebras. For more details see [a5], [a6], [a7].

Not much is known about general 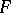-algebras; e.g., a Gelfand–Mazur-type theorem is still (1996) unknown. One result, however, has to be noted. Let 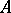be an 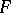-algebra with a continuous involution. Then each positive (i.e. satisfying 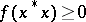) functional on 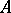is continuous [a2]. Every complete topological algebra is an inverse limit of a directed system of 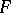-algebras.

References

[a1]	E. Beckenstein, L. Narici, C. Suffel, "Topological algebras" , Amsterdam (1977)
[a2]	T. Husain, ",Multiplicative functionals on topological algebras" , London (1983)
[a3]	A. Mallios, "Topological algebras. Selected topics" , Amsterdam (1986)
[a4]	E. Michael, "Locally multiplicatively-convex topological algebras" , Memoirs , 11 , Amer. Math. Soc. (1952)
[a5]	L. Waelbroeck, "Topological vector spaces and algebras" , Lecture Notes in Mathematics , 230 , Springer (1971)
[a6]	W. Zelazko, "Metric generalizations of Banach algebras" Dissert. Math. , 47 (1965)
[a7]	W. Zelazko, "Selected topics in topological algebras" , Lecture Notes , 31 , Aarhus Univ. (1971)