Small parameter, method of the

in the theory of differential equations

A method for constructing approximate solutions of differential equations and systems depending on a parameter.

1. The method of the small parameter for ordinary differential equations.

Ordinary differential equations arising from applied problems usually contain one or more parameters. Parameters may also occur in the initial data or boundary conditions. Since an exact solution of a differential equation can only be found in very special isolated cases, the problem of constructing approximate solutions arises. A typical scenario is: the equation and the initial (boundary) conditions contain a parameter 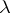and the solution is known (or may be assumed known) for 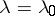; the requirement is to construct an approximate solution for values 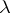close to 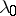, that is, to construct an asymptotic solution as 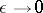, where is a "small" parameter. The method of the small parameter arises, e.g., in the three-body problem of celestial mechanics, which goes back to J. d'Alembert, and was intensively developed at the end of the 19th century.

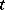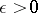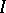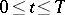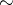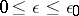The following notations are used below: is an independent variable, is a small parameter, is an interval , and the sign denotes asymptotic equality. All vector and matrix functions which appear in equations and boundary conditions are assumed to be smooth (of class ) with respect to all variables in their domain (with respect to for or ).

1) The Cauchy problem for an -th order system:

(1)

Let the solution 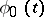of the limit problem (that is, (1) with 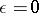) exist and be unique for 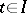. Then there is an asymptotic expansion for the solution 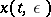of (1) as ,

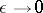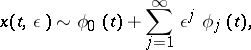(2)

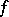which holds uniformly with respect to . This follows from the theorem on the smooth dependence on the parameter of the solution of a system of ordinary differential equations. If the vector functions and 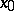are holomorphic for 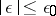, , 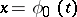, 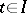then the series in (2) converges to a solution 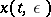for sufficiently small 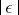uniformly relative to 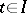(Poincaré's theorem). Similar results hold for boundary value problems for systems of the form (1), if the solution of the corresponding limit problem exists and is unique.

One distinguishes two forms of dependence of equations (or systems) on a small parameter — regular and singular. A system in normal form depends regularly on if all its right-hand sides are smooth functions of for small 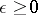; otherwise the system depends singularly on . When the system depends regularly on , the solution of the problem with a parameter, as a rule, converges uniformly on a finite 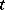-interval as 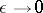to a solution of the limit problem.

2) In the linear theory one considers -th order systems which depend singularly on :

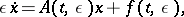where the entries of the 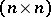-matrix and the components of the vector are complex-valued functions. The central problem in the linear theory is the construction of a fundamental system of solutions of the homogeneous system (that is, for 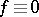), the asymptotic behaviour of which as 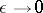is known throughout the interval .

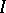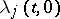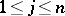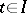The basic result of the linear theory is the following theorem of Birkhoff. Let: 1) the eigenvalues , , of be distinct for ; and 2) the quantities

not change sign. Then there is a fundamental system of solutions of the homogeneous system

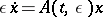for which there is the following asymptotic expansion as :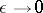

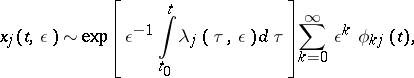(3)

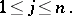This expansion is uniform relative to 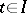and can be differentiated any number of times with respect to 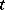and . If does not depend on , that is, 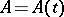, then

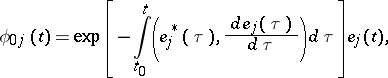where 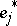, 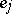are left and right eigenvectors of 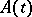normalized by

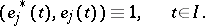Solutions having asymptotic behaviour of the form (3) are called WKB solutions (see WKB method). The qualitative structure of these solutions is as follows. If

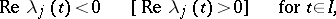then is a vector function of boundary-layer type for (), that is, it is noticeably different from zero only in an -neighbourhood of 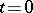(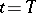). If, however, 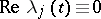, , 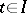then 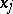strongly oscillates as 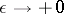and has order 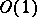on the whole interval .

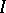If 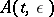is a holomorphic matrix function for 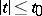, 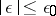and condition 1) is satisfied, then (3) is valid for , , 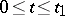where is sufficiently small. A difficult problem is the construction of asymptotics for fundamental systems of solutions in the presence of turning points on , that is, points at which 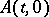has multiple eigenvalues. This problem has been completely solved only for special types of turning points (see [1]). In a neighbourhood of a turning point there is a domain of transition in which the solution is rather complicated and in the simplest case is expressed by an Airy function (cf. Airy functions).

Similar results (see [1], [17]) are valid for scalar equations of the form

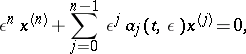where 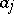is a complex-valued function; the roles of the functions 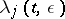are played by the roots of the characteristic equation

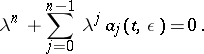WKB solutions also arise in non-linear systems of the form

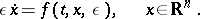The WKB asymptotic expansion (3), under the conditions of Birkhoff's theorem, is valid in an infinite interval 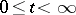(that is, (3) is asymptotic both as and as 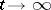) if 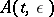is sufficiently well behaved as 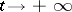, for example, if it rapidly converges to a constant matrix with distinct eigenvalues (see [2]). Many questions of spectral analysis (see [3]) and mathematical physics reduce to singular problems with a small parameter.

3) Of particular interest is the investigation of non-linear systems of the form

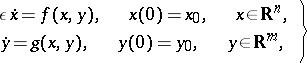(4)

where 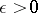is a small parameter. The first equation describes fast motions, the second slow motions. For example, the van der Pol equation reduces by the substitution

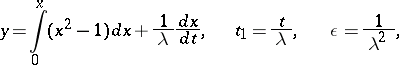for 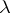large, to the system

which is of the form (4).

For 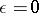the equation of fast motion degenerates to the equation 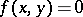. In some closed bounded domain 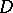of the variable 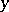, let this equation have an isolated stable continuous root 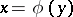(that is, the real parts of the eigenvalues of the Jacobi matrix 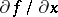are negative for , ); suppose that solutions of (4) and of the degenerate problem

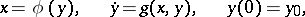(5)

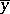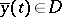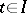exist and are unique for , and let for the function , obtained as the solution of (5), for . If 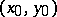is in the domain of influence of the root , then

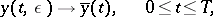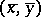as , where is the solution of the degenerate problem (Tikhonov's theorem). Close to the limit transition 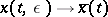is non-uniform — a boundary layer occurs. For problem (4) there is the following asymptotic expansion for the solution:

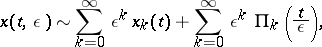(6)

and the asymptotic expansion for 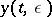has a similar form. In (6) the first sum is the regular part and the second sum is the boundary layer. The regular part of the asymptotic expansion is calculated by standard means: series of the form (2) are substituted into (4), the right-hand sides are expanded as power series in and the coefficients at equal powers of are equated. For the calculation of the boundary-layer part of the asymptotic expansion one introduces a new variable (the fast time) in a neighbourhood of 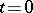and applies the above procedure. There is an interval on the 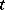-axis on which both the regular (or outer) expansion and the boundary-layer (or inner) expansion are useful. The functions , 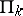are defined by the coincidence of these expansions (the so-called method of matching, see [4], [5]).

Similar results hold when the right-hand side of (4) depends explicitly on 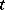, for scalar equations of the form

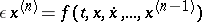(7)

and for boundary value problems for such systems and equations (see Differential equations with small parameter, [6], [7]).

For approximation of the solution of (4) at a break point, where stability is lost (for example, where one of the eigenvalues of for 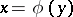is zero), series of the form (5) lose their asymptotic character. In a neighbourhood of a break point the asymptotic expansion has quite a different character (see [8]). The investigation of a neighbourhood of a break point is particularly essential for the construction of the asymptotic theory of relaxation oscillations (cf. Relaxation oscillation).

4) Problems in celestial mechanics and non-linear oscillation theory lead, in particular, to the necessity of investigating the behaviour of solutions of (1) not in a finite interval but in a large 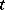-interval of the order of 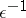or higher. For these problems a method of averaging is widely applied (see Krylov–Bogolyubov method of averaging; Small denominators, [9]–[11]).

5) Asymptotic behaviour of solutions of equations of the form (7) has been investigated, in particular, with the help of the so-called method of multiple scales (see [4], [5]); this method is a generalization of the WKB method. An example of the method has been given using the scalar equation

(8)

which has a periodic solution (see [12]). The solution is sought for in the form

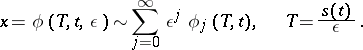(9)

(The functions are called the scales.) If (8) is linear, then 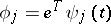and (9) is a WKB solution. In the non-linear case the equations of the first two approximations take the form

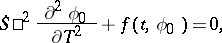where the first equation contains two unknown functions 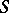and . Let this equation have a solution 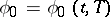periodic in 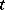. Then the missing equation, from which 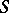is to be determined, is found from the periodicity in 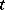of 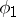and has the form

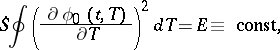where the integral is taken over a period of .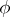

References

[1]	W. Wasov, "Asymptotic expansions for ordinary differential equations" , Interscience (1965)
[2]	M.V. Fedoryuk, "Asymptotic methods in the theory of ordinary linear differential equations" Math. USSR Sb. , 8 : 4 (1969) pp. 451–491 Mat. Sb. , 79 : 4 (1969) pp. 477–516
[3]	M.A. Naimark, "Linear differential operators" , 1–2 , Harrap (1968) (Translated from Russian)
[4]	J.D. Cole, "Perturbation methods in applied mathematics" , Blaisdell (1968)
[5]	A.H. Nayfeh, "Perturbation methods" , Wiley (1973)
[6]	A.B. Vasil'eva, V.F. Butuzov, "Asymptotic expansions of solutions of singularly perturbed equations" , Moscow (1973) (In Russian)
[7]	A.B. Vasil'eva, V.F. Butuzov, "Singularly perturbed equations in critical cases" , Moscow (1978) (In Russian)
[8]	E.F. Mishchenko, N.Kh. Rozov, "Differential equations with small parameters and relaxation oscillations" , Plenum (1980) (Translated from Russian)
[9]	N.N. Bogolyubov, Yu.A. Mitropol'skii, "Asymptotic methods in the theory of non-linear oscillations" , Hindushtan Publ. Comp. , Delhi (1961) (Translated from Russian)
[10]	V.M. Volosov, B.I. Morgunov, "Averaging methods in the theory of non-linear oscillatory systems" , Moscow (1971) (In Russian)
[11]	V.I. Arnol'd, "Small denominators and problems of stability of motion in classical and celestial mechanics" Russian Math. Surveys , 18 : 6 (1963) pp. 86–191 Uspekhi Mat. Nauk , 18 : 6 (1963) pp. 91–192
[12]	G.E. Kuzmak, "Asymptotic solutions of nonlinear second order differential equations with variable coefficients" J. Appl. Math. Mech. , 23 (1959) pp. 730–744 Prikl. Mat. i Mekh. , 23 : 3 (1959) pp. 515–520
[13]	A.A. Andronov, A.A. Vitt, A.E. Khaikin, "The theory of oscillators" , Dover, reprint (1987) (Translated from Russian)
[14]	G.E. Giacaglia, "Perturbation methods in non-linear systems" , Springer (1972)
[15]	N.N. Moiseev, "Asymptotic methods of non-linear mechanics" , Moscow (1969) (In Russian)
[16]	V.F. Butuzov, A.B. Vasil'eva, M.V. Fedoryuk, "Asymptotic methods in the theory of ordinary differential equations" Progress in Math. , 8 (1970) pp. 1–82 Itogi Nauk. Mat. Anal. 1967 (1969) pp. 5–73
[17]	W. Wasow, "Linear turning point theory" , Springer (1985)

N.Kh. RozovM.V. Fedoryuk

2. The method of the small parameter for partial differential equations.

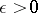As for ordinary differential equations, solutions of partial differential equations can regularly or singularly depend on a small parameter (it is assumed that ). Roughly speaking, regular dependence is observed when the leading terms of the differential operator do not depend on , and the minor terms are smooth functions of for small . The solution is then also a smooth function of . But if any of the leading terms vanish as 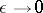, then the solution, as a rule, depends singularly on . In this case one often speaks of partial differential equations "with a small parameter in front of the leading derivatives" . Such a classification is somewhat arbitrary, since the choice of leading terms is not always obvious; also, the parameter itself may also occur in the boundary conditions. In addition, singularities may arise in unbounded domains, even when the small parameter only occurs in the minor derivatives (at infinity they play in a sense an equal, or even more major, role as the leading terms).

For example, consider a second-order elliptic partial differential equation in a bounded domain 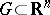. The solution of the problem

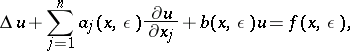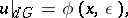is a smooth function of , for small , if the boundary is smooth, if 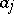, , 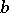, 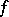are smooth functions of and , and if the limit boundary value problem

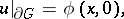is uniquely solvable for any smooth functions 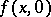, 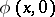. The solution can be expanded in an asymptotic series in powers of :

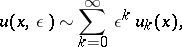(1)

whose coefficients 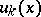are solutions of the same type of boundary value problem and are easily calculated by perturbation theory.

Quite a different situation holds, for example, for the boundary value problem

(2)

since for 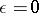the order of the equation is less. The limit problem has the form

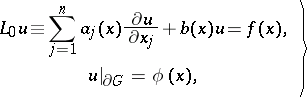(3)

and, in general, is unsolvable. Let , let the characteristics of the limit equation have the form depicted in the figure and let their orientation be induced by the vector field .

Figure: s085820a

If the solution of the limit equation is known at some point, then it is known along all characteristics passing through the point; therefore the boundary value problem (3) is unsolvable for 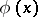arbitrary. As 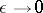, the solution of (2) converges to a solution 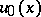of the limit equation 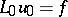which is equal to 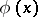on the segments 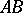and 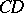. On the remainder of the boundary the boundary conditions are lost. In a neighbourhood of each of the segments and 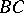, having the typical width and called a boundary layer, the solution of (2) is close to the sum

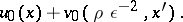Here 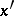is the coordinate along the boundary 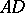(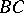), 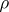is the distance from the boundary along the normal, and 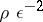is the so-called inner variable. The solution of (2) expands as an asymptotic series of the form (1) everywhere except on the boundary layer and some special characteristic (in the figure, this is 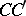). The partial sums of the asymptotic series uniformly approximate the solution of (2) in the domain obtained from 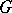by removing fixed neighbourhoods of the lines 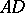, 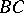and . 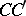In the boundary layer, outside a neighbourhood of the points 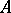, , 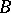, , , 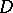to the asymptotic series (1) one adds the asymptotic series

The functions 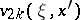decrease exponentially as 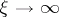. The first asymptotic series is usually called the outer asymptotic series and the second the inner asymptotic series, and the functions 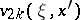are called boundary-layer functions. This terminology, as even the problem itself, comes from the problem of fluid flow around bodies with small viscosity (see Hydrodynamics, mathematical problems in, and also [1]–[4]). This method is called the method of the boundary layer and is essentially the same as the method for ordinary differential equations.

In neighbourhoods of the points 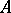, , 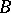, , 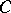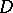at which the characteristics of 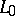touch the boundary, and close to 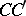, the asymptotic behaviour of the solution is more complicated. The complications arise when the boundary is not everywhere smooth (has corners and, for 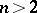, edges). In some simple cases it is possible to construct asymptotic formulas by the addition of supplementary boundary-layer functions depending on more variables, but, as before, tending exponentially to zero at infinity. However, as a rule the picture is more complicated: both the coefficients 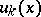of the outer expansion and the coefficients of the inner expansion have essential singularities at the singular points (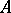, , 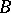, 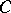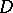in the figure). The asymptotic expansion of the solution, uniformly in the closed domain , can be constructed by the method of multiple scales (by the method of matched asymptotic series, [5]). Some problems for partial differential equations may be investigated by another variant of the method of multiple scales (the method of ascent): the solution is considered as a function of the basic independent variables and auxiliary "fast" variables. As a result the dimension of the original problem is increased but the dependence on the parameter is simplified (see [6]).

If the field of characteristics of the limit operator 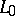has stationary points, then the problem significantly complicates. For example, if 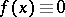, , 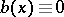and if all the characteristics are directed into the domain, then the solution of (2) tends to a constant as . Finding this constant and constructing an asymptotic series of the solution is a difficult, only partly solved problem (see [7]). Equation (2) describes random perturbations of the dynamical system . Problems in this area were also at the origin of the development of the method of the small parameter in the theory of partial differential equations (see [8]).

If 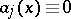in (2) and 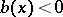, then the asymptotic series is easily found; far from the boundary the series has the form (1) and in the boundary layer, close to the boundary, the asymptotic series

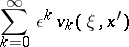is added, where now .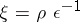

The problem is really complicated if 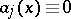, 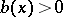. In this case the solution strongly oscillates; the asymptotic methods are the WKB method; semi-classical approximation; the parabolic-equation method, etc.

There is a class of problems in which the boundary of the domain degenerates as 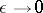. For the sake of being specific, consider the problem

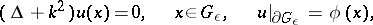(4)

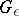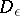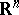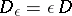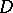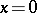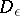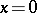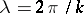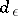where is the exterior of a bounded domain in and ; at infinity the radiation conditions are posed. For example, let , being a fixed domain containing ; then contracts to the point and (4) has no limit. The quantity has the sense of wavelength: here , where is the diameter of 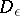, and one speaks of dispersion of wavelengths on the obstacle (or hydrodynamic approximation, or Rayleigh approximation). There are two overlapping zones: the nearer containing 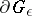, with size tending to zero as , and the farther, the exterior of the domain, contracting to the point as . The asymptotic series of the solution has different forms in these zones. The first boundary value problem for 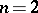turns out to be the most difficult; the inner asymptotic expansion has the form

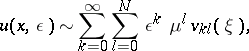where , and 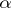is a constant (see [9]). The long-wave approximation has been studied mainly for the Helmholtz equation and for the Maxwell system (see [10], [11]).

Another variant arises when 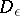contracts to an interval as 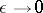; in this case there is a limit problem for 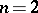but not for 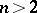. Problems of this type (including those for the Laplace equation, for linear hyperbolic equations and for non-linear partial differential equations) arise in hydrodynamics and aerodynamics, in the theory of diffraction of waves (flow around a thin body of a fluid or a gas). The problem (4) has been investigated for 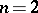(see [12]); for 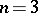it has been investigated if 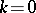and 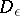is a solid of revolution (see [13]).

Partial differential equations containing a small parameter arise naturally in the study of non-linear oscillations when the perturbation has order but the solution is studied over a large time interval of order 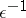. If a continuous medium is considered instead of a system of particles, then partial differential equations arise to which generalizations of the averaging method apply (see [14]).

References

[1]	H. Schlichting, "Boundary layer theory" , McGraw-Hill (1955) (Translated from German)
[2]	M. van Dyke, "Perturbation methods in fluid mechanics" , Parabolic Press (1975)
[3]	M.I. Vishik, L.A. Lyusternik, "Regular degeneracy and boundary layer for linear differential equations with a small parameter" Uspekhi Mat. Nauk , 12 : 5 (1957) pp. 3–122 (In Russian)
[4]	V.A. Trenogin, "The development and applications of the asymptotic method of Lyusternik and Vishik" Russian Math. Surveys , 25 : 4 (1970) pp. 119–156 Uspekhi Mat. Nauk , 25 : 4 (1970) pp. 123–156
[5]	A.H. Nayfeh, "Perturbation methods" , Wiley (1973)
[6]	S.A. Lomov, "The method of perturbations for singular problems" Math. USSR-Izv. , 6 : 3 (1972) pp. 631–648 Izv. Akad. Nauk SSSR Ser. Mat. , 36 : 3 (1972) pp. 635–651
[7]	L.D. Venttsel', "Random perturbations of dynamical systems" , Springer (1984) (Translated from Russian)
[8]	L.S. Pontryagin, A.A. Andronov, A.A. Vitt, Zh. Eksper. i Teoret. Fiz. , 3 : 3 (1933) pp. 165–180
[9]	A.M. Il'in, "A boundary value problem for the second order elliptic equation in a domain with a narrow slit. 2. Domain with a small cavity" Math. USSR Sb. , 32 : 2 (1977) pp. 227–244 Mat. Sb. , 103 : 2 (1977) pp. 265–284
[10]	A.W. Mane, "Theorie der Beugung" S. Flügge (ed.) , Handbuch der Physik , 25/1 , Springer (1961) pp. 218–573
[11]	P.M. Morse, H. Feshbach, "Methods of theoretical physics" , 2 , McGraw-Hill (1953)
[12]	A.M. Il'in, "A boundary value problem for the elliptic equation of second order in a domain with a narrow slit. 1. The two-dimensional case" Math. USSR Sb. , 28 : 4 (1976) pp. 459–480 Mat. Sb. , 99 : 4 (1976) pp. 514–537
[13]	J.D. Cole, "Perturbation methods in applied mathematics" , Blaisdell (1968)
[14]	Yu.A. Mitropol'skii, B.I. Moseenkov, "Asymptotic solutions of partial differential equations" , Kiev (1976) (In Russian)

A.M. Il'inM.V. Fedoryuk

Comments

References [a1]–[a4] are selected from the large Western literature concerning the general subject of singular perturbations, i.e. (a particular kind of) singular small parameter equations; cf. also (the editorial comments headed "singular perturbations" in) Perturbation theory. Another interesting specific topic in the same area is the Hamilton–Jacobi equation, as a limit of parabolic equations with a small parameter [a5].

References

[a1]	R.E. O'Malley, "Introduction to singular perturbations" , Acad. Press (1974)
[a2]	J. Kevorkian, J.D. Cole, "Perturbation methods in applied mathematics" , Springer (1981)
[a3]	J.D. Murray, "Asymptotic analysis" , Springer (1984)
[a4]	P.A. Lagerstrom, "Matched asymptotic expansions" , Springer (1988)
[a5]	D.G. Aronson, J.L. Vazquez, "The porous medium equation as a finite-speed approximation to a Hamiltonian–Jacobi equation" Ann. Inst. H. Poincaré Anal. Non Linéaire , 4 (1987) pp. 203–230