Parovichenko algebra

Parovičenko algebra

Let be a Boolean algebra. If , then one says that provided that for all finite and one has . In addition, is said to be a Parovichenko algebra provided that it is both Cantor- and DuBois-Reymond-separable. This means that for all and such that there exists an element such that .

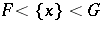The Parovichenko theorem from 1963 (see [a3]) asserts that under the continuum hypothesis (abbreviated CH), every Parovichenko algebra of size 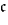(i.e., of the cardinality of the continuum, cf. also Continuum, cardinality of the) is isomorphic to the Boolean algebra 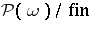, where is the power set algebra of 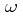and is its ideal of finite subsets. The theorem is proved by a transfinite induction with 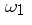many steps, where at each essential step of the process the separability properties of the Boolean algebras under consideration ensure that one can continue with the construction. This method goes back to W. Rudin [a7].

In 1978, E.K. van Douwen and J. van Mill proved the converse to Parovichenko's theorem in [a1]: If all Parovichenko algebras of size of the continuum are isomorphic, then the continuum hypothesis holds.

Parovichenko's theorem implies that every Boolean algebra of size at most that of the continuum can be embedded in under the continuum hypothesis. This result cannot be proved in ZFC alone (cf. also Set theory). In 1968, K. Kunen [a3] proved that in a model formed by adding 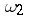Cohen reals to a model of the continuum hypothesis, there is no 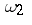sequence of subsets of 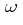which is strictly decreasing (modulo 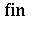). This implies that a Boolean algebra such as the clopen algebra of the ordinal space cannot be embedded in 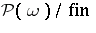. In 1978, E.K. van Douwen and T.C. Przymusiński [a2] used results of F. Rothberger [a6] to prove that there is a counterexample under the hypothesis

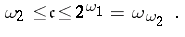This is interesting, since it only involves a hypothesis on cardinal numbers.

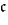Parovichenko's theorem has interesting consequences in topology. It implies, for example, that under the continuum hypothesis the Čech–Stone remainder of any zero-dimensional locally compact, -compact, non-compact space of weight at most is homeomorphic to , the Čech–Stone-remainder of 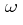with the discrete topology (cf. also Stone–Čech compactification; Topological structure (topology); Zero-dimensional space).

For more information, see e.g. [a4].

References

[a1]	E.K. van Douwen, J. van Mill, "Parovičenko's characterization of 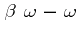implies CH" Proc. Amer. Math. Soc. , 72 (1978) pp. 539–541
[a2]	E.K. van Douwen, T.C. Przymusiński, "Separable extensions of first countable spaces" Fund. Math. , 105 (1980) pp. 147—158
[a3]	K. Kunen, "Inaccessibility properties of cardinals" PhD Thesis Stanford Univ. (1968)
[a4]	J. van Mill, "An introduction to " K. Kunen (ed.) J.E. Vaughan (ed.) , Handbook of Set–Theoretic Topology , North-Holland (1984) pp. 503–567
[a5]	I.I. Parovičenko, "A universal bicompact of weight 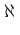" Soviet Math. Dokl. , 4 (1963) pp. 592–592
[a6]	F. Rothberger, "A remark on the existence of a denumberable base for a family of functions" Canad. J. Math. , 4 (1952) pp. 117–119
[a7]	W. Rudin, "Homogeneity problems in the theory of Čech compactifications" Duke Math. J. , 23 (1956) pp. 409–419