Pontryagin space
A Hilbert space with an indefinite metric $ \Pi _ \kappa $
that has a finite rank of indefiniteness $ \kappa $.
Basic facts concerning the geometry of these spaces were established by L.S. Pontryagin [1]. Besides the facts common for spaces with an indefinite metric, the following properties hold.
If $ {\mathcal P} $ is an arbitrary non-negative linear manifold in $ \Pi _ \kappa $, then $ \mathop{\rm dim} {\mathcal P} \leq \kappa $; if $ {\mathcal P} $ is a positive linear manifold and $ \mathop{\rm dim} {\mathcal P} = \kappa $, then its $ J $- orthogonal complement $ N $ is a negative linear manifold and $ \Pi _ \kappa = {\mathcal P} \oplus N $. Moreover, $ N $ is a complete space with respect to the norm $ | x | = \sqrt {- J ( x , x ) } $. If the linear manifold $ L \subset \Pi _ \kappa $ is non-degenerate, then its $ J $- orthogonal complement $ M $ is non-degenerate as well and $ \Pi _ \kappa = M \oplus L $.
The spectrum (in particular, the discrete spectrum) of a $ J $- unitary ( $ J $- self-adjoint) operator is symmetric with respect to the unit circle (real line), all elementary divisors corresponding to eigen values $ \lambda $, $ | \lambda | > 1 $, are of finite order $ \rho _ \lambda $, $ \rho _ \lambda \leq \kappa $, $ \rho _ \lambda = - \rho _ {\lambda ^ {-} 1 } $. The sum of the dimensions of the root subspaces of a $ J $- unitary ( $ J $- self-adjoint) operator corresponding to eigen values $ \lambda $, $ | \lambda | > 1 $( $ \mathop{\rm lm} \lambda > 0 $), does not exceed $ \kappa $.
The following theorem [1] is fundamental in the theory of $ J $- self-adjoint operators on a Pontryagin space $ \Pi _ \kappa $: For each $ J $- self-adjoint operator $ A $( $ \overline{ {D ( A) }}\; = \Pi _ \kappa $) there exists a $ \kappa $- dimensional (maximal) non-negative invariant subspace $ {\mathcal T} $ in which all eigen values of $ A $ have non-negative imaginary parts, and a $ \kappa $- dimensional non-negative invariant subspace $ {\mathcal T} ^ \prime $ in which all eigen values have non-positive imaginary parts. A similar statement in which the upper (lower) half-plane is replaced by the exterior (interior) of the unit disc is also valid for $ J $- unitary operators, and under certain additional conditions — even for operators on the space $ \Pi _ \infty $.
If $ U $ is a $ J $- unitary operator, then its maximal invariant subspaces $ {\mathcal T} $, $ {\mathcal T} ^ \prime $ can be chosen so that the elementary divisors of the operator $ U _ {\mathcal T} = U \mid _ {\mathcal T} $, $ U _ { {\mathcal T} ^ \prime } = U \mid _ { {\mathcal T} ^ \prime } $ are of minimal order. In order that a polynomial $ P ( \lambda ) $ with no roots inside the unit disc has the property: $ ( P ( U) x , P ( U) x ) \leq 0 $, $ x \in \Pi _ \kappa $, it is necessary and sufficient that it can be divided by the minimal annihilating polynomial of the operator $ U _ {\mathcal T} $. If $ U $ is a cyclic operator, then its non-negative invariant subspaces of dimension $ \kappa $ are uniquely determined. In this case the above-mentioned property of the polynomial $ P $ with roots $ \{ \lambda _ {i} \} $ outside the unit disc, $ | \lambda _ {i} | > 1 $, is equivalent to the divisibility of $ P ( \lambda ) $ by the characteristic polynomial of $ U _ {\mathcal T} $.
Each completely-continuous $ J $- self-adjoint operator $ A $ on a Pontryagin space $ \Pi _ \kappa $ such that zero belongs to its continuous spectrum does not have a residual spectrum. The root vectors of such an operator form a Riesz basis in $ \Pi _ \kappa $ with respect to the (definite) norm $ ( | J | x , x ) $.
Many facts concerning invariant subspaces and the spectrum can be generalized to a case of $ J $- isometric and $ J $- non-expanding operators. Thus, if $ \lambda _ {1} \dots \lambda _ {n} $ is an arbitrary set of eigen values of a $ J $- isometric operator, $ \lambda _ {i} \overline \lambda \; _ {k} \neq 1 $, $ i , k = 1 \dots n $, and if $ \rho _ {i} $ is the order of the elementary divisor at the point $ \lambda _ {i} $, then $ \sum _ {1} ^ {n} \rho _ {i} \leq \kappa $. Any $ J $- non-expanding boundedly-invertible operator $ T $ has a $ \kappa $- dimensional invariant non-negative subspace $ {\mathcal T} $ such that all eigen values of the restriction $ T \mid _ {\mathcal T} $ lie in the unit disc [2]. A similar fact holds for maximal $ J $- dissipative operators. In general, a $ J $- dissipative operator $ A $, $ D ( A) \subset D ( A ^ {*} ) $, has at most $ \kappa $ eigen values in the upper half-plane. $ J $- isometric and $ J $- symmetric (and more generally, $ J $- non-expanding and $ J $- dissipative) operators are related by the Cayley transformation (cf. Cayley transform), which has on $ \Pi _ \kappa $ all natural properties [2]. This fact allows one to develop the extension theory simultaneously for $ J $- isometric and $ J $- symmetric operators. In particular, every $ J $- isometric ( $ J $- symmetric) operator can be extended to a maximal one. If its deficiency indices are different, then it has no $ J $- unitary ( $ J $- self-adjoint) extensions. If these indices are equal and finite, then any maximal extension is $ J $- unitary ( $ J $- self-adjoint).
For completely-continuous operators on $ \Pi _ \kappa $, a number of statements on the completeness of the system of root vectors, analogous to the corresponding facts from the theory of dissipative operators on spaces with a definite metric, is valid.
References
| [1] | L.S. Pontryagin, "Hermitian operators in a space with indefinite metric" Izv. Akad. Nauk. SSSR Ser. Mat. , 8 (1944) pp. 243–280 (In Russian) |
| [2] | I.S. Iokhvidov, M.G. Krein, "Spectral theory of operators in a space with indefinite metric I" Transl. Amer. Math. Soc. (2) , 13 (1960) pp. 105–175 Trudy Moskov. Mat. Obshch. , 5 (1956) pp. 367–432 |
| [3] | I.S. Iokhvidov, M.G. Krein, "Spectral theory of operators in a space with indefinite metric II" Trudy Moskov. Mat. Obshch. , 8 (1959) pp. 413–496 (In Russian) |
| [4] | T.Ya. Azizov, I.S. Iokhvidov, "Linear operators in Hilbert spaces with 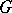 -metric" Russian Math. Surveys , 26 : 4 (1971) pp. 45–97 Uspekhi Mat. Nauk , 26 : 4 (1971) pp. 43–92 -metric" Russian Math. Surveys , 26 : 4 (1971) pp. 45–97 Uspekhi Mat. Nauk , 26 : 4 (1971) pp. 43–92 |
| [5] | M.G. Krein, "Introduction to the geometry of indefinite  -spaces and the theory of operators in these spaces" , Second Math. Summer School , 1 , Kiev (1965) pp. 15–92 (In Russian) -spaces and the theory of operators in these spaces" , Second Math. Summer School , 1 , Kiev (1965) pp. 15–92 (In Russian) |
| [6] | M.A. Naimark, R.S. Ismagilov, "Representations of groups and algebras in a space with indefinite metric" Itogi Nauk. i Tekhn. Mat. Anal. (1969) pp. 73–105 (In Russian) |
| [7] | L. Nagy, "State vector spaces with indefinite metric in quantum field theory" , Noordhoff (1966) |
Comments
Pontryagin spaces form a subclass of the class of Krein spaces (cf. Krein space and also Hilbert space with an indefinite metric). The operator $ J $ appearing in the beginning of the main article above is the fundamental symmetry (see Krein space), which defines the indefinite inner product via the formula $ [ x, y] = ( Jx, y) $.
References
| [a1] | T.Ya. Azizov, I.S. [I.S. Iokhvidov] Iohidov, "Linear operators in spaces with an indefinite metric" , Wiley (1989) (Translated from Russian) |
| [a2] | I.S. [I.S. Iokhvidov] Iohidov, M.G. Krein, H. Langer, "Introduction to the spectral theory of operators in spaces with an indefinite metric" , Akademie Verlag (1982) |
Pontryagin space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pontryagin_space&oldid=11492