Measurable flow
in a measure space 
2020 Mathematics Subject Classification: Primary: 37A10 [MSN][ZBL]
A family 
(
runs over the set of real numbers 
) of automorphisms of the space such that: 1) 
for all 
, 
; and 2) the mapping 
taking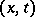 
to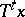 
is measurable (a measure is introduced on 
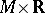as the direct product of the measure 
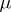in 
and the Lebesgue measure in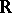 
). "Automorphisms" here are to be understood in the strict sense of the word (and not modulo 0), that is, the 
must be bijections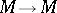 
carrying measurable sets to measurable sets of the same measure. In using automorphisms modulo 0, it turns out to be expedient to replace condition 2) by a condition of a different character, which leads to the concept of a continuous flow. Measurable flows are used in ergodic theory.
Measurable flow. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Measurable_flow&oldid=11332