Difference between revisions of "Quasi-discrete spectrum"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
m (fixing spaces) |
||
| Line 14: | Line 14: | ||
In ergodic theory, the concept "transformation with a quasi-discrete spectrum" is in fact considered only in connection with an ergodic automorphism $ T $ | In ergodic theory, the concept "transformation with a quasi-discrete spectrum" is in fact considered only in connection with an ergodic automorphism $ T $ | ||
| − | of a [[Lebesgue space|Lebesgue space]] $ ( X , \mu ) $( | + | of a [[Lebesgue space|Lebesgue space]] $ ( X , \mu ) $ (although the definition given below is also formally suitable in a more general situation). For $ T $ |
| − | although the definition given below is also formally suitable in a more general situation). For $ T $ | ||
one defines by induction quasi-eigen functions and quasi-eigen values of order $ n $. | one defines by induction quasi-eigen functions and quasi-eigen values of order $ n $. | ||
A quasi-eigen function of order one is an ordinary eigen function corresponding to the shift operator | A quasi-eigen function of order one is an ordinary eigen function corresponding to the shift operator | ||
| Line 24: | Line 23: | ||
that is, a non-zero $ f \in L _ {2} ( X , \mu ) $ | that is, a non-zero $ f \in L _ {2} ( X , \mu ) $ | ||
| − | for which $ f ( T x ) = \lambda f ( x) $( | + | for which $ f ( T x ) = \lambda f ( x) $ (almost-everywhere, below this is also assumed to hold), where $ \lambda $ |
| − | almost-everywhere, below this is also assumed to hold), where $ \lambda $ | ||
is a constant (an eigen value; it is a quasi-eigen value of order one). If $ f \in L _ {2} ( X , \mu ) $, | is a constant (an eigen value; it is a quasi-eigen value of order one). If $ f \in L _ {2} ( X , \mu ) $, | ||
$ f \neq 0 $ | $ f \neq 0 $ | ||
| Line 53: | Line 51: | ||
of a compactum $ X $ | of a compactum $ X $ | ||
that is assumed to be completely minimal (that is, all its powers are minimal). The quasi-eigen functions and values are defined in the same way as above by replacing $ L _ {2} ( X , \mu ) $ | that is assumed to be completely minimal (that is, all its powers are minimal). The quasi-eigen functions and values are defined in the same way as above by replacing $ L _ {2} ( X , \mu ) $ | ||
| − | by $ C ( X) $( | + | by $ C ( X) $ (the continuous complex-valued functions). $ T $ |
| − | the continuous complex-valued functions). $ T $ | ||
has a quasi-discrete spectrum if the quasi-eigen functions separate the points of $ X $. | has a quasi-discrete spectrum if the quasi-eigen functions separate the points of $ X $. | ||
The topological version of the theory is by and large analogous to the metric one (see [[#References|[5]]]–[[#References|[7]]]). However, in passing from the topological theory of cascades to that of flows with quasi-discrete spectra, essentially different arguments are required and it is even necessary, to some extent, to go outside the framework of the ordinary concepts of topological dynamics (it turns out to be expedient to consider flows $ \{ T _ {t} \} $ | The topological version of the theory is by and large analogous to the metric one (see [[#References|[5]]]–[[#References|[7]]]). However, in passing from the topological theory of cascades to that of flows with quasi-discrete spectra, essentially different arguments are required and it is even necessary, to some extent, to go outside the framework of the ordinary concepts of topological dynamics (it turns out to be expedient to consider flows $ \{ T _ {t} \} $ | ||
Latest revision as of 05:56, 19 March 2022
A term in ergodic theory and topological dynamics used in phrases like: "a dynamical system (flow, cascade or transformation generating the latter) has a quasi-discrete spectrum (or is a system, flow, etc. with a quasi-discrete spectrum)" .
In ergodic theory, the concept "transformation with a quasi-discrete spectrum" is in fact considered only in connection with an ergodic automorphism $ T $ of a Lebesgue space $ ( X , \mu ) $ (although the definition given below is also formally suitable in a more general situation). For $ T $ one defines by induction quasi-eigen functions and quasi-eigen values of order $ n $. A quasi-eigen function of order one is an ordinary eigen function corresponding to the shift operator
$$ U _ {T} : f ( x) \mapsto f ( T x ) \ \mathop{\rm in} L _ {2} ( X , \mu ) , $$
that is, a non-zero $ f \in L _ {2} ( X , \mu ) $ for which $ f ( T x ) = \lambda f ( x) $ (almost-everywhere, below this is also assumed to hold), where $ \lambda $ is a constant (an eigen value; it is a quasi-eigen value of order one). If $ f \in L _ {2} ( X , \mu ) $, $ f \neq 0 $ and $ f ( T x ) = \phi ( x) f ( x) $, where $ \phi $ is a quasi-eigen function of order $ n $, then $ f $ is called a quasi-eigen function of order $ n+ 1 $, and $ \phi $ is the corresponding quasi-eigen value (of the same order). (In [2] instead of quasi-eigen functions and values one speaks of generalized eigen functions and generalized eigen values.) It follows from the ergodicity of $ T $ that $ | f ( x) | = \textrm{ const } $ for any quasi-eigen function $ f $, while if $ f $ is also a quasi-eigen value, then $ | f ( x) | = 1 $. For this reason one often includes in the definition of a quasi-eigen function the normalization condition $ | f ( x) | = 1 $. One says that $ T $ has a quasi-discrete spectrum if the quasi-eigen functions of all possible orders form a complete system in $ L _ {2} ( X , \mu ) $. There are complete results for the case when in addition the automorphism $ T $ is completely ergodic (that is, all its powers are ergodic). There is a complete metric classification of such automorphisms $ T $, and their properties are well known [3]. The concept of a quasi-discrete spectrum and the theory related to it can be generalized for locally compact commutative groups of transformations of Lebesgue spaces (in particular, for a measurable flow); see the account of T. Wieting's results in [4].
Although "quasi-discrete spectrum" at first sight suggests that one is dealing with a certain type of spectrum of a dynamical system, in fact the property of a cascade $ \{ T ^ {n} \} $ of having a quasi-discrete spectrum is not a spectral one, that is, it cannot be expressed in terms of the spectral properties of the shift operator $ U _ {T} $. Even if it is known that a cascade has a quasi-discrete spectrum, the spectrum still does not uniquely determine its metric properties. The quasi-discrete spectrum was introduced just in connection with the first example of metrically non-isomorphic ergodic cascades with the same spectrum ([1], see also [2]). In this example the cascades have quasi-discrete spectra but in one of them there are quasi-eigen functions of third order, while in the other there are not.
In topological dynamics the notion of a quasi-discrete spectrum was introduced for a homeomorphism $ T $ of a compactum $ X $ that is assumed to be completely minimal (that is, all its powers are minimal). The quasi-eigen functions and values are defined in the same way as above by replacing $ L _ {2} ( X , \mu ) $ by $ C ( X) $ (the continuous complex-valued functions). $ T $ has a quasi-discrete spectrum if the quasi-eigen functions separate the points of $ X $. The topological version of the theory is by and large analogous to the metric one (see [5]–[7]). However, in passing from the topological theory of cascades to that of flows with quasi-discrete spectra, essentially different arguments are required and it is even necessary, to some extent, to go outside the framework of the ordinary concepts of topological dynamics (it turns out to be expedient to consider flows $ \{ T _ {t} \} $ with discontinuous dependence of the homeomorphisms $ T _ {t} $ on $ t $) (see [8], [9]).
References
| [1] | P.R. Halmos, J. von Neumann, "Operator methods in classical mechanics" Ann. of Math. , 43 : 2 (1942) pp. 332–350 |
| [2] | P.R. Halmos, "Lectures on ergodic theory" , Math. Soc. Japan (1956) |
| [3] | L.M. Abramov, "Metric automorphisms with quasi-discrete spectrum" Transl. Amer. Math. Soc. , 39 (1964) pp. 37–56 Izv. Akad. Nauk SSSR. Ser. Mat. , 26 : 4 (1962) pp. 513–530 |
| [4] | R.J. Zimmer, "Ergodic actions with generalized spectrum" Illinois J. Math. , 20 (1976) pp. 555–588 |
| [5] | F. Hahn, W. Parry, "Minimal dynamical systems with quasi-discrete spectrum" J. London Math. Soc. , 40 : 2 (1965) pp. 309–323 |
| [6] | F. Hahn, W. Parry, "Some characteristic properties of dynamical systems with quasi-discrete spectra" Math. Systems Theory , 2 : 2 (1968) pp. 179–190 |
| [7] | J.R. Brown, "Ergodic theory and topological dynamics" , Acad. Press (1976) |
| [8] | F. Hahn, "Discrete real time flows with quasi-discrete spectra and algebras generated by 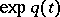 " Israel J. Math. , 16 : 1 (1973) pp. 20–37 " Israel J. Math. , 16 : 1 (1973) pp. 20–37 |
| [9] | W. Parry, "Notes on a posthumous paper by F. Hahn" Israel J. Math. , 16 : 1 (1973) pp. 38–45 |
Quasi-discrete spectrum. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quasi-discrete_spectrum&oldid=52225