Difference between revisions of "Tate conjectures"
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | t0922501.png | ||
| + | $#A+1 = 43 n = 2 | ||
| + | $#C+1 = 43 : ~/encyclopedia/old_files/data/T092/T.0902250 Tate conjectures | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
Conjectures expressed by J. Tate (see [[#References|[1]]]) and describing relations between Diophantine and algebro-geometric properties of an [[Algebraic variety|algebraic variety]]. | Conjectures expressed by J. Tate (see [[#References|[1]]]) and describing relations between Diophantine and algebro-geometric properties of an [[Algebraic variety|algebraic variety]]. | ||
| − | Conjecture 1. If the field | + | Conjecture 1. If the field $ k $ |
| + | is finitely generated over its prime subfield, if $ V $ | ||
| + | is a smooth projective variety over $ k $, | ||
| + | if $ l $ | ||
| + | is a prime number different from the characteristic of the field $ k $, | ||
| + | if | ||
| − | + | $$ | |
| + | \rho _ {l} ^ {(} i) : \ | ||
| + | \mathop{\rm Gal} ( \widetilde{k} /k) \rightarrow \ | ||
| + | \mathop{\rm Aut} _ {\mathbf Q _ {l} } H _ {l} ^ {2i} | ||
| + | ( V \otimes _ {k} \overline{k}\; ) ( i) | ||
| + | $$ | ||
| − | is the natural | + | is the natural $ l $- |
| + | adic representation, and $ g _ {l} ^ {(} i) = \mathop{\rm Lie} ( \mathop{\rm Im} ( \rho _ {l} ^ {(} i) )) $, | ||
| + | then the $ \mathbf Q _ {l} $- | ||
| + | space $ [ H _ {l} ^ {2i} ( V \otimes _ {k} \overline{k}\; ) ( i) ] ^ {g _ {l} ^ {(} i) } $, | ||
| + | the space of elements of $ H _ {l} ^ {2i} ( V \otimes _ {k} \overline{k}\; ) ( i) $ | ||
| + | annihilated by $ g _ {l} ^ {(} i) $, | ||
| + | is generated by the homology classes of algebraic cycles of codimension $ i $ | ||
| + | on $ V \otimes _ {k} \overline{k}\; $( | ||
| + | cf. also [[Algebraic cycle|Algebraic cycle]]). | ||
| − | Conjecture 2. The rank of the group of classes of algebraic cycles of codimension | + | Conjecture 2. The rank of the group of classes of algebraic cycles of codimension $ i $ |
| + | on $ V $ | ||
| + | modulo homology equivalence coincides with the order of the pole of the function $ L _ {2i} ( V, s) $ | ||
| + | at the point $ s = \mathop{\rm dim} Y + i $. | ||
| − | These conjectures were verified for a large number of particular cases; restrictions are imposed both on the field | + | These conjectures were verified for a large number of particular cases; restrictions are imposed both on the field $ k $ |
| + | and on the variety $ V $. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> J.T. Tate, "Algebraic cycles and poles of zeta-functions" D.F.G. Schilling (ed.) , ''Arithmetical Algebraic geometry (Proc. Purdue Conf. 1963)'' , Harper & Row (1965) pp. 93–110 {{MR|0225778}} {{ZBL|0213.22804}} </TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> J.T. Tate, "Algebraic cycles and poles of zeta-functions" D.F.G. Schilling (ed.) , ''Arithmetical Algebraic geometry (Proc. Purdue Conf. 1963)'' , Harper & Row (1965) pp. 93–110 {{MR|0225778}} {{ZBL|0213.22804}} </TD></TR></table> | ||
| + | ====Comments==== | ||
| + | In conjecture 2 above $ L _ {i} ( V, s ) $ | ||
| + | is the $ L $- | ||
| + | series of $ V $, | ||
| + | defined by | ||
| + | $$ | ||
| + | L _ {i} ( V, s) = \prod _ {\mathfrak p } | ||
| + | \{ P _ {i} ( q ^ {-} s ) \} ^ {-} 1 , | ||
| + | $$ | ||
| − | + | where the product is over all primes $ \mathfrak p $ | |
| − | + | where $ V $ | |
| − | + | has good reduction and where $ P _ {i} ( q ^ {-} s ) $ | |
| − | + | is the $ i $- | |
| + | th polynomial factor appearing in the [[Zeta-function|zeta-function]] of the variety $ V \mathop{\rm mod} \mathfrak p $ | ||
| + | over the residue field $ \mathbf F _ {q} $ | ||
| + | of $ k $ | ||
| + | at $ \mathfrak p $, | ||
| − | + | $$ | |
| + | \zeta _ {V \mathop{\rm mod} \mathfrak p } ( s) = \ | ||
| − | + | \frac{P _ {1} ( q ^ {-} s ) \dots P _ {2d-} 1 ( q ^ {-} s ) }{P _ {0} ( q ^ {-} s ) \dots P _ {2d} ( q ^ {-} s ) } | |
| + | . | ||
| + | $$ | ||
| − | In the case | + | In the case $ V = A \times \widehat{B} $, |
| + | with $ A $ | ||
| + | and $ B $ | ||
| + | Abelian varieties, conjecture 1 takes for $ i = 1 $( | ||
| + | i.e. for divisors) the following form: The natural homomorphism | ||
| − | + | $$ | |
| + | \mathop{\rm Hom} _ {k} ( A, B) \otimes \mathbf Z _ {l} \rightarrow \ | ||
| + | \mathop{\rm Hom} _ { \mathop{\rm Gal} ( \overline{k}\; / k ) } | ||
| + | ( T _ {l} ( A), T _ {l} ( B) ) | ||
| + | $$ | ||
| − | is an isomorphism (where | + | is an isomorphism (where $ T _ {l} (-) $ |
| + | is the [[Tate module|Tate module]] of the Abelian variety) (see [[#References|[1]]]). This case of the conjecture has been proved: i) $ k $ | ||
| + | is a finite field by J. Tate [[#References|[a1]]]; ii) if $ k $ | ||
| + | is a function field over a finite field by J.G. Zarkin [[#References|[a2]]]; and iii) if $ k $ | ||
| + | is a number field by G. Faltings [[#References|[a3]]]. | ||
| − | For examples of particular cases where the Tate conjecture has been proved see, e.g., [[#References|[a4]]] for ordinary | + | For examples of particular cases where the Tate conjecture has been proved see, e.g., [[#References|[a4]]] for ordinary $ K3 $- |
| + | surfaces over finite fields and [[#References|[a5]]] for Hilbert modular surfaces. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Tate, "Endomorphisms of Abelian varieties over finite fields" ''Invent. Math.'' , '''2''' (1966) pp. 104–145 {{MR|0206004}} {{ZBL|0147.20303}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.G. Zarking, "A remark on endomorphisms of Abelian varieties over function fields of finite characteristic" ''Math. USSR Izv.'' , '''8''' (1974) pp. 477–480 ''Izv. Akad. Nauk SSSR'' , '''38''' : 3 (1974) pp. 471–474</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> G. Faltings, "Endlichkeitssätze für abelsche Varietäten über Zahlkörpern" ''Invent. Math.'' , '''73''' (1983) pp. 349–366 (Erratum: Invent. Math <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092250/t09225045.png" /> (1984), 381) {{MR|0718935}} {{MR|0732554}} {{ZBL|0588.14026}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> N.O. Nygaard, "The Tate conjecture for ordinary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092250/t09225046.png" />-surfaces over finite fields" ''Invent. Math.'' , '''74''' (1983) pp. 213–237 {{MR|723215}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> G. van der Geer, "Hilbert modular surfaces" , Springer (1987) {{MR|}} {{ZBL|0634.14022}} {{ZBL|0511.14021}} {{ZBL|0483.14009}} {{ZBL|0418.14021}} {{ZBL|0349.14022}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> G. Wüstholz (ed.) , ''Rational points'' , Vieweg (1984) {{MR|0766568}} {{ZBL|0588.14027}} </TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Tate, "Endomorphisms of Abelian varieties over finite fields" ''Invent. Math.'' , '''2''' (1966) pp. 104–145 {{MR|0206004}} {{ZBL|0147.20303}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J.G. Zarking, "A remark on endomorphisms of Abelian varieties over function fields of finite characteristic" ''Math. USSR Izv.'' , '''8''' (1974) pp. 477–480 ''Izv. Akad. Nauk SSSR'' , '''38''' : 3 (1974) pp. 471–474</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> G. Faltings, "Endlichkeitssätze für abelsche Varietäten über Zahlkörpern" ''Invent. Math.'' , '''73''' (1983) pp. 349–366 (Erratum: Invent. Math <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092250/t09225045.png" /> (1984), 381) {{MR|0718935}} {{MR|0732554}} {{ZBL|0588.14026}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> N.O. Nygaard, "The Tate conjecture for ordinary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092250/t09225046.png" />-surfaces over finite fields" ''Invent. Math.'' , '''74''' (1983) pp. 213–237 {{MR|723215}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> G. van der Geer, "Hilbert modular surfaces" , Springer (1987) {{MR|}} {{ZBL|0634.14022}} {{ZBL|0511.14021}} {{ZBL|0483.14009}} {{ZBL|0418.14021}} {{ZBL|0349.14022}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> G. Wüstholz (ed.) , ''Rational points'' , Vieweg (1984) {{MR|0766568}} {{ZBL|0588.14027}} </TD></TR></table> | ||
Revision as of 08:25, 6 June 2020
Conjectures expressed by J. Tate (see [1]) and describing relations between Diophantine and algebro-geometric properties of an algebraic variety.
Conjecture 1. If the field $ k $ is finitely generated over its prime subfield, if $ V $ is a smooth projective variety over $ k $, if $ l $ is a prime number different from the characteristic of the field $ k $, if
$$ \rho _ {l} ^ {(} i) : \ \mathop{\rm Gal} ( \widetilde{k} /k) \rightarrow \ \mathop{\rm Aut} _ {\mathbf Q _ {l} } H _ {l} ^ {2i} ( V \otimes _ {k} \overline{k}\; ) ( i) $$
is the natural $ l $- adic representation, and $ g _ {l} ^ {(} i) = \mathop{\rm Lie} ( \mathop{\rm Im} ( \rho _ {l} ^ {(} i) )) $, then the $ \mathbf Q _ {l} $- space $ [ H _ {l} ^ {2i} ( V \otimes _ {k} \overline{k}\; ) ( i) ] ^ {g _ {l} ^ {(} i) } $, the space of elements of $ H _ {l} ^ {2i} ( V \otimes _ {k} \overline{k}\; ) ( i) $ annihilated by $ g _ {l} ^ {(} i) $, is generated by the homology classes of algebraic cycles of codimension $ i $ on $ V \otimes _ {k} \overline{k}\; $( cf. also Algebraic cycle).
Conjecture 2. The rank of the group of classes of algebraic cycles of codimension $ i $ on $ V $ modulo homology equivalence coincides with the order of the pole of the function $ L _ {2i} ( V, s) $ at the point $ s = \mathop{\rm dim} Y + i $.
These conjectures were verified for a large number of particular cases; restrictions are imposed both on the field $ k $ and on the variety $ V $.
References
| [1] | J.T. Tate, "Algebraic cycles and poles of zeta-functions" D.F.G. Schilling (ed.) , Arithmetical Algebraic geometry (Proc. Purdue Conf. 1963) , Harper & Row (1965) pp. 93–110 MR0225778 Zbl 0213.22804 |
Comments
In conjecture 2 above $ L _ {i} ( V, s ) $ is the $ L $- series of $ V $, defined by
$$ L _ {i} ( V, s) = \prod _ {\mathfrak p } \{ P _ {i} ( q ^ {-} s ) \} ^ {-} 1 , $$
where the product is over all primes $ \mathfrak p $ where $ V $ has good reduction and where $ P _ {i} ( q ^ {-} s ) $ is the $ i $- th polynomial factor appearing in the zeta-function of the variety $ V \mathop{\rm mod} \mathfrak p $ over the residue field $ \mathbf F _ {q} $ of $ k $ at $ \mathfrak p $,
$$ \zeta _ {V \mathop{\rm mod} \mathfrak p } ( s) = \ \frac{P _ {1} ( q ^ {-} s ) \dots P _ {2d-} 1 ( q ^ {-} s ) }{P _ {0} ( q ^ {-} s ) \dots P _ {2d} ( q ^ {-} s ) } . $$
In the case $ V = A \times \widehat{B} $, with $ A $ and $ B $ Abelian varieties, conjecture 1 takes for $ i = 1 $( i.e. for divisors) the following form: The natural homomorphism
$$ \mathop{\rm Hom} _ {k} ( A, B) \otimes \mathbf Z _ {l} \rightarrow \ \mathop{\rm Hom} _ { \mathop{\rm Gal} ( \overline{k}\; / k ) } ( T _ {l} ( A), T _ {l} ( B) ) $$
is an isomorphism (where $ T _ {l} (-) $ is the Tate module of the Abelian variety) (see [1]). This case of the conjecture has been proved: i) $ k $ is a finite field by J. Tate [a1]; ii) if $ k $ is a function field over a finite field by J.G. Zarkin [a2]; and iii) if $ k $ is a number field by G. Faltings [a3].
For examples of particular cases where the Tate conjecture has been proved see, e.g., [a4] for ordinary $ K3 $- surfaces over finite fields and [a5] for Hilbert modular surfaces.
References
| [a1] | J. Tate, "Endomorphisms of Abelian varieties over finite fields" Invent. Math. , 2 (1966) pp. 104–145 MR0206004 Zbl 0147.20303 |
| [a2] | J.G. Zarking, "A remark on endomorphisms of Abelian varieties over function fields of finite characteristic" Math. USSR Izv. , 8 (1974) pp. 477–480 Izv. Akad. Nauk SSSR , 38 : 3 (1974) pp. 471–474 |
| [a3] | G. Faltings, "Endlichkeitssätze für abelsche Varietäten über Zahlkörpern" Invent. Math. , 73 (1983) pp. 349–366 (Erratum: Invent. Math 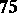 (1984), 381) MR0718935 MR0732554 Zbl 0588.14026 (1984), 381) MR0718935 MR0732554 Zbl 0588.14026 |
| [a4] | N.O. Nygaard, "The Tate conjecture for ordinary 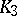 -surfaces over finite fields" Invent. Math. , 74 (1983) pp. 213–237 MR723215 -surfaces over finite fields" Invent. Math. , 74 (1983) pp. 213–237 MR723215 |
| [a5] | G. van der Geer, "Hilbert modular surfaces" , Springer (1987) Zbl 0634.14022 Zbl 0511.14021 Zbl 0483.14009 Zbl 0418.14021 Zbl 0349.14022 |
| [a6] | G. Wüstholz (ed.) , Rational points , Vieweg (1984) MR0766568 Zbl 0588.14027 |
Tate conjectures. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tate_conjectures&oldid=48950