Difference between revisions of "Lavrent'ev theorem"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| − | + | <!-- | |
| + | l0577001.png | ||
| + | $#A+1 = 33 n = 1 | ||
| + | $#C+1 = 33 : ~/encyclopedia/old_files/data/L057/L.0507700 Lavrent\AApev theorem | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| − | + | {{TEX|auto}} | |
| + | {{TEX|done}} | ||
| − | Lavrent'ev's theorem in | + | Lavrent'ev's theorem in descriptive set theory: A topological mapping between two sets in $ \mathbf R ^ {n} $ |
| + | can be extended to a [[Homeomorphism|homeomorphism]] between certain sets of type $ G _ \delta $ | ||
| + | containing them. A consequence of this theorem is that the Hausdorff type of a set is a topological invariant (see ). | ||
| − | + | Lavrent'ev's theorem in approximation theory gives a criterion for the possibility of uniform approximation: In order that a function, continuous on a compact set $ K \subset \mathbf C $, | |
| + | can be uniformly approximated on $ K $ | ||
| + | by polynomials it is necessary and sufficient that $ K $ | ||
| + | is a compact set without interior points that does not partition the complex plane (see ). | ||
| − | + | Lavrent'ev's theorem in the theory of quasi-conformal mapping: Let $ D _ {z} $ | |
| + | and $ D _ {w} $ | ||
| + | be two simply-connected domains in the plane bounded by piecewise-smooth curves and let $ z _ {1} , z _ {2} , z _ {3} $ | ||
| + | and $ w _ {1} , w _ {2} , w _ {3} $ | ||
| + | be triples of positively enumerated points on their boundaries. Then for any strongly-elliptic system of equations | ||
| − | with uniformly continuous partial derivatives of the functions that specify the equations of the characteristics, there is always a unique homeomorphic mapping of | + | $$ |
| + | \Phi _ {1} ( x , y , u , v , u _ {x} ^ \prime ,\ | ||
| + | u _ {y} ^ \prime , v _ {x} ^ \prime , v _ {y} ^ \prime ) = 0 , | ||
| + | $$ | ||
| + | |||
| + | $$ | ||
| + | \Phi _ {2} ( x , y , u , v , u _ {x} ^ \prime , u _ {y} ^ \prime , v _ {x} ^ \prime , v _ {y} ^ \prime ) = 0 , | ||
| + | $$ | ||
| + | |||
| + | with uniformly continuous partial derivatives of the functions that specify the equations of the characteristics, there is always a unique homeomorphic mapping of $ D _ {z} $ | ||
| + | onto $ D _ {w} $ | ||
| + | that realizes a solution $ u ( x , y ) $, | ||
| + | $ v ( x , y ) $ | ||
| + | of the system, and under which the given triples of boundary points correspond to each other. | ||
For Lavrent'ev's theorem in mechanics (aerofoil theory, solitary wave, forms of dynamical loss of stability, flows, the theory of cumulative charge, directed detonation) see [[#References|[4]]]. | For Lavrent'ev's theorem in mechanics (aerofoil theory, solitary wave, forms of dynamical loss of stability, flows, the theory of cumulative charge, directed detonation) see [[#References|[4]]]. | ||
| Line 17: | Line 47: | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> M. [M.A. Lavrent'ev] Lavrentieff, "Contribution à la théorie des ensembles homéomorphes" ''Fund. Math.'' , '''6''' (1924) pp. 149–160</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> M.A. Lavrent'ev, ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''12''' : 6 (1948) pp. 513–554</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M.A. Lavrent'ev, "On the theory of conformal mapping" ''Tr. Fiz.-Mat. Inst. Akad. Nauk SSSR, Otdel. Mat.'' , '''5''' (1934) pp. 195–245 (In Russian)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> "Mikhail Alekseevich Lavrent'ev" , ''Bibliography of Soviet Scientists, Mathematics Series'' , '''12''' , Acad. Sci. USSR , Moscow (1971) (In Russian)</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> M. [M.A. Lavrent'ev] Lavrentieff, "Contribution à la théorie des ensembles homéomorphes" ''Fund. Math.'' , '''6''' (1924) pp. 149–160</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> M.A. Lavrent'ev, ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''12''' : 6 (1948) pp. 513–554</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M.A. Lavrent'ev, "On the theory of conformal mapping" ''Tr. Fiz.-Mat. Inst. Akad. Nauk SSSR, Otdel. Mat.'' , '''5''' (1934) pp. 195–245 (In Russian)</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> "Mikhail Alekseevich Lavrent'ev" , ''Bibliography of Soviet Scientists, Mathematics Series'' , '''12''' , Acad. Sci. USSR , Moscow (1971) (In Russian)</TD></TR></table> | ||
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
| − | + | 1) This theorem is valid in the following more general situation: If $ X $ | |
| − | 1) This theorem is valid in the following more general situation: If | + | and $ Y $ |
| + | are completely-metrizable spaces, $ A \subseteq X, B \subseteq Y $, | ||
| + | and $ f : A \rightarrow B $ | ||
| + | is a homeomorphism, then there are $ G _ \delta $ | ||
| + | sets (cf. [[Set of type F sigma(G delta)|Set of type $ F _ \sigma $( | ||
| + | $ G _ \delta $)]]) | ||
| + | $ A ^ {*} \subseteq X $ | ||
| + | and $ B ^ {*} \subseteq Y $ | ||
| + | with $ A \subseteq A ^ {*} $, | ||
| + | $ B \subseteq B ^ {*} $ | ||
| + | and a homeomorphism $ f ^ { * } : A ^ {*} \rightarrow B ^ {*} $ | ||
| + | extending $ f $. | ||
This theorem has proved to be very useful in extension theory and in the construction of counterexamples. | This theorem has proved to be very useful in extension theory and in the construction of counterexamples. | ||
| Line 33: | Line 72: | ||
Consider the following two optimization problems: | Consider the following two optimization problems: | ||
| − | + | $$ \tag{a1 } | |
| + | \left . | ||
| + | \begin{array}{c} | ||
| + | \inf \int\limits _ { 0 } ^ { T } L ( t , x , \dot{x} ) dt , \\ | ||
| + | x ( 0) = \xi _ {0} ,\ x ( T) = \xi _ {1} ,\ \ | ||
| + | x \in L _ {1} ; \\ | ||
| + | \end{array} | ||
| + | \right \} | ||
| + | $$ | ||
| − | + | $$ \tag{a2 } | |
| + | \left . | ||
| + | \begin{array}{c} | ||
| + | \inf \int\limits _ { 0 } ^ { T } L ( t , x , \dot{x} ) d t , \\ | ||
| + | x ( 0) = \xi _ {0} ,\ x ( T) = \ | ||
| + | \xi _ {1} ,\ x \in C ^ {1} . \\ | ||
| + | \end{array} | ||
| + | \right \} | ||
| + | $$ | ||
| − | I.e. | + | I.e. $ x $ |
| + | is supposed to be absolutely continuous in (a1) and continuously differentiable in (a2), Then it may happen that $ \inf ( A _ {1} ) < \inf ( A _ {2} ) $. | ||
| + | This is known as the Lavrent'ev phenomenon. As a consequence, the minimizer for (a1) will not satisfy the [[Euler–Lagrange equation|Euler–Lagrange equation]] even though $ L $ | ||
| + | is smooth. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Aarts, "Completeness degree, a generalization of dimension" ''Fund. Math.'' , '''63''' (1968) pp. 28–41</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> T.A. Chapman, "Dense <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057700/l05770034.png" />-compact subsets of infinite-dimensional manifolds" ''Trans. Amer. Math. Soc.'' , '''154''' (1971) pp. 399–426</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E.K. van Douwen, "A compact space with a measure that knows which sets are homeomorphic" ''Adv. Math.'' , '''52''' (1984) pp. 1–33</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R. Engelking, "General topology" , Heldermann (1989)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> J. van Mill, "Domain invariance in infinite-dimensional linear spaces" ''Proc. Amer. Math. Soc.'' , '''101''' (1987) pp. 173–180</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S.N. Mergelyan, "On a theorem of M.A. Lavrent'ev" ''Transl. Amer. Math. Soc.'' , '''3''' (1962) pp. 281–286 ''Dokl. Akad. Nauk SSSR'' , '''77''' (1951) pp. 565–568</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> S.N. Mergelyan, "On the representation of functions by series of polynomials on closed sets" ''Transl. Amer. Math. Soc.'' , '''3''' (1962) pp. 287–293 ''Dokl. Akad. Nauk SSSR'' , '''78''' (1951) pp. 405–408</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> L. Cesari, "Optimization - Theory and applications" , Springer (1983) pp. Sect. 18.5</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> M. [M.A. Lavrent'ev] Lavrentiev, "Sur quelques problèmes du calcul des variations" ''Ann. Mat. Pura Appl.'' , '''4''' (1926) pp. 7–28</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 278ff</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> M.A. Lavrent'ev, "Variational methods for boundary value problems for systems of elliptic equations" , Noordhoff (1963) (Translated from Russian)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Aarts, "Completeness degree, a generalization of dimension" ''Fund. Math.'' , '''63''' (1968) pp. 28–41</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> T.A. Chapman, "Dense <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057700/l05770034.png" />-compact subsets of infinite-dimensional manifolds" ''Trans. Amer. Math. Soc.'' , '''154''' (1971) pp. 399–426</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> E.K. van Douwen, "A compact space with a measure that knows which sets are homeomorphic" ''Adv. Math.'' , '''52''' (1984) pp. 1–33</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R. Engelking, "General topology" , Heldermann (1989)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> J. van Mill, "Domain invariance in infinite-dimensional linear spaces" ''Proc. Amer. Math. Soc.'' , '''101''' (1987) pp. 173–180</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> S.N. Mergelyan, "On a theorem of M.A. Lavrent'ev" ''Transl. Amer. Math. Soc.'' , '''3''' (1962) pp. 281–286 ''Dokl. Akad. Nauk SSSR'' , '''77''' (1951) pp. 565–568</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> S.N. Mergelyan, "On the representation of functions by series of polynomials on closed sets" ''Transl. Amer. Math. Soc.'' , '''3''' (1962) pp. 287–293 ''Dokl. Akad. Nauk SSSR'' , '''78''' (1951) pp. 405–408</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> L. Cesari, "Optimization - Theory and applications" , Springer (1983) pp. Sect. 18.5</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> M. [M.A. Lavrent'ev] Lavrentiev, "Sur quelques problèmes du calcul des variations" ''Ann. Mat. Pura Appl.'' , '''4''' (1926) pp. 7–28</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 278ff</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> M.A. Lavrent'ev, "Variational methods for boundary value problems for systems of elliptic equations" , Noordhoff (1963) (Translated from Russian)</TD></TR></table> | ||
Revision as of 22:15, 5 June 2020
Lavrent'ev's theorem in descriptive set theory: A topological mapping between two sets in $ \mathbf R ^ {n} $
can be extended to a homeomorphism between certain sets of type $ G _ \delta $
containing them. A consequence of this theorem is that the Hausdorff type of a set is a topological invariant (see ).
Lavrent'ev's theorem in approximation theory gives a criterion for the possibility of uniform approximation: In order that a function, continuous on a compact set $ K \subset \mathbf C $, can be uniformly approximated on $ K $ by polynomials it is necessary and sufficient that $ K $ is a compact set without interior points that does not partition the complex plane (see ).
Lavrent'ev's theorem in the theory of quasi-conformal mapping: Let $ D _ {z} $ and $ D _ {w} $ be two simply-connected domains in the plane bounded by piecewise-smooth curves and let $ z _ {1} , z _ {2} , z _ {3} $ and $ w _ {1} , w _ {2} , w _ {3} $ be triples of positively enumerated points on their boundaries. Then for any strongly-elliptic system of equations
$$ \Phi _ {1} ( x , y , u , v , u _ {x} ^ \prime ,\ u _ {y} ^ \prime , v _ {x} ^ \prime , v _ {y} ^ \prime ) = 0 , $$
$$ \Phi _ {2} ( x , y , u , v , u _ {x} ^ \prime , u _ {y} ^ \prime , v _ {x} ^ \prime , v _ {y} ^ \prime ) = 0 , $$
with uniformly continuous partial derivatives of the functions that specify the equations of the characteristics, there is always a unique homeomorphic mapping of $ D _ {z} $ onto $ D _ {w} $ that realizes a solution $ u ( x , y ) $, $ v ( x , y ) $ of the system, and under which the given triples of boundary points correspond to each other.
For Lavrent'ev's theorem in mechanics (aerofoil theory, solitary wave, forms of dynamical loss of stability, flows, the theory of cumulative charge, directed detonation) see [4].
Theorems 1)–4) are due to M.A. Lavrent'ev.
References
| [1] | M. [M.A. Lavrent'ev] Lavrentieff, "Contribution à la théorie des ensembles homéomorphes" Fund. Math. , 6 (1924) pp. 149–160 |
| [2] | M.A. Lavrent'ev, Izv. Akad. Nauk SSSR Ser. Mat. , 12 : 6 (1948) pp. 513–554 |
| [3] | M.A. Lavrent'ev, "On the theory of conformal mapping" Tr. Fiz.-Mat. Inst. Akad. Nauk SSSR, Otdel. Mat. , 5 (1934) pp. 195–245 (In Russian) |
| [4] | "Mikhail Alekseevich Lavrent'ev" , Bibliography of Soviet Scientists, Mathematics Series , 12 , Acad. Sci. USSR , Moscow (1971) (In Russian) |
Comments
1) This theorem is valid in the following more general situation: If $ X $ and $ Y $ are completely-metrizable spaces, $ A \subseteq X, B \subseteq Y $, and $ f : A \rightarrow B $ is a homeomorphism, then there are $ G _ \delta $ sets (cf. Set of type $ F _ \sigma $( $ G _ \delta $)) $ A ^ {*} \subseteq X $ and $ B ^ {*} \subseteq Y $ with $ A \subseteq A ^ {*} $, $ B \subseteq B ^ {*} $ and a homeomorphism $ f ^ { * } : A ^ {*} \rightarrow B ^ {*} $ extending $ f $.
This theorem has proved to be very useful in extension theory and in the construction of counterexamples.
2) This theorem is subsumed by the Mergelyan theorem. See [a6]–[a8], [a11].
5) Another problem related with the name of Lavrent'ev is as follows.
Consider the following two optimization problems:
$$ \tag{a1 } \left . \begin{array}{c} \inf \int\limits _ { 0 } ^ { T } L ( t , x , \dot{x} ) dt , \\ x ( 0) = \xi _ {0} ,\ x ( T) = \xi _ {1} ,\ \ x \in L _ {1} ; \\ \end{array} \right \} $$
$$ \tag{a2 } \left . \begin{array}{c} \inf \int\limits _ { 0 } ^ { T } L ( t , x , \dot{x} ) d t , \\ x ( 0) = \xi _ {0} ,\ x ( T) = \ \xi _ {1} ,\ x \in C ^ {1} . \\ \end{array} \right \} $$
I.e. $ x $ is supposed to be absolutely continuous in (a1) and continuously differentiable in (a2), Then it may happen that $ \inf ( A _ {1} ) < \inf ( A _ {2} ) $. This is known as the Lavrent'ev phenomenon. As a consequence, the minimizer for (a1) will not satisfy the Euler–Lagrange equation even though $ L $ is smooth.
References
| [a1] | J. Aarts, "Completeness degree, a generalization of dimension" Fund. Math. , 63 (1968) pp. 28–41 |
| [a2] | T.A. Chapman, "Dense 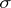 -compact subsets of infinite-dimensional manifolds" Trans. Amer. Math. Soc. , 154 (1971) pp. 399–426 -compact subsets of infinite-dimensional manifolds" Trans. Amer. Math. Soc. , 154 (1971) pp. 399–426 |
| [a3] | E.K. van Douwen, "A compact space with a measure that knows which sets are homeomorphic" Adv. Math. , 52 (1984) pp. 1–33 |
| [a4] | R. Engelking, "General topology" , Heldermann (1989) |
| [a5] | J. van Mill, "Domain invariance in infinite-dimensional linear spaces" Proc. Amer. Math. Soc. , 101 (1987) pp. 173–180 |
| [a6] | S.N. Mergelyan, "On a theorem of M.A. Lavrent'ev" Transl. Amer. Math. Soc. , 3 (1962) pp. 281–286 Dokl. Akad. Nauk SSSR , 77 (1951) pp. 565–568 |
| [a7] | S.N. Mergelyan, "On the representation of functions by series of polynomials on closed sets" Transl. Amer. Math. Soc. , 3 (1962) pp. 287–293 Dokl. Akad. Nauk SSSR , 78 (1951) pp. 405–408 |
| [a8] | E.F. Collingwood, A.J. Lohwater, "The theory of cluster sets" , Cambridge Univ. Press (1966) |
| [a9] | L. Cesari, "Optimization - Theory and applications" , Springer (1983) pp. Sect. 18.5 |
| [a10] | M. [M.A. Lavrent'ev] Lavrentiev, "Sur quelques problèmes du calcul des variations" Ann. Mat. Pura Appl. , 4 (1926) pp. 7–28 |
| [a11] | P.J. Davis, "Interpolation and approximation" , Dover, reprint (1975) pp. 278ff |
| [a12] | M.A. Lavrent'ev, "Variational methods for boundary value problems for systems of elliptic equations" , Noordhoff (1963) (Translated from Russian) |
Lavrent'ev theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lavrent%27ev_theorem&oldid=47593