Difference between revisions of "Dickman-function(2)"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
||
| Line 1: | Line 1: | ||
| + | <!-- | ||
| + | d1101801.png | ||
| + | $#A+1 = 16 n = 2 | ||
| + | $#C+1 = 16 : ~/encyclopedia/old_files/data/D110/D.1100180 Dickman function | ||
| + | Automatically converted into TeX, above some diagnostics. | ||
| + | Please remove this comment and the {{TEX|auto}} line below, | ||
| + | if TeX found to be correct. | ||
| + | --> | ||
| + | |||
| + | {{TEX|auto}} | ||
| + | {{TEX|done}} | ||
| + | |||
The unique continuous solution of the system | The unique continuous solution of the system | ||
| − | + | $$ | |
| + | \rho ( u ) = 1 ( 0 \leq u \leq 1 ) , | ||
| + | $$ | ||
| − | + | $$ | |
| + | u \rho ^ \prime ( u ) = - \rho ( u - 1 ) ( u > 1 ) . | ||
| + | $$ | ||
| − | The Dickman function | + | The Dickman function $ \rho ( u ) $ |
| + | occurs in the problem of estimating the number $ \Psi ( x,y ) $ | ||
| + | of positive integers not exceeding $ x $ | ||
| + | that are free of prime factors greater than $ y $: | ||
| + | for any fixed $ u > 0 $, | ||
| + | one has $ \Psi ( x,x ^ { {1 / u } } ) \sim \rho ( u ) x $ | ||
| + | as $ u \rightarrow \infty $[[#References|[a2]]], [[#References|[a4]]]. | ||
| − | The function | + | The function $ \rho ( u ) $ |
| + | is positive, non-increasing and tends to zero at a rate faster than exponential as $ u \rightarrow \infty $. | ||
| + | A precise asymptotic estimate is given by the de Bruijn–Alladi formula [[#References|[a1]]], [[#References|[a3]]]: | ||
| − | + | $$ | |
| + | \rho ( u ) = ( 1 + O ( { | ||
| + | \frac{1}{u} | ||
| + | } ) ) \sqrt { { | ||
| + | \frac{\xi ^ \prime ( u ) }{2 \pi } | ||
| + | } } \times | ||
| + | $$ | ||
| − | + | $$ | |
| + | \times | ||
| + | { \mathop{\rm exp} } \left \{ \gamma - u \xi ( u ) + \int\limits _ { 0 } ^ { \xi ( u ) } { { | ||
| + | \frac{e ^ {s} - 1 }{s} | ||
| + | } } {ds } \right \} ( u > 1 ) , | ||
| + | $$ | ||
| − | where | + | where $ \gamma $ |
| + | is the [[Euler constant|Euler constant]] and $ \xi ( u ) $ | ||
| + | is the unique positive solution of the equation $ e ^ {\xi ( u ) } = 1 + u \xi ( u ) $. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> K. Alladi, "The Turán–Kubilius inequality for integers without large prime factors" ''J. Reine Angew. Math.'' , '''335''' (1982) pp. 180–196</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> N.G. de Bruijn, "On the number of positive integers <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d110/d110180/d11018017.png" /> and free of prime factors <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d110/d110180/d11018018.png" />" ''Nederl. Akad. Wetensch. Proc. Ser. A'' , '''54''' (1951) pp. 50–60</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> N.G. de Bruijn, "The asymptotic behaviour of a function occurring in the theory of primes" ''J. Indian Math. Soc. (N.S.)'' , '''15''' (1951) pp. 25–32</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> A. Hildebrand, G. Tenenbaum, "Integers without large prime factors" ''J. de Théorie des Nombres de Bordeaux'' , '''5''' (1993) pp. 411–484</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> K. Alladi, "The Turán–Kubilius inequality for integers without large prime factors" ''J. Reine Angew. Math.'' , '''335''' (1982) pp. 180–196</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> N.G. de Bruijn, "On the number of positive integers <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d110/d110180/d11018017.png" /> and free of prime factors <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/d/d110/d110180/d11018018.png" />" ''Nederl. Akad. Wetensch. Proc. Ser. A'' , '''54''' (1951) pp. 50–60</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> N.G. de Bruijn, "The asymptotic behaviour of a function occurring in the theory of primes" ''J. Indian Math. Soc. (N.S.)'' , '''15''' (1951) pp. 25–32</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> A. Hildebrand, G. Tenenbaum, "Integers without large prime factors" ''J. de Théorie des Nombres de Bordeaux'' , '''5''' (1993) pp. 411–484</TD></TR></table> | ||
Revision as of 17:33, 5 June 2020
The unique continuous solution of the system
$$ \rho ( u ) = 1 ( 0 \leq u \leq 1 ) , $$
$$ u \rho ^ \prime ( u ) = - \rho ( u - 1 ) ( u > 1 ) . $$
The Dickman function $ \rho ( u ) $ occurs in the problem of estimating the number $ \Psi ( x,y ) $ of positive integers not exceeding $ x $ that are free of prime factors greater than $ y $: for any fixed $ u > 0 $, one has $ \Psi ( x,x ^ { {1 / u } } ) \sim \rho ( u ) x $ as $ u \rightarrow \infty $[a2], [a4].
The function $ \rho ( u ) $ is positive, non-increasing and tends to zero at a rate faster than exponential as $ u \rightarrow \infty $. A precise asymptotic estimate is given by the de Bruijn–Alladi formula [a1], [a3]:
$$ \rho ( u ) = ( 1 + O ( { \frac{1}{u} } ) ) \sqrt { { \frac{\xi ^ \prime ( u ) }{2 \pi } } } \times $$
$$ \times { \mathop{\rm exp} } \left \{ \gamma - u \xi ( u ) + \int\limits _ { 0 } ^ { \xi ( u ) } { { \frac{e ^ {s} - 1 }{s} } } {ds } \right \} ( u > 1 ) , $$
where $ \gamma $ is the Euler constant and $ \xi ( u ) $ is the unique positive solution of the equation $ e ^ {\xi ( u ) } = 1 + u \xi ( u ) $.
References
| [a1] | K. Alladi, "The Turán–Kubilius inequality for integers without large prime factors" J. Reine Angew. Math. , 335 (1982) pp. 180–196 |
| [a2] | N.G. de Bruijn, "On the number of positive integers 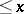 and free of prime factors and free of prime factors 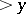 " Nederl. Akad. Wetensch. Proc. Ser. A , 54 (1951) pp. 50–60 " Nederl. Akad. Wetensch. Proc. Ser. A , 54 (1951) pp. 50–60 |
| [a3] | N.G. de Bruijn, "The asymptotic behaviour of a function occurring in the theory of primes" J. Indian Math. Soc. (N.S.) , 15 (1951) pp. 25–32 |
| [a4] | A. Hildebrand, G. Tenenbaum, "Integers without large prime factors" J. de Théorie des Nombres de Bordeaux , 5 (1993) pp. 411–484 |
Dickman-function(2). Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Dickman-function(2)&oldid=46649