Difference between revisions of "Quasi-symmetric function"
(Importing text file) |
(Tex partly done) |
||
| Line 1: | Line 1: | ||
''quasi-symmetric polynomial (in combinatorics)'' | ''quasi-symmetric polynomial (in combinatorics)'' | ||
| − | Let | + | Let $X$ be a finite or infinite set (of variables) and consider the ring of polynomials $R[X]$ and the ring of power series $R[[X]]$ over a [[commutative ring]] $R$ with unit element in the commuting variables from $X$. A polynomial or power series $f(X) \in R[[X]]$ is called ''symmetric'' if for any two finite sequences of indeterminates $X_1,\ldots,X_n$ and $Y_1,\ldots,Y_n$ from $X$ and any sequence of exponents $i_1,\ldots,i_n \in \mathbf{N}$, the coefficients in $f$ of $X_1^{i_1} \cdots X_n^{i_n}$ and $Y_1^{i_1} \cdots Y_n^{i_n}$ are the same. |
| − | |||
| − | |||
| − | |||
| − | |||
| + | Quasi-symmetric formal power series are a generalization introduced by I.M. Gessel, [[#References|[a2]]], in connection with the combinatorics of plane partitions and descent sets of permutations [[#References|[a3]]]. This time one takes a totally ordered set of indeterminates, e.g. $V = \{V_1,V_2,\ldots\}$, with the ordering that of the natural numbers, and the condition is that the coefficients of $X_1^{i_1} \cdots X_n^{i_n}$ and $Y_1^{i_1} \cdots Y_n^{i_n}$ are equal for all totally ordered sets of indeterminates $X_1 < \ldots < X_n$ and $Y_1 < \ldots < Y_n$. For example, | ||
| + | $$ | ||
| + | X_1 X_2^2 + X_1 X_3^2 + X_2 X_3^3$ | ||
| + | $$ | ||
is a quasi-symmetric polynomial in three variables that is not symmetric. | is a quasi-symmetric polynomial in three variables that is not symmetric. | ||
| Line 37: | Line 37: | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> I.M. Gel'fand, D. Krob, A. Lascoux, B. Leclerc, V.S. Retakh, J.-Y. Thibon, "Noncommutative symmetric functions" ''Adv. Math.'' , '''112''' (1995) pp. 218–348</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.M. Gessel, "Multipartite <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/q/q120/q120060/q12006030.png" />-partitions and inner product of skew Schur functions" ''Contemp. Math.'' , '''34''' (1984) pp. 289–301</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> I.M. Gessel, Ch. Reutenauer, "Counting permutations with given cycle-structure and descent set" ''J. Combin. Th. A'' , '''64''' (1993) pp. 189–215</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> C. Malvenuto, Ch. Reutenauer, "Duality between quasi-symmetric functions and the Solomon descent algebra" ''J. Algebra'' , '''177''' (1994) pp. 967–982</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> L. Solomon, "A Mackey formula in the group ring of a Coxeter group" ''J. Algebra'' , '''41''' (1976) pp. 255–268</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M. Hazewinkel, "The algebra of quasi-symmetric functions is free over the integers" ''Preprint CWI (Amsterdam) and ICTP (Trieste)'' (1999)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> M. Chuaqui, B. Osgood, "Weak Schwarzians, bounded hyperbolic distortion, and smooth quasi-symmetric functions" ''J. d'Anal. Math.'' , '''68''' (1996) pp. 209–252</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> I.M. Gel'fand, D. Krob, A. Lascoux, B. Leclerc, V.S. Retakh, J.-Y. Thibon, "Noncommutative symmetric functions" ''Adv. Math.'' , '''112''' (1995) pp. 218–348</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.M. Gessel, "Multipartite <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/q/q120/q120060/q12006030.png" />-partitions and inner product of skew Schur functions" ''Contemp. Math.'' , '''34''' (1984) pp. 289–301</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> I.M. Gessel, Ch. Reutenauer, "Counting permutations with given cycle-structure and descent set" ''J. Combin. Th. A'' , '''64''' (1993) pp. 189–215</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> C. Malvenuto, Ch. Reutenauer, "Duality between quasi-symmetric functions and the Solomon descent algebra" ''J. Algebra'' , '''177''' (1994) pp. 967–982</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> L. Solomon, "A Mackey formula in the group ring of a Coxeter group" ''J. Algebra'' , '''41''' (1976) pp. 255–268</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M. Hazewinkel, "The algebra of quasi-symmetric functions is free over the integers" ''Preprint CWI (Amsterdam) and ICTP (Trieste)'' (1999)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> M. Chuaqui, B. Osgood, "Weak Schwarzians, bounded hyperbolic distortion, and smooth quasi-symmetric functions" ''J. d'Anal. Math.'' , '''68''' (1996) pp. 209–252</TD></TR></table> | ||
| + | |||
| + | {{TEX|part}} | ||
Revision as of 16:31, 28 March 2018
quasi-symmetric polynomial (in combinatorics)
Let $X$ be a finite or infinite set (of variables) and consider the ring of polynomials $R[X]$ and the ring of power series $R[[X]]$ over a commutative ring $R$ with unit element in the commuting variables from $X$. A polynomial or power series $f(X) \in R[[X]]$ is called symmetric if for any two finite sequences of indeterminates $X_1,\ldots,X_n$ and $Y_1,\ldots,Y_n$ from $X$ and any sequence of exponents $i_1,\ldots,i_n \in \mathbf{N}$, the coefficients in $f$ of $X_1^{i_1} \cdots X_n^{i_n}$ and $Y_1^{i_1} \cdots Y_n^{i_n}$ are the same.
Quasi-symmetric formal power series are a generalization introduced by I.M. Gessel, [a2], in connection with the combinatorics of plane partitions and descent sets of permutations [a3]. This time one takes a totally ordered set of indeterminates, e.g. $V = \{V_1,V_2,\ldots\}$, with the ordering that of the natural numbers, and the condition is that the coefficients of $X_1^{i_1} \cdots X_n^{i_n}$ and $Y_1^{i_1} \cdots Y_n^{i_n}$ are equal for all totally ordered sets of indeterminates $X_1 < \ldots < X_n$ and $Y_1 < \ldots < Y_n$. For example, $$ X_1 X_2^2 + X_1 X_3^2 + X_2 X_3^3$ $$ is a quasi-symmetric polynomial in three variables that is not symmetric.
Products and sums of quasi-symmetric polynomials and power series are again quasi-symmetric (obviously), and thus one has, for example, the ring of quasi-symmetric power series
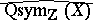 |
in countably many commuting variables over the integers and its subring
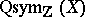 |
of quasi-symmetric polynomials in finite of countably many indeterminates, which are the quasi-symmetric power series of bounded degree.
Given a word 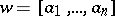 over
over 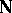 , also called a composition in this context, consider the quasi-monomial function
, also called a composition in this context, consider the quasi-monomial function
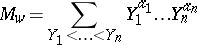 |
defined by 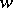 . These form a basis over the integers of
. These form a basis over the integers of 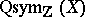 .
.
The algebra of quasi-symmetric functions is dual to the Leibniz–Hopf algebra, or, equivalently to the Solomon descent algebra, more precisely, to the direct sum
 |
of the Solomon descent algebras 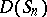 of the symmetric groups (cf. also Symmetric group), [a5], with a new multiplication over which the direct sum of the original multiplications is distributive. See [a1], [a4].
of the symmetric groups (cf. also Symmetric group), [a5], with a new multiplication over which the direct sum of the original multiplications is distributive. See [a1], [a4].
The algebra of quasi-symmetric functions in countably many indeterminates over the integers, 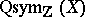 , is a free polynomial algebra over the integers, [a6].
, is a free polynomial algebra over the integers, [a6].
There is a completely different notion in the theory of functions of a complex variable that also goes by the name quasi-symmetric function; cf., e.g., [a7].
References
| [a1] | I.M. Gel'fand, D. Krob, A. Lascoux, B. Leclerc, V.S. Retakh, J.-Y. Thibon, "Noncommutative symmetric functions" Adv. Math. , 112 (1995) pp. 218–348 |
| [a2] | I.M. Gessel, "Multipartite 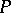 -partitions and inner product of skew Schur functions" Contemp. Math. , 34 (1984) pp. 289–301 -partitions and inner product of skew Schur functions" Contemp. Math. , 34 (1984) pp. 289–301 |
| [a3] | I.M. Gessel, Ch. Reutenauer, "Counting permutations with given cycle-structure and descent set" J. Combin. Th. A , 64 (1993) pp. 189–215 |
| [a4] | C. Malvenuto, Ch. Reutenauer, "Duality between quasi-symmetric functions and the Solomon descent algebra" J. Algebra , 177 (1994) pp. 967–982 |
| [a5] | L. Solomon, "A Mackey formula in the group ring of a Coxeter group" J. Algebra , 41 (1976) pp. 255–268 |
| [a6] | M. Hazewinkel, "The algebra of quasi-symmetric functions is free over the integers" Preprint CWI (Amsterdam) and ICTP (Trieste) (1999) |
| [a7] | M. Chuaqui, B. Osgood, "Weak Schwarzians, bounded hyperbolic distortion, and smooth quasi-symmetric functions" J. d'Anal. Math. , 68 (1996) pp. 209–252 |
Quasi-symmetric function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Quasi-symmetric_function&oldid=43034