Difference between revisions of "De la Vallée-Poussin theorem"
Ulf Rehmann (talk | contribs) m (moved De la Vallee-Poussin theorem to De la Vallée-Poussin theorem over redirect: accented title) |
(TeX partly done) |
||
| Line 1: | Line 1: | ||
| − | The de la Vallée-Poussin theorem on the distribution of prime numbers: Let | + | The de la Vallée-Poussin theorem on the distribution of prime numbers: Let $\pi(x)$ be the number of primes smaller than $x$; then, if $x \ge 1$, the following equality is valid: |
| + | $$ | ||
| + | \pi(x) = \mathrm{li}(x) + O\left({ x \exp(-C\sqrt{\log x}) }\right) | ||
| + | $$ | ||
| + | where $C$ is a positive constant and $\mathrm{li}(x)$ is the [[logarithmic integral]] of $x$. This theorem demonstrates the correctness of Gauss' hypothesis on the distribution of prime numbers, viz., as $x \rightarrow \infty$, | ||
| + | $$ | ||
| + | \pi(x) \sim \frac{x}{\log x} \ . | ||
| + | $$ | ||
| − | + | Established by Ch.J. de la Vallée-Poussin [[#References|[1]]]. Cf. [[Distribution of prime numbers]]. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | Established by Ch.J. de la Vallée-Poussin [[#References|[1]]]. Cf. [[ | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> Ch.J. de la Vallée-Poussin, "Recherches analytiques sur la théorie des nombers premiers" ''Ann. Soc. Sci. Bruxelles'' , '''20''' (1899) pp. 183–256</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> Ch.J. de la Vallée-Poussin, "Sur la fonction | + | <table> |
| + | <TR><TD valign="top">[1]</TD> <TD valign="top"> Ch.J. de la Vallée-Poussin, "Recherches analytiques sur la théorie des nombers premiers" ''Ann. Soc. Sci. Bruxelles'' , '''20''' (1899) pp. 183–256</TD></TR> | ||
| + | <TR><TD valign="top">[2]</TD> <TD valign="top"> Ch.J. de la Vallée-Poussin, "Sur la fonction $\zeta(s)$ de Riemann et la nombre des nombres premiers inférieurs à une limite donnée" ''Mem. Couronnes Acad. Sci. Belg.'' , '''59''' : 1 (1899–1900)</TD></TR> | ||
| + | <TR><TD valign="top">[3]</TD> <TD valign="top"> K. Prachar, "Primzahlverteilung" , Springer (1957)</TD></TR> | ||
| + | </table> | ||
''S.M. Vorazhin'' | ''S.M. Vorazhin'' | ||
| Line 40: | Line 45: | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Favard, "Hommage à Charles de la Vallée Poussin (1866–1962)" P.L. Butzer (ed.) J. Korevaar (ed.) , ''On approximation theory'' , Birkhäuser (1964) pp. 1–3</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> J. Favard, "Hommage à Charles de la Vallée Poussin (1866–1962)" P.L. Butzer (ed.) J. Korevaar (ed.) , ''On approximation theory'' , Birkhäuser (1964) pp. 1–3</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff</TD></TR></table> | ||
| + | |||
| + | {{TEX|part}} | ||
Revision as of 17:02, 12 October 2017
The de la Vallée-Poussin theorem on the distribution of prime numbers: Let $\pi(x)$ be the number of primes smaller than $x$; then, if $x \ge 1$, the following equality is valid: $$ \pi(x) = \mathrm{li}(x) + O\left({ x \exp(-C\sqrt{\log x}) }\right) $$ where $C$ is a positive constant and $\mathrm{li}(x)$ is the logarithmic integral of $x$. This theorem demonstrates the correctness of Gauss' hypothesis on the distribution of prime numbers, viz., as $x \rightarrow \infty$, $$ \pi(x) \sim \frac{x}{\log x} \ . $$
Established by Ch.J. de la Vallée-Poussin [1]. Cf. Distribution of prime numbers.
References
| [1] | Ch.J. de la Vallée-Poussin, "Recherches analytiques sur la théorie des nombers premiers" Ann. Soc. Sci. Bruxelles , 20 (1899) pp. 183–256 |
| [2] | Ch.J. de la Vallée-Poussin, "Sur la fonction $\zeta(s)$ de Riemann et la nombre des nombres premiers inférieurs à une limite donnée" Mem. Couronnes Acad. Sci. Belg. , 59 : 1 (1899–1900) |
| [3] | K. Prachar, "Primzahlverteilung" , Springer (1957) |
S.M. Vorazhin
The de la Vallée-Poussin alternation theorem: If a sequence of points 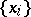 ,
,  , in a closed set
, in a closed set 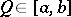 forms an alternation, then for the best approximation of a function
forms an alternation, then for the best approximation of a function 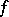 by polynomials of the form
by polynomials of the form
 |
the estimate
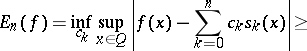 |
 |
is valid, where  is a Chebyshev system. Established by Ch.J. de la Vallée-Poussin [1].
is a Chebyshev system. Established by Ch.J. de la Vallée-Poussin [1].
According to the Chebyshev theorem, equality holds if and only if 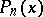 is the polynomial of best approximation. Analogues of this theorem exist for arbitrary Banach spaces [2]. The theorem is employed in numerical methods for constructing polynomials of best approximation.
is the polynomial of best approximation. Analogues of this theorem exist for arbitrary Banach spaces [2]. The theorem is employed in numerical methods for constructing polynomials of best approximation.
References
| [1] | Ch.J. de la Vallée-Poussin, "Sur les polynômes d'approximation et la répresentation approchée d'un angle" Bull. Acad. Belg. , 12 (1910) pp. 808–845 |
| [2] | A.L. Garkavi, "The theory of approximation in normed linear spaces" Itogi Nauk. Mat. Anal. 1967 (1969) pp. 75–132 (In Russian) |
Yu.N. Subbotin
Comments
An account of the life and work of de la Vallée-Poussin can be found in, e.g., [a1].
A sequence of points 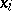 ,
, 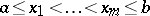 , is called an alternation for a continuous function
, is called an alternation for a continuous function 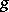 on
on 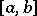 if
if 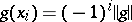 where
where 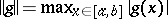 .
.
References
| [a1] | J. Favard, "Hommage à Charles de la Vallée Poussin (1866–1962)" P.L. Butzer (ed.) J. Korevaar (ed.) , On approximation theory , Birkhäuser (1964) pp. 1–3 |
| [a2] | E.W. Cheney, "Introduction to approximation theory" , Chelsea, reprint (1982) pp. 203ff |
De la Vallée-Poussin theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=De_la_Vall%C3%A9e-Poussin_theorem&oldid=42049