Yosida representation theorem

Let 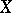be a topological space, the set of continuous real-valued functions on (cf. Continuous functions, space of). Using the pointwise defined partial order: 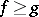if and only if for all 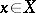, 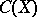becomes a Riesz space. The question arises whether it is possible to represent an arbitrary Riesz space by continuous functions with this order relation where, possibly, more general (extended) functions that can also take the values 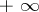and 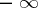may be used. Answers are given by various representation theorems. Below the Yosida representation theorem for the case of Archimedean Riesz spaces with a strong unit is described. For the Yosida representation theorem for Riesz spaces 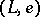, where is a weak unit, see [a1], and for the more general Johnson–Kist representation theorem, see [a2].

A strong unit in a Riesz space 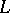is an element 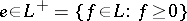such that for all 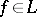there is an 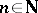such that 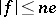, i.e. the principal ideal generated by should equal the whole space. A weak unit in 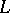is an element of 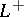such that the principal band generated by is all of .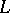

The Riesz space .

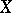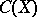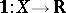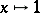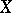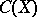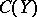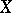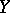Let be a compact Hausdorff space. Then is an Archimedean Riesz space and the function , for all , is a strong unit. Let be a second compact Hausdorff space. The Banach–Stone theorem says that if and are isomorphic as Riesz spaces, then and are homeomorphic. 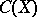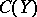As immediate corollaries one obtains that if and are isomorphic as algebras (pointwise multiplication), then and are homeomorphic; also, if and are isomorphic as Banach spaces (sup-norm), then and are homeomorphic.

A topological space 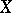is extremely disconnected if every open subset 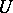has an open closure (i.e. 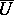is both open and closed). Nakano's theorem says that 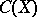is Dedekind complete if and only if 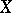is extremely disconnected. It was also obtained independently by T. Ogasawara and M.H. Stone, cf. [a2].

Representation of Archimedean Riesz spaces with strong unit.

Let be an Archimedean Riesz space. Let 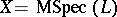be the set of maximal ideals of 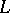. For each 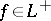, let 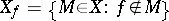. Define a topology on by taking the 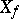as a subbase (cf. Pre-base). The closed sets of 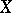are the sets 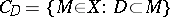, where runs through all subsets of 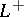. This topology is called the hull-kernel topology on . The construction is a fairly familiar one and occurs in several parts of mathematics. It is originally due to M.H. Stone. Depending on which sets of ideals are used, the mathematical specialism involved, and the ideosyncracies of authors it is also called the Zariski topology, Gel'fand topology, Gel'fand–Kolmogorov topology, Jacobson topology, Grothendieck topology, etc.

From now on, let have a strong unit . For each 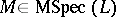, 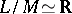and there is a unique homomorphism of Riesz spaces such that 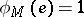. Using this, one defines for every 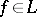a function 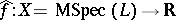by . The number 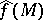can also be described as the unique real number such that 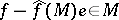. One now has the following representation theorem (K. Yosida, S. Kakutani, M.G. Krein, S.G. Krein, H. Nakano). Let , , be as just described. 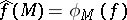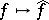Then defines a continuous function on and the mapping is a Riesz isomorphism of 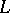onto a Riesz subspace 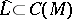. There are a number of complementary facts. 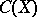Using the Stone–Weierstrass theorem one obtains that is norm dense in ; it is then also order dense.

Given 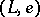, where is a weak unit, 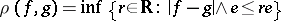defines a metric on , called the uniform metric. If is complete with respect to this metric, 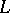is called uniformly closed. A further addition to the representation theorem is then that 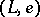is isomorphic to 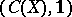if and only if is a strong unit and 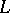is uniformly closed. This last statement, together with that fact that 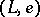is isomorphic to a sub-Riesz space o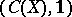f (if is a strong unit), is also referred to as the Krein–Kakutani theorem.

A final complement to the Yosida representation theorem is that if 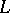has the principal projection property, i.e. 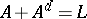for every principal band 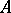, then 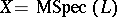is zero dimensional and contains all locally constant functions on 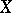. This can also be called the Freudenthal spectral theorem, [a3], in the sense that that theorem in its traditional formulation is an immediate consequence of this result, cf. (the editorial comments to) Riesz space.

References

[a1]	A.W. Hager, L.C. Robertson, "Representing and ringifying a Riesz space" , Symp. Math. INDAM , 21 , Acad. Press (1977) pp. 411–432
[a2]	W.A.J. Luxemburg, A.C. Zaanen, "Riesz spaces" , I , North-Holland (1971) pp. Chapt. 7
[a3]	E. de Jonge, A.C.M. van Rooy, "Introduction to Riesz spaces" , Tracts , 8 , Math. Centre (1977)