Difference between revisions of "De Moivre formula"
From Encyclopedia of Mathematics
(Importing text file) |
m |
||
| Line 1: | Line 1: | ||
| + | {{TEX|want}} | ||
| + | |||
The formula expressing the rule for raising a [[Complex number|complex number]], expressed in trigonometric form | The formula expressing the rule for raising a [[Complex number|complex number]], expressed in trigonometric form | ||
Revision as of 03:57, 5 April 2013
The formula expressing the rule for raising a complex number, expressed in trigonometric form
 |
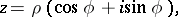to an 
-th power. According to de Moivre's formula the modulus 
of the complex number is raised to that power and the argument 
is multiplied by the exponent:
 |
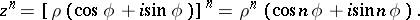The formula was found by A. de Moivre (1707), its modern notation was suggested by L. Euler (1748).
De Moivre's formula can be used to express 
and 
in powers of 
and 
:
 |
 |
 |
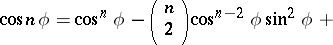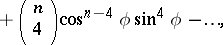Inversion of de Moivre's formula leads to a formula for extracting roots of a complex number:
 |
 |
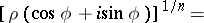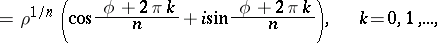which is also sometimes called de Moivre's formula.
Comments
References
| [a1] | A.I. Markushevich, "Theory of functions of a complex variable" , 1 , Chelsea (1977) (Translated from Russian) |
How to Cite This Entry:
De Moivre formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=De_Moivre_formula&oldid=29591
De Moivre formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=De_Moivre_formula&oldid=29591
This article was adapted from an original article by BSE-3 (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article