Difference between revisions of "Lebesgue integral"
(MSC|28A25 Category:Classical measure theory) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 81: | Line 81: | ||
is absolutely continuous with respect to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786076.png" /> (cf. [[Absolute continuity|Absolute continuity]]); if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786077.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786078.png" /> is a non-negative measure that is absolutely continuous with respect to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786079.png" />. The converse assertion is the [[Radon–Nikodým theorem|Radon–Nikodým theorem]]. | is absolutely continuous with respect to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786076.png" /> (cf. [[Absolute continuity|Absolute continuity]]); if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786077.png" />, then <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786078.png" /> is a non-negative measure that is absolutely continuous with respect to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786079.png" />. The converse assertion is the [[Radon–Nikodým theorem|Radon–Nikodým theorem]]. | ||
| − | For functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786080.png" /> the name | + | For functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786080.png" /> the name "Lebesgue integral" is applied to the corresponding functional if the measure <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786081.png" /> is the [[Lebesgue measure|Lebesgue measure]]; here, the set of summable functions is denoted simply by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786082.png" />, and the integral by |
<table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786083.png" /></td> </tr></table> | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/l/l057/l057860/l05786083.png" /></td> </tr></table> | ||
| Line 100: | Line 100: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> H. Lebesgue, "Leçons sur l'intégration et la récherche des fonctions primitives" , Gauthier-Villars (1928) {{MR|2857993}} {{ZBL|54.0257.01}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> N.N. Luzin, "The integral and trigonometric series" , Moscow-Leningrad (1915) (In Russian) (Thesis; also: Collected Works, Vol. 1, Moscow, 1953, pp. 48–212)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , '''1–2''' , Graylock (1957–1961) (Translated from Russian) {{MR|1025126}} {{MR|0708717}} {{MR|0630899}} {{MR|0435771}} {{MR|0377444}} {{MR|0234241}} {{MR|0215962}} {{MR|0118796}} {{MR|1530727}} {{MR|0118795}} {{MR|0085462}} {{MR|0070045}} {{ZBL|0932.46001}} {{ZBL|0672.46001}} {{ZBL|0501.46001}} {{ZBL|0501.46002}} {{ZBL|0235.46001}} {{ZBL|0103.08801}} </TD></TR></table> |
| Line 108: | Line 108: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> P.R. Halmos, "Measure theory" , v. Nostrand (1950) {{MR|0033869}} {{ZBL|0040.16802}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> I.N. Pesin, "Classical and modern integration theories" , Acad. Press (1970) (Translated from Russian) {{MR|0264015}} {{ZBL|0206.06401}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French) {{MR|0167578}} {{ZBL|1196.28001}} {{ZBL|0017.30004}} {{ZBL|63.0183.05}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H.L. Royden, "Real analysis" , Macmillan (1968) {{MR|1013117}} {{MR|1532990}} {{MR|0151555}} {{ZBL|1191.26002}} {{ZBL|0704.26006}} {{ZBL|0197.03501}} {{ZBL|0121.05501}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> W. Rudin, "Real and complex analysis" , McGraw-Hill (1978) pp. 24 {{MR|1736644}} {{MR|1645547}} {{MR|0924157}} {{MR|0850722}} {{MR|0662565}} {{MR|0344043}} {{MR|0210528}} {{ZBL|1038.00002}} {{ZBL|0954.26001}} {{ZBL|0925.00005}} {{ZBL|0613.26001}} {{ZBL|0925.00003}} {{ZBL|0278.26001}} {{ZBL|0142.01701}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965) {{MR|0188387}} {{ZBL|0137.03202}} </TD></TR></table> |
Revision as of 10:31, 27 March 2012
2020 Mathematics Subject Classification: Primary: 28A25 [MSN][ZBL]
The most important generalization of the concept of an integral. Let  be a space with a non-negative complete countably-additive measure
be a space with a non-negative complete countably-additive measure  (cf. Countably-additive set function; Measure space), where
(cf. Countably-additive set function; Measure space), where  . A simple function is a measurable function
. A simple function is a measurable function  that takes at most a countable set of values:
that takes at most a countable set of values:  ,
,  for
for  , if
, if  ,
,  . A simple function
. A simple function  is said to be summable if the series
is said to be summable if the series
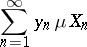 |
converges absolutely (cf. Absolutely convergent series); the sum of this series is the Lebesgue integral
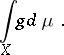 |
A function 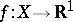 is summable on
is summable on 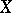 ,
, 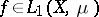 , if there is a sequence of simple summable functions
, if there is a sequence of simple summable functions 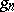 uniformly convergent (cf. Uniform convergence) to
uniformly convergent (cf. Uniform convergence) to 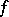 on a set of full measure, and if the limit
on a set of full measure, and if the limit
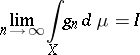 |
is finite. The number 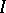 is the Lebesgue integral
is the Lebesgue integral
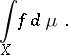 |
This is well-defined: the limit 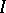 exists and does not depend on the choice of the sequence
exists and does not depend on the choice of the sequence 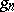 . If
. If 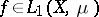 , then
, then  is a measurable almost-everywhere finite function on
is a measurable almost-everywhere finite function on 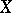 . The Lebesgue integral is a linear non-negative functional on
. The Lebesgue integral is a linear non-negative functional on 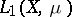 with the following properties:
with the following properties:
1) if  and if
and if
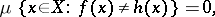 |
then 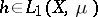 and
and
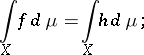 |
2) if  , then
, then 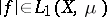 and
and
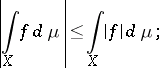 |
3) if 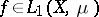 ,
,  and
and 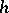 is measurable, then
is measurable, then 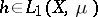 and
and
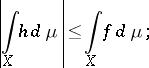 |
4) if  and
and  is measurable, then
is measurable, then 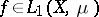 and
and
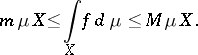 |
In the case when 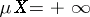 and
and 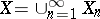 ,
, 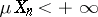 , the Lebesgue integral is defined as
, the Lebesgue integral is defined as
 |
under the condition that this limit exists and is finite for any sequence 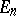 such that
such that 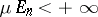 ,
,  ,
,  . In this case the properties 1), 2), 3) are preserved, but condition 4) is violated.
. In this case the properties 1), 2), 3) are preserved, but condition 4) is violated.
For the transition to the limit under the Lebesgue integral sign see Lebesgue theorem.
If 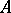 is a measurable set in
is a measurable set in  , then the Lebesgue integral
, then the Lebesgue integral
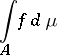 |
is defined either as above, by replacing  by
by  , or as
, or as
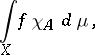 |
where 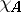 is the characteristic function of
is the characteristic function of 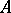 ; these definitions are equivalent. If
; these definitions are equivalent. If 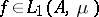 , then
, then 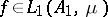 for any measurable
for any measurable 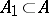 . If
. If
 |
if 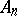 is measurable for every
is measurable for every  , if
, if
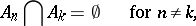 |
and if 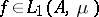 , then
, then
 |
Conversely, if under these conditions on 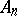 one has
one has 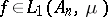 for every
for every  and if
and if
 |
then  and the previous equality is true (
and the previous equality is true ( -additivity of the Lebesgue integral).
-additivity of the Lebesgue integral).
The function of sets  given by
given by
 |
is absolutely continuous with respect to 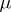 (cf. Absolute continuity); if
(cf. Absolute continuity); if  , then
, then  is a non-negative measure that is absolutely continuous with respect to
is a non-negative measure that is absolutely continuous with respect to 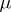 . The converse assertion is the Radon–Nikodým theorem.
. The converse assertion is the Radon–Nikodým theorem.
For functions 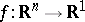 the name "Lebesgue integral" is applied to the corresponding functional if the measure
the name "Lebesgue integral" is applied to the corresponding functional if the measure 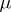 is the Lebesgue measure; here, the set of summable functions is denoted simply by
is the Lebesgue measure; here, the set of summable functions is denoted simply by 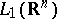 , and the integral by
, and the integral by
 |
For other measures this functional is called a Lebesgue–Stieltjes integral.
If  ,
, 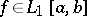 and if
and if 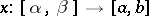 is a non-decreasing absolutely continuous function, then
is a non-decreasing absolutely continuous function, then
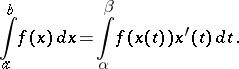 |
If 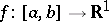 ,
, 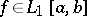 and if
and if  is monotone on
is monotone on 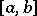 , then
, then 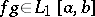 and there is a point
and there is a point  such that
such that
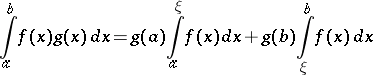 |
(the second mean-value theorem).
In 1902 H. Lebesgue gave (see [1]) a definition of the integral for 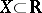 and measure
and measure 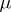 equal to the Lebesgue measure. He constructed simple functions that uniformly approximate almost-everywhere on a set
equal to the Lebesgue measure. He constructed simple functions that uniformly approximate almost-everywhere on a set 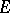 of finite measure a measurable non-negative function
of finite measure a measurable non-negative function 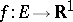 , and proved the existence of a common limit (finite or infinite) of the integrals of these simple functions as they tend to
, and proved the existence of a common limit (finite or infinite) of the integrals of these simple functions as they tend to 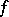 . The Lebesgue integral is a basis for various generalizations of the concept of an integral. As N.N. Luzin remarked [2], property 2), called absolute integrability, distinguishes the Lebesgue integral for
. The Lebesgue integral is a basis for various generalizations of the concept of an integral. As N.N. Luzin remarked [2], property 2), called absolute integrability, distinguishes the Lebesgue integral for 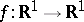 from all possible generalized integrals.
from all possible generalized integrals.
References
| [1] | H. Lebesgue, "Leçons sur l'intégration et la récherche des fonctions primitives" , Gauthier-Villars (1928) MR2857993 Zbl 54.0257.01 |
| [2] | N.N. Luzin, "The integral and trigonometric series" , Moscow-Leningrad (1915) (In Russian) (Thesis; also: Collected Works, Vol. 1, Moscow, 1953, pp. 48–212) |
| [3] | A.N. Kolmogorov, S.V. Fomin, "Elements of the theory of functions and functional analysis" , 1–2 , Graylock (1957–1961) (Translated from Russian) MR1025126 MR0708717 MR0630899 MR0435771 MR0377444 MR0234241 MR0215962 MR0118796 MR1530727 MR0118795 MR0085462 MR0070045 Zbl 0932.46001 Zbl 0672.46001 Zbl 0501.46001 Zbl 0501.46002 Zbl 0235.46001 Zbl 0103.08801 |
Comments
For other generalizations of the notion of an integral see 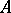 -integral; Bochner integral; Boks integral; Burkill integral; Daniell integral; Darboux sum; Denjoy integral; Kolmogorov integral; Perron integral; Perron–Stieltjes integral; Pettis integral; Radon integral; Stieltjes integral; Strong integral; Wiener integral. See also, of course, Riemann integral. See also Double integral; Improper integral; Fubini theorem (on changing the order of integration).
-integral; Bochner integral; Boks integral; Burkill integral; Daniell integral; Darboux sum; Denjoy integral; Kolmogorov integral; Perron integral; Perron–Stieltjes integral; Pettis integral; Radon integral; Stieltjes integral; Strong integral; Wiener integral. See also, of course, Riemann integral. See also Double integral; Improper integral; Fubini theorem (on changing the order of integration).
References
| [a1] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) MR0033869 Zbl 0040.16802 |
| [a2] | I.N. Pesin, "Classical and modern integration theories" , Acad. Press (1970) (Translated from Russian) MR0264015 Zbl 0206.06401 |
| [a3] | S. Saks, "Theory of the integral" , Hafner (1952) (Translated from French) MR0167578 Zbl 1196.28001 Zbl 0017.30004 Zbl 63.0183.05 |
| [a4] | H.L. Royden, "Real analysis" , Macmillan (1968) MR1013117 MR1532990 MR0151555 Zbl 1191.26002 Zbl 0704.26006 Zbl 0197.03501 Zbl 0121.05501 |
| [a5] | W. Rudin, "Real and complex analysis" , McGraw-Hill (1978) pp. 24 MR1736644 MR1645547 MR0924157 MR0850722 MR0662565 MR0344043 MR0210528 Zbl 1038.00002 Zbl 0954.26001 Zbl 0925.00005 Zbl 0613.26001 Zbl 0925.00003 Zbl 0278.26001 Zbl 0142.01701 |
| [a6] | E. Hewitt, K.R. Stromberg, "Real and abstract analysis" , Springer (1965) MR0188387 Zbl 0137.03202 |
Lebesgue integral. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lebesgue_integral&oldid=23618